We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

TEXT
Unlock Solution Steps
Sign in to
Symbolab
Get full access to all Solution Steps for any math problem
By continuing, you agree to our Terms of Use
and have read our Privacy Policy

Study Guides > Boundless Algebra

Graphs of Quadratic Functions

Parts of a Parabola

The graph of a quadratic function is a parabola, and its parts provide valuable information about the function.

Learning Objectives

Describe the parts and features of parabolas

Key Takeaways

Key Points

  • The graph of a quadratic function is a U-shaped curve called a parabola.
  • The sign on the coefficient [latex]a[/latex] of the quadratic function affects whether the graph opens up or down. If [latex]a<0[/latex], the graph makes a frown (opens down) and if [latex]a>0[/latex] then the graph makes a smile (opens up).
  • The extreme point ( maximum or minimum ) of a parabola is called the vertex, and the axis of symmetry is a vertical line that passes through the vertex.
  • The x-intercepts are the points at which the parabola crosses the x-axis. If they exist, the x-intercepts represent the zeros, or roots, of the quadratic function.

Key Terms

  • vertex: The point at which a parabola changes direction, corresponding to the minimum or maximum value of the quadratic function.
  • axis of symmetry: A vertical line drawn through the vertex of a parabola around which the parabola is symmetric.
  • zeros: In a given function, the values of [latex]x[/latex] at which [latex]y=0[/latex], also called roots.
Recall that a quadratic function has the form [latex]\displaystyle f(x)=ax^{2}+bx+c[/latex]. where [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are constants, and [latex]a\neq 0[/latex]. The graph of a quadratic function is a U-shaped curve called a parabola.  This shape is shown below.
A parabola has a maximum or a minimum, called the vertex, an axis of symmetry through the middle of the parabola, a y-intercept where it crosses the y-axis, and can have as many as two x-intercepts where it crosses the x-axis. Parabola : The graph of a quadratic function is a parabola.
In graphs of quadratic functions, the sign on the coefficient [latex]a[/latex] affects whether the graph opens up or down. If [latex]a<0[/latex], the graph makes a frown (opens down) and if [latex]a>0[/latex] then the graph makes a smile (opens up). This is shown below.
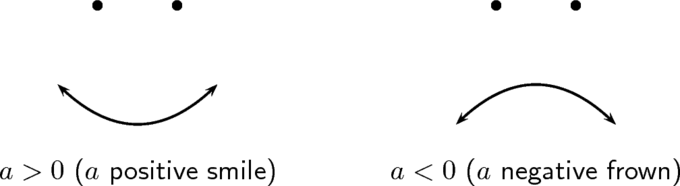image Direction of Parabolas: The sign on the coefficient [latex]a[/latex] determines the direction of the parabola.

Features of Parabolas

Parabolas have several recognizable features that characterize their shape and placement on the Cartesian plane.

Vertex

One important feature of the parabola is that it has an extreme point, called the vertex. If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. In either case, the vertex is a turning point on the graph.

Axis of Symmetry

Parabolas also have an axis of symmetry, which is parallel to the y-axis. The axis of symmetry is a vertical line drawn through the vertex.

[latex]y[/latex]-intercept

The y-intercept is the point at which the parabola crosses the y-axis. There cannot be more than one such point, for the graph of a quadratic function. If there were, the curve would not be a function, as there would be two [latex]y[/latex] values for one [latex]x[/latex] value, at zero.

[latex]x[/latex]-intercepts

The x-intercepts are the points at which the parabola crosses the x-axis. If they exist, the x-intercepts represent the zeros, or roots, of the quadratic function, the values of [latex]x[/latex] at which [latex]y=0[/latex]. There may be zero, one, or two [latex]x[/latex]-intercepts. The number of [latex]x[/latex]-intercepts varies depending upon the location of the graph (see the diagram below).
A parabola with a vertex above the x-axis that opens up (or a vertex below the x-axis that opens down) does not cross the x-axis at all, and has no x-intercepts. A parabola with its vertex on the x-axis has one x-intercept. All other parabolas (vertex below the x-axis opening up, or vertex above the x-axis opening down) have two x-intercepts. Possible [latex]x[/latex]-intercepts: A parabola can have no x-intercepts, one x-intercept, or two x-intercepts

Recall that if the quadratic function is set equal to zero, then the result is a quadratic equation. The solutions to the equation are called the roots of the function. These are the same roots that are observable as the [latex]x[/latex]-intercepts of the parabola.

Notice that, for parabolas with two [latex]x[/latex]-intercepts, the vertex always falls between the roots. Due to the fact that parabolas are symmetric, the [latex]x[/latex]-coordinate of the vertex is exactly in the middle of the [latex]x[/latex]-coordinates of the two roots.

A Graphical Interpretation of Quadratic Solutions

The roots of a quadratic function can be found algebraically or graphically.

Learning Objectives

Describe the solutions to a quadratic equation as the points where the parabola crosses the x-axis

Key Takeaways

Key Points

  • The roots of a quadratic function can be found algebraically with the quadratic formula, and graphically by making observations about its parabola.
  • The solutions, or roots, of a given quadratic equation are the same as the zeros, or [latex]x[/latex]-intercepts, of the graph of the corresponding quadratic function.

Key Terms

  • zeros: In a given function, the values of [latex]x[/latex] at which [latex]y = 0[/latex], also called roots.
Recall how the roots of quadratic functions can be found algebraically, using the quadratic formula [latex](x=\frac{-b \pm \sqrt {b^2-4ac}}{2a})[/latex]. The roots of a quadratic function can also be found graphically by making observations about its graph. These are two different methods that can be used to reach the same values, and we will now see how they are related. Consider the quadratic function that is graphed below. Let's solve for its roots both graphically and algebraically.
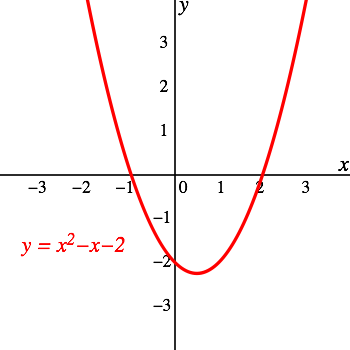The parabola opens up and has a vertex at approximately (.5, -2). Graph of the quadratic function [latex]f(x) = x^2 - x - 2[/latex]: Graph showing the parabola on the Cartesian plane, including the points where it crosses the x-axis.
Notice that the parabola intersects the [latex]x[/latex]-axis at two points: [latex](-1, 0)[/latex] and [latex](2, 0)[/latex]. Recall that the [latex]x[/latex]-intercepts of a parabola indicate the roots, or zeros, of the quadratic function. Therefore, there are roots at [latex]x = -1[/latex] and [latex]x = 2[/latex]. Now, let's solve for the roots of [latex]f(x) = x^2 - x- 2[/latex] algebraically with the quadratic formula. Recall that the quadratic equation sets the quadratic expression equal to zero instead of [latex]f(x)[/latex]: [latex-display]0 = x^2 - x - 2[/latex-display] Now the quadratic formula can be applied to find the [latex]x[/latex]-values for which this statement is true. For the given equation, we have the following coefficients: [latex]a = 1[/latex], [latex]b = -1[/latex], and [latex]c = -2[/latex]. Substitute these values in the quadratic formula: [latex-display]x = \dfrac{-(-1) \pm \sqrt {(-1)^2-4(1)(-2)}}{2(1)}[/latex-display] Simplifying, we have: [latex-display]x = \dfrac{1 \pm \sqrt {9}}{2} \\[/latex-display] and [latex-display]x = \dfrac{1 \pm 3}{2}[/latex-display] We now have two possible values for x: [latex]\frac{1+3}{2}[/latex] and [latex]\frac{1-3}{2}[/latex]. These reduce to [latex]x = 2[/latex] and [latex]x = - 1[/latex], respectively. Notice that these are the same values that when found when we solved for roots graphically.

Example

Find the roots of the quadratic function [latex]f(x) = x^2 - 4x + 4[/latex]. Solve graphically and algebraically.
The parabola opens up and has a y-intercept at (0, 5). The graph of [latex]f(x) = x^2 - 4x + 4[/latex].: The graph of the above function, with the vertex labeled at [latex](2, 1)[/latex].
Looking at the graph of the function, we notice that it does not intersect the [latex]x[/latex]-axis. Therefore, it has no real roots. We can verify this algebraically. First, identify the values for the coefficients: [latex]a = 1[/latex], [latex]b = - 4[/latex], and [latex]c = 5[/latex]. Substituting these into the quadratic formula, we have: [latex-display]x=\dfrac{-(-4) \pm \sqrt {(-4)^2-4(1)(5)}}{2(1)}[/latex-display] Simplifying, we have: [latex]x=\dfrac{4 \pm \sqrt {16-20}}{2} \\ x=\dfrac{4 \pm \sqrt {-4}}{2}[/latex] Notice that we have [latex]\sqrt{-4}[/latex] in the formula, which is not a real number. Therefore, there are no real roots for the given quadratic function. We have arrived at the same conclusion that we reached graphically.

Graphing Quadratic Equations in Vertex Form

The vertex form of a quadratic function lets its vertex be found easily.

Learning Objectives

Explain the meanings of the constants [latex]a[/latex], [latex]h[/latex], and [latex]k[/latex] for a quadratic equation in vertex form

Key Takeaways

Key Points

  • An important form of a quadratic function is vertex form: [latex]f(x) = a(x-h)^2 + k[/latex]
  • When written in vertex form, it is easy to see the vertex of the parabola at [latex](h, k)[/latex].
  • It is easy to convert from vertex form to standard form.
  • It is more difficult, but still possible, to convert from standard form to vertex form. The process involves a technique called completing the square.

Key Terms

  • constant: An identifier that is bound to an invariant value.
  • vertex: A point on the curve with a local minimum or maximum of curvature.
  • quadratic: A polynomial of degree two.
Quadratic equations may take various forms. You have already seen the standard form: [latex-display]f(x)=a{ x }^{ 2 }+bx+c[/latex-display] Another common form is called vertex form, because when a quadratic is written in this form, it is very easy to tell where its vertex is located. The vertex form is given by: [latex-display]f(x)=a(x-h)^2+k[/latex-display] The vertex is [latex](h,k).[/latex] Note that if the form were [latex]f(x)=a(x+h)^2+k[/latex], the vertex would be [latex](-h,k).[/latex] The coefficient [latex]a[/latex] as before controls whether the parabola opens upward or downward, as well as the speed of increase or decrease of the parabola.

Converting From Vertex Form to Standard Form

If you want to convert a quadratic in vertex form to one in standard form, simply multiply out the square and combine like terms. For example, the quadratic [latex-display]y=(x-2)^2+1[/latex-display] Can be rewritten as follows: [latex-display]\begin{align} y&=(x-2)(x-2)+1 \\ &=x^2-2x-2x+4+1 \\ &=x^2-4x+5 \end{align}[/latex-display]

Converting From Standard Form to Vertex Form

It is more difficult to convert from standard form to vertex form. The process is called "completing the square."

Conversion When [latex]a=1[/latex]

Consider the following example: suppose you want to write [latex]y=x^2+4x+6[/latex] in vertex form. Note that the coefficient on [latex]x^2[/latex] (the one we call [latex]a[/latex]) is [latex]1[/latex]. When this is the case, we look at the coefficient on [latex]x[/latex] (the one we call [latex]b[/latex]) and take half of it. Then we square that number. Thus for this example, we divide [latex]4[/latex] by [latex]2[/latex] to obtain [latex]2[/latex] and then square it to obtain [latex]4[/latex]. We then both add and subtract this number as follows: [latex-display]y=(x^2+4x+4)+6-4[/latex-display] Note that we both added and subtracted 4, so we didn't actually change our function. Now the expression in the parentheses is a square; we can write [latex]y=(x+2)^2+2.[/latex] Our equation is now in vertex form and we can see that the vertex is [latex](-2,2).[/latex]

Conversion When [latex]a \neq 1[/latex]

It is slightly more complicated to convert standard form to vertex form when the coefficient [latex]a[/latex] is not equal to [latex]1[/latex]. We can still use the technique, but must be careful to first factor out the [latex]a[/latex] as in the following example: Consider [latex]y=2x^2+12x+5. [/latex] We factor out the coefficient [latex]2[/latex] from the first two terms, writing this as: [latex-display]y=2(x^2+6x) + 5[/latex-display] We then complete the square within the parentheses. Note that half of [latex]6[/latex] is [latex]3[/latex] and [latex]3^2=9[/latex]. So we add and subtract [latex]9[/latex] within the parentheses, obtaining: [latex-display]y=2(x^2+6x+9-9)+5[/latex-display] We can then finish the calculation as follows: [latex-display]\begin{align} y&=2((x+3)^2-9)+5 \\ &=2(x+3)^2-18+5 \\ &=(x+3)^2-13 \end{align}[/latex-display] So the vertex of this parabola is [latex](-3,-13).[/latex]

Graphing Quadratic Equations In Standard Form

A quadratic function is a polynomial function of the form [latex]y=ax^2+bx+c[/latex].

Learning Objectives

Explain the meanings of the constants [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] for a quadratic equation in standard form

Key Takeaways

Key Points

  • The graph of a quadratic function is a parabola whose axis of symmetry is parallel to the [latex]y[/latex]-axis.
  • The coefficients [latex]a, b,[/latex] and [latex]c[/latex] in the equation [latex]y=ax^2+bx+c[/latex] control various facets of what the parabola looks like when graphed.

Key Terms

  • vertex: The maximum or minimum of a quadratic function.
  • parabola: The shape formed by the graph of a quadratic function.
  • quadratic: A polynomial of degree two.
A quadratic function in the form [latex-display]f(x)=a{ x }^{ 2 }+bx+x[/latex-display] is in standard form. Regardless of the format, the graph of a quadratic function is a parabola.
image The graph of [latex]y=x^2-4x+3[/latex] : The graph of any quadratic equation is always a parabola.

Coefficients and Graphs of Quadratic Function

Each coefficient in a quadratic function in standard form has an impact on the shape and placement of the function's graph.

Coefficient of [latex]x^2[/latex], [latex]a[/latex]

The coefficient [latex]a[/latex] controls the speed of increase (or decrease) of the quadratic function from the vertex. A larger, positive [latex]a[/latex] makes the function increase faster and the graph appear thinner.
image The coefficient [latex]a[/latex] controls the speed of increase of the parabola.: The black curve is [latex]y=4x^2[/latex] while the blue curve is [latex]y=3x^2.[/latex] The black curve appears thinner because its coefficient [latex]a[/latex] is bigger than that of the blue curve.
Whether the parabola opens upward or downward is also controlled by [latex]a[/latex]. If the coefficient [latex]a>0[/latex], the parabola opens upward, and if the coefficient [latex]a<0[/latex], the parabola opens downward.
image Quadratics either open upward or downward: The blue parabola is the graph of [latex]y=3x^2.[/latex] It opens upward since [latex]a=3>0.[/latex] The black parabola is the graph of [latex]y=-3x^2.[/latex] It opens downward since [latex]a=-3<0.[/latex].

The Axis of Symmetry

The coefficients [latex]b[/latex] and [latex]a[/latex] together control the axis of symmetry of the parabola and the [latex]x[/latex]-coordinate of the vertex. The axis of symmetry for a parabola is given by: [latex-display]x=-\dfrac{b}{2a}[/latex-display] For example, consider the parabola [latex]y=2x^2-4x+4 [/latex] shown below. Because [latex]a=2[/latex] and [latex]b=-4,[/latex] the axis of symmetry is: [latex-display]x=-\frac{-4}{2\cdot 2} = 1[/latex-display] The vertex also has [latex]x[/latex] coordinate [latex]1[/latex].
image The graph of [latex]y=2x^2-4x+4.[/latex]: The axis of symmetry is a vertical line parallel to the y-axis at  [latex]x=1[/latex].

The [latex]y[/latex]-intercept of the Parabola

The coefficient [latex]c[/latex] controls the height of the parabola. More specifically, it is the point where the parabola intercepts the y-axis. The point [latex](0,c)[/latex] is the [latex]y[/latex] intercept of the parabola. Note that the parabola above has [latex]c=4[/latex] and it intercepts the [latex]y[/latex]-axis at the point [latex](0,4).[/latex]

Licenses & Attributions

CC licensed content, Shared previously

CC licensed content, Specific attribution