Cardinality
Often times we are interested in the number of items in a set or subset. This is called the cardinality of the set.
Cardinality
The number of elements in a set is the cardinality of that set. The cardinality of the set A is often notated as |A| or n(A)Example 12
Let A = {1, 2, 3, 4, 5, 6} and B = {2, 4, 6, 8}. What is the cardinality of B? A ⋃ B, A ⋂ B? The cardinality of B is 4, since there are 4 elements in the set. The cardinality of A ⋃ B is 7, since A ⋃ B = {1, 2, 3, 4, 5, 6, 8}, which contains 7 elements. The cardinality of A ⋂ B is 3, since A ⋂ B = {2, 4, 6}, which contains 3 elements.Example 13
What is the cardinality of P = the set of English names for the months of the year? The cardinality of this set is 12, since there are 12 months in the year. Sometimes we may be interested in the cardinality of the union or intersection of sets, but not know the actual elements of each set. This is common in surveying.Example 14
A survey asks 200 people “What beverage do you drink in the morning”, and offers choices:- Tea only
- Coffee only
- Both coffee and tea

Example 15
A survey asks: Which online services have you used in the last month:- Have used both
Cardinality properties
n(A ⋃ B) = n(A) + n(B) – n(A ⋂ B) n(Ac) = n(U) – n(A)Example 16
Fifty students were surveyed, and asked if they were taking a social science (SS), humanities (HM) or a natural science (NS) course the next quarter. 21 were taking a SS course 26 were taking a HM course 19 were taking a NS course 9 were taking SS and HM 7 were taking SS and NS 10 were taking HM and NS 3 were taking all three 7 were taking none How many students are only taking a SS course? It might help to look at a Venn diagram.
From the given data, we know that there are
3 students in region e and
7 students in region h.
Since 7 students were taking a SS and NS course, we know that n(d) + n(e) = 7. Since we know there are 3 students in region 3, there must be
7 – 3 = 4 students in region d.
Similarly, since there are 10 students taking HM and NS, which includes regions e and f, there must be
10 – 3 = 7 students in region f.
Since 9 students were taking SS and HM, there must be 9 – 3 = 6 students in region b.
Now, we know that 21 students were taking a SS course. This includes students from regions a, b, d, and e. Since we know the number of students in all but region a, we can determine that 21 – 6 – 4 – 3 = 8 students are in region a.
8 students are taking only a SS course.
It might help to look at a Venn diagram.
From the given data, we know that there are
3 students in region e and
7 students in region h.
Since 7 students were taking a SS and NS course, we know that n(d) + n(e) = 7. Since we know there are 3 students in region 3, there must be
7 – 3 = 4 students in region d.
Similarly, since there are 10 students taking HM and NS, which includes regions e and f, there must be
10 – 3 = 7 students in region f.
Since 9 students were taking SS and HM, there must be 9 – 3 = 6 students in region b.
Now, we know that 21 students were taking a SS course. This includes students from regions a, b, d, and e. Since we know the number of students in all but region a, we can determine that 21 – 6 – 4 – 3 = 8 students are in region a.
8 students are taking only a SS course.
Try it Now 4
One hundred fifty people were surveyed and asked if they believed in UFOs, ghosts, and Bigfoot. 43 believed in UFOs 44 believed in ghosts 25 believed in Bigfoot 10 believed in UFOs and ghosts 8 believed in ghosts and Bigfoot 5 believed in UFOs and Bigfoot 2 believed in all three How many people surveyed believed in at least one of these things?Try it Now Answers
1. There are several answers: The set of all odd numbers less than 10. The set of all odd numbers. The set of all integers. The set of all real numbers. 2. A ⋃ C = {red, orange, yellow, green, blue purple} Bc ⋂ A = {green, blue} 3. A ⋃ B ⋂ Cc 4. Starting with the intersection of all three circles, we work our way out. Since 10 people believe in UFOs and Ghosts, and 2 believe in all three, that leaves 8 that believe in only UFOs and Ghosts. We work our way out, filling in all the regions. Once we have, we can add up all those regions, getting 91 people in the union of all three sets. This leaves 150 – 91 = 59 who believe in none.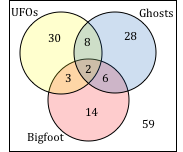
Licenses & Attributions
CC licensed content, Shared previously
- Math in Society. Authored by: Open Textbook Store, Transition Math Project, and the Open Course Library. Located at: http://www.opentextbookstore.com/mathinsociety/. License: CC BY-SA: Attribution-ShareAlike.
