We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > College Algebra

Solutions

Solutions to Try Its

1. [latex]|x - 2|\le 3[/latex]

2. using the variable [latex]p[/latex] for passing, [latex]|p - 80|\le 20[/latex]

3. [latex]f\left(x\right)=-|x+2|+3[/latex]

4. [latex]x=-1[/latex] or [latex]x=2[/latex]

5. [latex]f\left(0\right)=1[/latex], so the graph intersects the vertical axis at [latex]\left(0,1\right)[/latex]. [latex]f\left(x\right)=0[/latex] when [latex]x=-5[/latex] and [latex]x=1[/latex] so the graph intersects the horizontal axis at [latex]\left(-5,0\right)[/latex] and [latex]\left(1,0\right)[/latex].

6. [latex]4\le x\le 8[/latex]

7. [latex]k\le 1[/latex] or [latex]k\ge 7[/latex]; in interval notation, this would be [latex]\left(-\infty ,1\right]\cup \left[7,\infty \right)[/latex]

Solutions to Odd-Numbered Exercises

1. Isolate the absolute value term so that the equation is of the form [latex]|A|=B[/latex]. Form one equation by setting the expression inside the absolute value symbol, [latex]A[/latex], equal to the expression on the other side of the equation, [latex]B[/latex]. Form a second equation by setting [latex]A[/latex] equal to the opposite of the expression on the other side of the equation, -B. Solve each equation for the variable.

3. The graph of the absolute value function does not cross the [latex]x[/latex] -axis, so the graph is either completely above or completely below the [latex]x[/latex] -axis.

5. First determine the boundary points by finding the solution(s) of the equation. Use the boundary points to form possible solution intervals. Choose a test value in each interval to determine which values satisfy the inequality.

7. [latex]|x+4|=\frac{1}{2}[/latex]

9. [latex]|f\left(x\right)-8|<0.03[/latex]

11. [latex]\left\{1,11\right\}[/latex]

13. [latex]\left\{\frac{9}{4},\frac{13}{4}\right\}[/latex]

15. [latex]\left\{\frac{10}{3},\frac{20}{3}\right\}[/latex]

17. [latex]\left\{\frac{11}{5},\frac{29}{5}\right\}[/latex]

19. [latex]\left\{\frac{5}{2},\frac{7}{2}\right\}[/latex]

21. No solution

23. [latex]\left\{-57,27\right\}[/latex]

25. [latex]\left(0,-8\right);\left(-6,0\right),\left(4,0\right)[/latex]

27. [latex]\left(0,-7\right)[/latex]; no [latex]x[/latex] -intercepts

29. [latex]\left(-\infty ,-8\right)\cup \left(12,\infty \right)[/latex]

31. [latex]\frac{-4}{3}\le x\le 4[/latex]

33. [latex]\left(-\infty ,-\frac{8}{3}\right]\cup \left[6,\infty \right)[/latex]

35. [latex]\left(-\infty ,-\frac{8}{3}\right]\cup \left[16,\infty \right)[/latex]

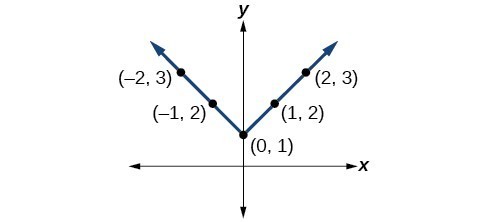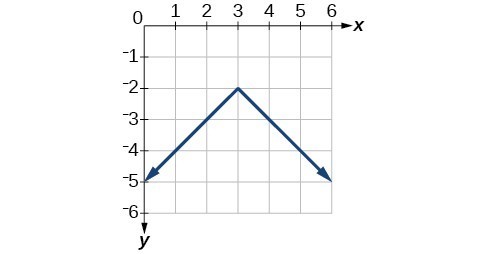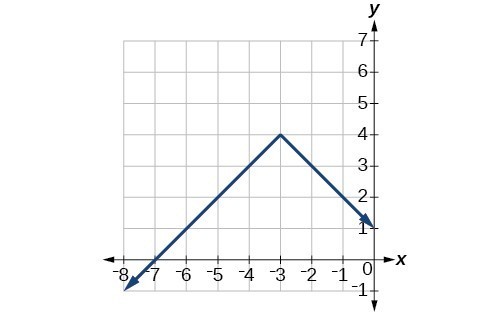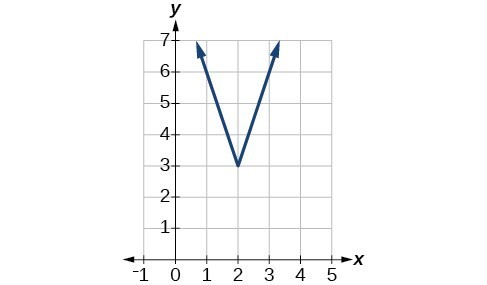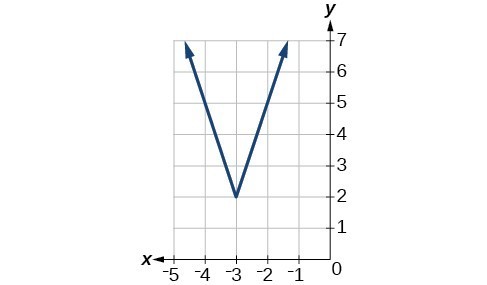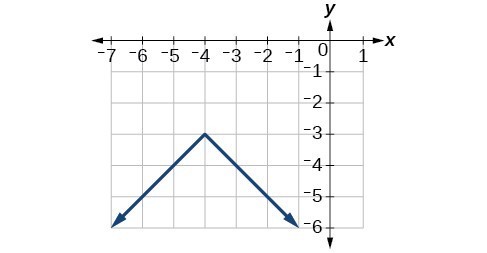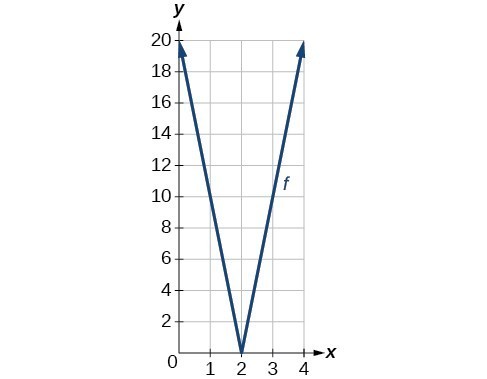37. Graph of an absolute function with points at (-1, 2), (0, 1), (1, 0), (2, 1), and (3, 2). 39. Graph of an absolute function with points at (-2, 3), (-1, 2), (0, 1), (1, 2), and (2, 3). 41. Graph of an absolute function. 43. Graph of an absolute function. 45. Graph of an absolute function. 47. Graph of an absolute function. 49. Graph of an absolute function. 51. Graph of an absolute function. 53. range: [latex]\left[0,20\right][/latex] Graph of an absolute function. 55. [latex]x\text{-}[/latex] intercepts: 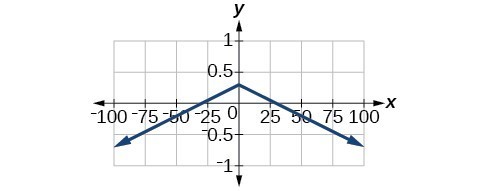Graph of an absolute function. 57. [latex]\left(-\infty ,\infty \right)[/latex] 59. There is no solution for [latex]a[/latex] that will keep the function from having a [latex]y[/latex] -intercept. The absolute value function always crosses the [latex]y[/latex] -intercept when [latex]x=0[/latex]. 61. [latex]|p - 0.08|\le 0.015[/latex] 63. [latex]|x - 5.0|\le 0.01[/latex]

Licenses & Attributions

CC licensed content, Shared previously

  • Precalculus. Provided by: OpenStax Authored by: Jay Abramson, et al.. Located at: https://openstax.org/books/precalculus/pages/1-introduction-to-functions. License: CC BY: Attribution. License terms: Download For Free at : http://cnx.org/contents/[email protected]..