We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Guías de estudio > College Algebra

Points and Lines in the Plane

Tracie set out from Elmhurst, IL, to go to Franklin Park. On the way, she made a few stops to do errands. Each stop is indicated by a red dot in Figure 1. Laying a rectangular coordinate grid over the map, we can see that each stop aligns with an intersection of grid lines. In this section, we will learn how to use grid lines to describe locations and changes in locations.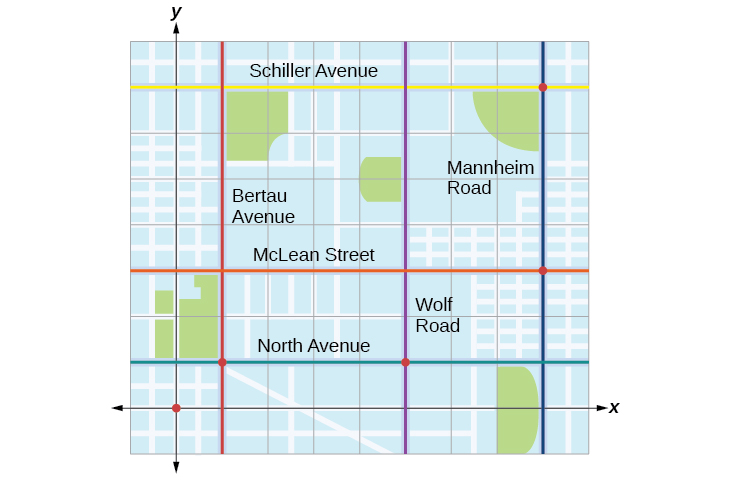
Road map of a city with street names on an x, y coordinate grid. Various points are marked in red on the grid lines indicating different locations on the map. Figure 1

Plot Points on the Coordinate Plane

An old story describes how seventeenth-century philosopher/mathematician René Descartes invented the system that has become the foundation of algebra while sick in bed. According to the story, Descartes was staring at a fly crawling on the ceiling when he realized that he could describe the fly’s location in relation to the perpendicular lines formed by the adjacent walls of his room. He viewed the perpendicular lines as horizontal and vertical axes. Further, by dividing each axis into equal unit lengths, Descartes saw that it was possible to locate any object in a two-dimensional plane using just two numbers—the displacement from the horizontal axis and the displacement from the vertical axis. While there is evidence that ideas similar to Descartes’ grid system existed centuries earlier, it was Descartes who introduced the components that comprise the Cartesian coordinate system, a grid system having perpendicular axes. Descartes named the horizontal axis the x-axis and the vertical axis the y-axis. The Cartesian coordinate system, also called the rectangular coordinate system, is based on a two-dimensional plane consisting of the x-axis and the y-axis. Perpendicular to each other, the axes divide the plane into four sections. Each section is called a quadrant; the quadrants are numbered counterclockwise as shown in Figure 2.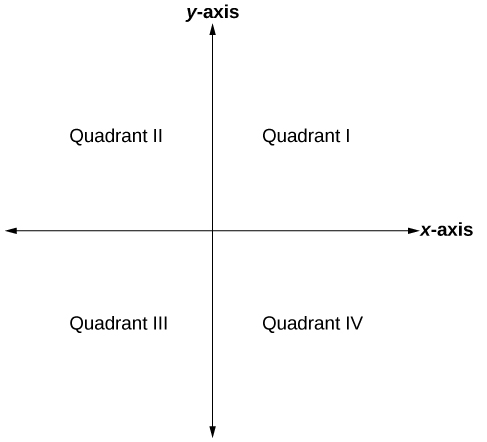
This is an image of an x, y plane with the axes labeled. The upper right section is labeled: Quadrant I. The upper left section is labeled: Quadrant II. The lower left section is labeled: Quadrant III. The lower right section is labeled: Quadrant IV. Figure 2
  The center of the plane is the point at which the two axes cross. It is known as the origin, or point [latex]\left(0,0\right)[/latex]. From the origin, each axis is further divided into equal units: increasing, positive numbers to the right on the x-axis and up the y-axis; decreasing, negative numbers to the left on the x-axis and down the y-axis. The axes extend to positive and negative infinity as shown by the arrowheads in Figure 3.
This is an image of an x, y coordinate plane. The x and y axis range from negative 5 to 5. Figure 3
Each point in the plane is identified by its x-coordinate, or horizontal displacement from the origin, and its y-coordinate, or vertical displacement from the origin. Together, we write them as an ordered pair indicating the combined distance from the origin in the form [latex]\left(x,y\right)[/latex]. An ordered pair is also known as a coordinate pair because it consists of x- and y-coordinates. For example, we can represent the point [latex]\left(3,-1\right)[/latex] in the plane by moving three units to the right of the origin in the horizontal direction, and one unit down in the vertical direction.
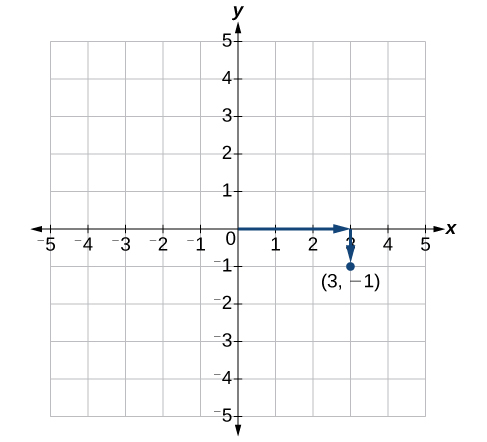This is an image of an x, y coordinate plane. The x and y axis range from negative 5 to 5. The point (3, -1) is labeled. An arrow extends rightward from the origin 3 units and another arrow extends downward one unit from the end of that arrow to the point. Figure 4
When dividing the axes into equally spaced increments, note that the x-axis may be considered separately from the y-axis. In other words, while the x-axis may be divided and labeled according to consecutive integers, the y-axis may be divided and labeled by increments of 2, or 10, or 100. In fact, the axes may represent other units, such as years against the balance in a savings account, or quantity against cost, and so on. Consider the rectangular coordinate system primarily as a method for showing the relationship between two quantities.

A General Note: Cartesian Coordinate System

A two-dimensional plane where the
  • x-axis is the horizontal axis
  • y-axis is the vertical axis
A point in the plane is defined as an ordered pair, [latex]\left(x,y\right)[/latex], such that x is determined by its horizontal distance from the origin and y is determined by its vertical distance from the origin.

Example: Plotting Points in a Rectangular Coordinate System

Plot the points [latex]\left(-2,4\right)[/latex], [latex]\left(3,3\right)[/latex], and [latex]\left(0,-3\right)[/latex] in the plane.

Answer: To plot the point [latex]\left(-2,4\right)[/latex], begin at the origin. The x-coordinate is –2, so move two units to the left. The y-coordinate is 4, so then move four units up in the positive y direction. To plot the point [latex]\left(3,3\right)[/latex], begin again at the origin. The x-coordinate is 3, so move three units to the right. The y-coordinate is also 3, so move three units up in the positive y direction. To plot the point [latex]\left(0,-3\right)[/latex], begin again at the origin. The x-coordinate is 0. This tells us not to move in either direction along the x-axis. The y-coordinate is –3, so move three units down in the negative y direction. See the graph in Figure 5.

This is an image of a graph on an x, y coordinate plane. The x and y axes range from negative 5 to 5. The points (-2, 4); (3, 3); and (0, -3) are labeled. Arrows extend from the origin to the points. Figure 5

Analysis of the Solution

Note that when either coordinate is zero, the point must be on an axis. If the x-coordinate is zero, the point is on the y-axis. If the y-coordinate is zero, the point is on the x-axis.

 

Distance in the Plane

Derived from the Pythagorean Theorem, the distance formula is used to find the distance between two points in the plane. The Pythagorean Theorem, [latex]{a}^{2}+{b}^{2}={c}^{2}[/latex], is based on a right triangle where a and b are the lengths of the legs adjacent to the right angle, and c is the length of the hypotenuse.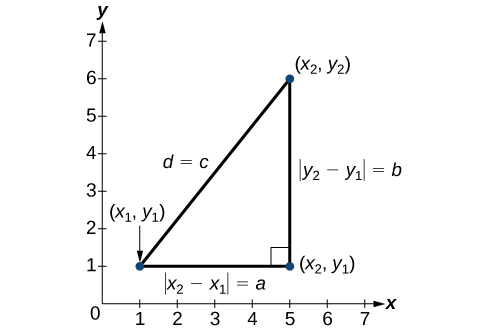 This is an image of a triangle on an x, y coordinate plane. The x and y axes range from 0 to 7. The points (x sub 1, y sub 1); (x sub 2, y sub 1); and (x sub 2, y sub 2) are labeled and connected to form a triangle. Along the base of the triangle, the following equation is displayed: the absolute value of x sub 2 minus x sub 1 equals a. The hypotenuse of the triangle is labeled: d = c. The remaining side is labeled: the absolute value of y sub 2 minus y sub 1 equals b. The relationship of sides [latex]|{x}_{2}-{x}_{1}|[/latex] and [latex]|{y}_{2}-{y}_{1}|[/latex] to side d is the same as that of sides a and b to side c. We use the absolute value symbol to indicate that the length is a positive number because the absolute value of any number is positive. (For example, [latex]|-3|=3[/latex]. ) The symbols [latex]|{x}_{2}-{x}_{1}|[/latex] and [latex]|{y}_{2}-{y}_{1}|[/latex] indicate that the lengths of the sides of the triangle are positive. To find the length c, take the square root of both sides of the Pythagorean Theorem.
[latex]{c}^{2}={a}^{2}+{b}^{2}\rightarrow c=\sqrt{{a}^{2}+{b}^{2}}[/latex]
It follows that the distance formula is given as
[latex]{d}^{2}={\left({x}_{2}-{x}_{1}\right)}^{2}+{\left({y}_{2}-{y}_{1}\right)}^{2}\to d=\sqrt{{\left({x}_{2}-{x}_{1}\right)}^{2}+{\left({y}_{2}-{y}_{1}\right)}^{2}}[/latex]
We do not have to use the absolute value symbols in this definition because any number squared is positive.

A General Note: The Distance Formula

Given endpoints [latex]\left({x}_{1},{y}_{1}\right)[/latex] and [latex]\left({x}_{2},{y}_{2}\right)[/latex], the distance between two points is given by
[latex]d=\sqrt{{\left({x}_{2}-{x}_{1}\right)}^{2}+{\left({y}_{2}-{y}_{1}\right)}^{2}}[/latex]

Example: Finding the Distance between Two Points

Find the distance between the points [latex]\left(-3,-1\right)[/latex] and [latex]\left(2,3\right)[/latex].

Answer: Let us first look at the graph of the two points. Connect the points to form a right triangle. This is an image of a triangle on an x, y coordinate plane. The x-axis ranges from negative 4 to 4. The y-axis ranges from negative 2 to 4. The points (-3, -1); (2, -1); and (2, 3) are plotted and labeled on the graph. The points are connected to form a triangle Then, calculate the length of d using the distance formula.

[latex]\begin{array}{l}d=\sqrt{{\left({x}_{2}-{x}_{1}\right)}^{2}+{\left({y}_{2}-{y}_{1}\right)}^{2}}\hfill \\ d=\sqrt{{\left(2-\left(-3\right)\right)}^{2}+{\left(3-\left(-1\right)\right)}^{2}}\hfill \\ =\sqrt{{\left(5\right)}^{2}+{\left(4\right)}^{2}}\hfill \\ =\sqrt{25+16}\hfill \\ =\sqrt{41}\hfill \end{array}[/latex]

 

Try It

Find the distance between two points: [latex]\left(1,4\right)[/latex] and [latex]\left(11,9\right)[/latex].

Answer: [latex]\sqrt{125}=5\sqrt{5}[/latex]

Example: Finding the Center of a Circle

The diameter of a circle has endpoints [latex]\left(-1,-4\right)[/latex] and [latex]\left(5,-4\right)[/latex]. Find the center of the circle.

Answer: The center of a circle is the center, or midpoint, of its diameter. Thus, the midpoint formula will yield the center point.

[latex]\begin{array}{c}\left(\frac{{x}_{1}+{x}_{2}}{2},\frac{{y}_{1}+{y}_{2}}{2}\right)\\ \left(\frac{-1+5}{2},\frac{-4 - 4}{2}\right)=\left(\frac{4}{2},-\frac{8}{2}\right)=\left(2,-4\right)\end{array}[/latex]

Graph Linear Equations

We can plot a set of points to represent an equation. When such an equation contains both an x variable and a y variable, it is called an equation in two variables. Its graph is called a graph in two variables. Any graph on a two-dimensional plane is a graph in two variables. Suppose we want to graph the equation [latex]y=2x - 1[/latex]. We can begin by substituting a value for x into the equation and determining the resulting value of y. Each pair of x- and y-values is an ordered pair that can be plotted. The table below lists values of x from –3 to 3 and the resulting values for y.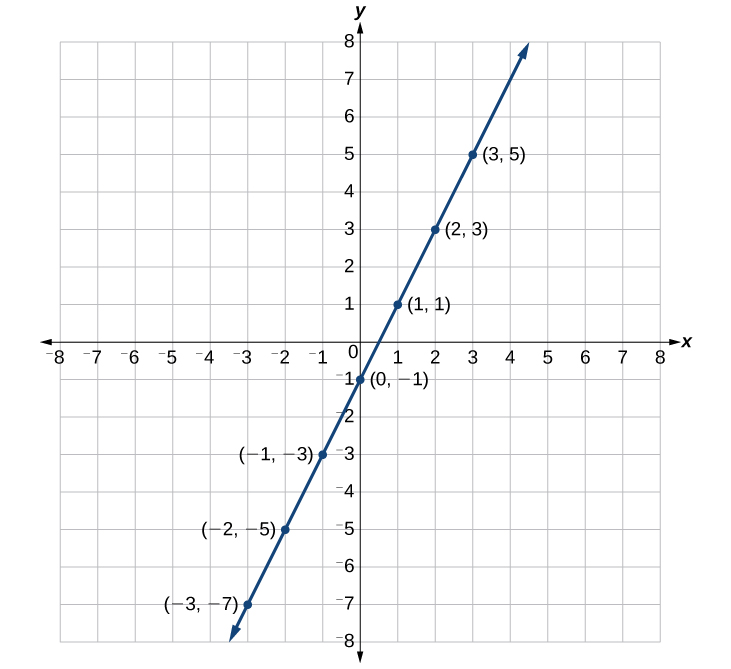
[latex]x[/latex] [latex]y=2x - 1[/latex] [latex]\left(x,y\right)[/latex]
[latex]-3[/latex] [latex]y=2\left(-3\right)-1=-7[/latex] [latex]\left(-3,-7\right)[/latex]
[latex]-2[/latex] [latex]y=2\left(-2\right)-1=-5[/latex] [latex]\left(-2,-5\right)[/latex]
[latex]-1[/latex] [latex]y=2\left(-1\right)-1=-3[/latex] [latex]\left(-1,-3\right)[/latex]
[latex]0[/latex] [latex]y=2\left(0\right)-1=-1[/latex] [latex]\left(0,-1\right)[/latex]
[latex]1[/latex] [latex]y=2\left(1\right)-1=1[/latex] [latex]\left(1,1\right)[/latex]
[latex]2[/latex] [latex]y=2\left(2\right)-1=3[/latex] [latex]\left(2,3\right)[/latex]
[latex]3[/latex] [latex]y=2\left(3\right)-1=5[/latex] [latex]\left(3,5\right)[/latex]
We can plot the points in the table. The points for this particular equation form a line, so we can connect them. This is not true for all equations. This is a graph of a line on an x, y coordinate plane. The x- and y-axis range from negative 8 to 8. A line passes through the points (-3, -7); (-2, -5); (-1, -3); (0, -1); (1, 1); (2, 3); and (3, 5). Note that the x-values chosen are arbitrary, regardless of the type of equation we are graphing. Of course, some situations may require particular values of x to be plotted in order to see a particular result. Otherwise, it is logical to choose values that can be calculated easily, and it is always a good idea to choose values that are both negative and positive. There is no rule dictating how many points to plot, although we need at least two to graph a line. Keep in mind, however, that the more points we plot, the more accurately we can sketch the graph.

How To: Given an equation, graph by plotting points.

  1. Make a table with one column labeled x, a second column labeled with the equation, and a third column listing the resulting ordered pairs.
  2. Enter x-values down the first column using positive and negative values. Selecting the x-values in numerical order will make the graphing simpler.
  3. Select x-values that will yield y-values with little effort, preferably ones that can be calculated mentally.
  4. Plot the ordered pairs.
  5. Connect the points if they form a line.

Example: Graphing an Equation in Two Variables by Plotting Points

Graph the equation [latex]y=-x+2[/latex] by plotting points.

Answer: First, we construct a table similar to the one below. Choose x values and calculate y.

[latex]x[/latex] [latex]y=-x+2[/latex] [latex]\left(x,y\right)[/latex]
[latex]-5[/latex] [latex]y=-\left(-5\right)+2=7[/latex] [latex]\left(-5,7\right)[/latex]
[latex]-3[/latex] [latex]y=-\left(-3\right)+2=5[/latex] [latex]\left(-3,5\right)[/latex]
[latex]-1[/latex] [latex]y=-\left(-1\right)+2=3[/latex] [latex]\left(-1,3\right)[/latex]
[latex]0[/latex] [latex]y=-\left(0\right)+2=2[/latex] [latex]\left(0,2\right)[/latex]
[latex]1[/latex] [latex]y=-\left(1\right)+2=1[/latex] [latex]\left(1,1\right)[/latex]
[latex]3[/latex] [latex]y=-\left(3\right)+2=-1[/latex] [latex]\left(3,-1\right)[/latex]
[latex]5[/latex] [latex]y=-\left(5\right)+2=-3[/latex] [latex]\left(5,-3\right)[/latex]
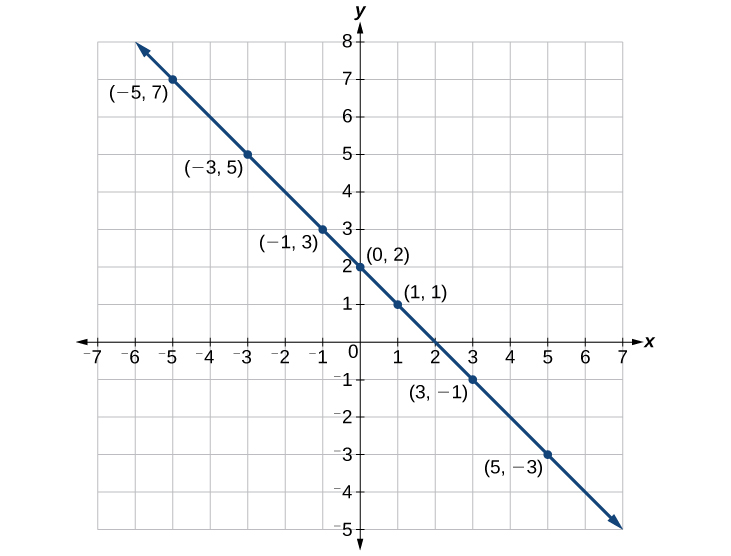
Now, plot the points. Connect them if they form a line. This image is a graph of a line on an x, y coordinate plane. The x-axis includes numbers that range from negative 7 to 7. The y-axis includes numbers that range from negative 5 to 8. A line passes through the points: (-5, 7); (-3, 5); (-1, 3); (0, 2); (1, 1); (3, -1); and (5, -3).

 

Try It

Construct a table and graph the equation by plotting points: [latex]y=\frac{1}{2}x+2[/latex].

Answer:

[latex]x[/latex] [latex]y=\frac{1}{2}x+2[/latex] [latex]\left(x,y\right)[/latex]
[latex]-2[/latex] [latex]y=\frac{1}{2}\left(-2\right)+2=1[/latex] [latex]\left(-2,1\right)[/latex]
[latex]-1[/latex] [latex]y=\frac{1}{2}\left(-1\right)+2=\frac{3}{2}[/latex] [latex]\left(-1,\frac{3}{2}\right)[/latex]
[latex]0[/latex] [latex]y=\frac{1}{2}\left(0\right)+2=2[/latex] [latex]\left(0,2\right)[/latex]
[latex]1[/latex] [latex]y=\frac{1}{2}\left(1\right)+2=\frac{5}{2}[/latex] [latex]\left(1,\frac{5}{2}\right)[/latex]
[latex]2[/latex] [latex]y=\frac{1}{2}\left(2\right)+2=3[/latex] [latex]\left(2,3\right)[/latex]
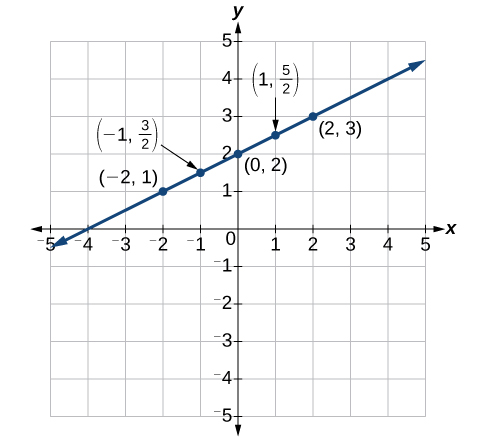
This is an image of a graph on an x, y coordinate plane. The x and y-axis range from negative 5 to 5. A line passes through the points (-2, 1); (-1, 3/2); (0, 2); (1, 5/2); and (2, 3).

Plot Points With a Graphing Utility

Most graphing calculators require similar techniques to graph an equation. The equations sometimes have to be manipulated so they are written in the style y=_____. The TI-84 Plus, and many other calculator makes and models, have a mode function, which allows the window (the screen for viewing the graph) to be altered so the pertinent parts of a graph can be seen. For example, the equation [latex]y=2x - 20[/latex] has been entered in the TI-84 Plus shown in Figure 9a. In Figure 9b, the resulting graph is shown. Notice that we cannot see on the screen where the graph crosses the axes. The standard window screen on the TI-84 Plus shows [latex]-10\le x\le 10[/latex], and [latex]-10\le y\le 10[/latex]. See Figure (c) below.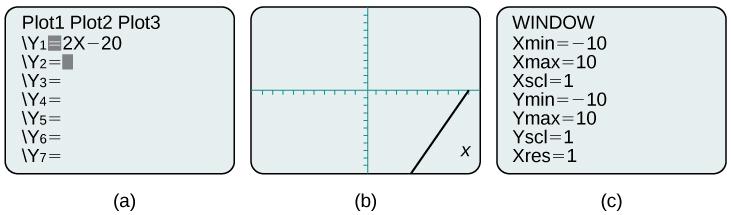
This is an image of three side-by-side calculator screen captures. The first screen is the plot screen with the function y sub 1 equals two times x minus twenty. The second screen shows the plotted line on the coordinate plane. The third screen shows the window edit screen with the following settings: Xmin = -10; Xmax = 10; Xscl = 1; Ymin = -10; Ymax = 10; Yscl = 1; Xres = 1. a. Enter the equation. b. This is the graph in the original window. c. These are the original settings.
By changing the window to show more of the positive x-axis and more of the negative y-axis, we have a much better view of the graph and the x- and y-intercepts. See (a) and (b) below.
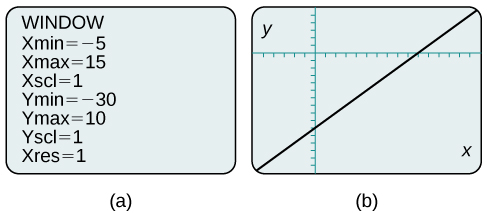This is an image of two side-by-side calculator screen captures. The first screen is the window edit screen with the following settings: Xmin = negative 5; Xmax = 15; Xscl = 1; Ymin = -30; Ymax = 10; Yscl = 1; Xres =1. The second screen shows the plot of the previous graph, but is more centered on the line. a. This screen shows the new window settings. b. We can clearly view the intercepts in the new window.

Example: Using a Graphing Utility to Graph an Equation

Use a graphing utility to graph the equation: [latex]y=-\frac{2}{3}x-\frac{4}{3}[/latex].

Answer: Enter the equation in the y= function of the calculator. Set the window settings so that both the x- and y- intercepts are showing in the window.

This image is of a line graph on an x, y coordinate plane. The x-axis has numbers that range from negative 3 to 4. The y-axis has numbers that range from negative 3 to 3. The function y = -2x/3 + 4/3 is plotted.

Key Concepts

  • We can locate, or plot, points in the Cartesian coordinate system using ordered pairs, which are defined as displacement from the x-axis and displacement from the y-axis.
  • An equation can be graphed in the plane by creating a table of values and plotting points.
  • Using a graphing calculator or a computer program makes graphing equations faster and more accurate. Equations usually have to be entered in the form y=_____.
  • Finding the x- and y-intercepts can define the graph of a line. These are the points where the graph crosses the axes.
  • The distance formula is derived from the Pythagorean Theorem and is used to find the length of a line segment.
  • The midpoint formula provides a method of finding the coordinates of the midpoint dividing the sum of the x-coordinates and the sum of the y-coordinates of the endpoints by 2.

Glossary

Cartesian coordinate system a grid system designed with perpendicular axes invented by René Descartes equation in two variables a mathematical statement, typically written in x and y, in which two expressions are equal graph in two variables the graph of an equation in two variables, which is always shown in two variables in the two-dimensional plane intercepts the points at which the graph of an equation crosses the x-axis and the y-axis ordered pair a pair of numbers indicating horizontal displacement and vertical displacement from the origin; also known as a coordinate pair, [latex]\left(x,y\right)[/latex] origin the point where the two axes cross in the center of the plane, described by the ordered pair [latex]\left(0,0\right)[/latex] quadrant one quarter of the coordinate plane, created when the axes divide the plane into four sections x-axis the common name of the horizontal axis on a coordinate plane; a number line increasing from left to right x-coordinate the first coordinate of an ordered pair, representing the horizontal displacement and direction from the origin x-intercept the point where a graph intersects the x-axis; an ordered pair with a y-coordinate of zero y-axis the common name of the vertical axis on a coordinate plane; a number line increasing from bottom to top y-coordinate the second coordinate of an ordered pair, representing the vertical displacement and direction from the origin y-intercept a point where a graph intercepts the y-axis; an ordered pair with an x-coordinate of zero   distance formula a formula that can be used to find the length of a line segment if the endpoints are known midpoint formula a formula to find the point that divides a line segment into two parts of equal length

 

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
  • Question ID 92752, 92753, 92757. Authored by: Michael Jenck. License: CC BY: Attribution. License terms: IMathAS Community License, CC-BY + GPL.
  • Question ID 19140. Authored by: Amy Lambert. License: CC BY: Attribution. License terms: IMathAS Community LicenseCC-BY + GPL.
  • Question ID 2308. Authored by: David Lippman. License: CC BY: Attribution. License terms: IMathAS Community License, CC-BY + GPL.
  • Question ID 110938, 110939. Authored by: Lumen Learning. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.