Applications of Systems of Linear Inequalities
Learning Outcomes
- Write and graph a system that models the quantity that must be sold to achieve a given amount of sales
Example
Cathy is selling ice cream cones at a school fundraiser. She is selling two sizes: small (which has scoop) and large (which has scoops). She knows that she can get a maximum of scoops of ice cream out of her supply. She charges $3 for a small cone and $5 for a large cone. Cathy wants to earn at least $120 to give back to the school. Write and graph a system of inequalities that models this situation.Answer: First, identify the variables. There are two variables: the number of small cones sold and the number of large cones sold.
s = the number of small cones sold
l = the number of large cones sold
Write the first equation: the maximum number of scoops she can give out. The scoops she has available must be greater than or equal to the number of scoops used to make the small cones and the large cones she sells. That is, scoop each times the number of small cones sold together with scoops each times the number of large cones sold cannot exceed the total supply of 70 scoops.
Write the second equation: the amount of money she raises. She wants the total amount of money earned from small cones, $3 times the number sold, and large cones $5 times the number sold to be at least $120.
Write the system.
Now graph the system. The variables x and y have been replaced by s and l; graph s along the x-axis and l along the y-axis. First graph the region . Graph the boundary line and then test individual points to see which region to shade. We only shade the regions that also satisfy , . The graph is shown below.
 Now graph the region Graph the boundary line and then test individual points to see which region to shade. The graph is shown below.
Now graph the region Graph the boundary line and then test individual points to see which region to shade. The graph is shown below.
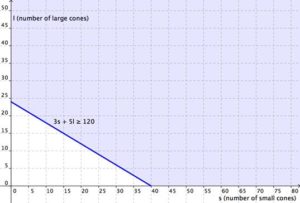 Graphing the regions together, you find the following:
Graphing the regions together, you find the following:
 Looking at just the overlapping region, you have:
Looking at just the overlapping region, you have:
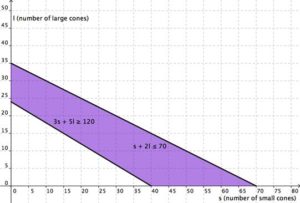 The region in purple is the solution. As long as the combination of the number of small cones and large cones that Cathy sells can be mapped in the purple region, she will have earned at least $120 and not used more than scoops of ice cream.
The region in purple is the solution. As long as the combination of the number of small cones and large cones that Cathy sells can be mapped in the purple region, she will have earned at least $120 and not used more than scoops of ice cream.
