We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > College Algebra CoRequisite Course

Characteristics of Rational Functions

Learning Outcomes

  • Use arrow notation to describe local and end behavior of rational functions.
  • Identify horizontal and vertical asymptotes of rational functions from graphs.
  • Graph a rational function given horizontal and vertical shifts.
  • Solve applied problems involving rational functions.

Using Arrow Notation

recall Characteristics of rational expressions

  • We've seen that rational expressions are fractions that may contain a polynomial in the numerator, denominator, or both.
  • A rational function is a function whose argument is defined using a rational expression.
  • When a variable is present in the denominator of a rational expression, certain values of the variable may cause the denominator to equal zero. A rational expression with a zero in the denominator is not defined since we cannot divide by zero.
  • We'll see in this section that the values of the input to a rational function causing the denominator to equal zero will have an impact on the shape of the function's graph. The graph appears to "bend around" these input values, and the function value increases or decreases without bound near those inputs.
  • See the graphs below for how to use a notation called arrow notation to describe this and other behaviors sometimes present in the graphs of functions. ​
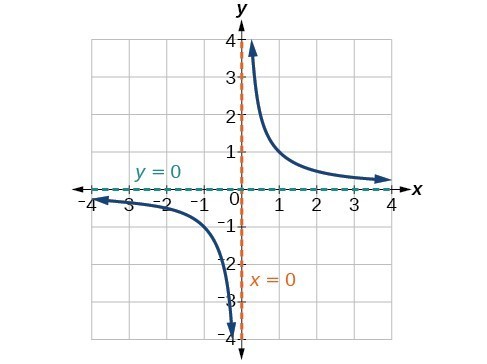
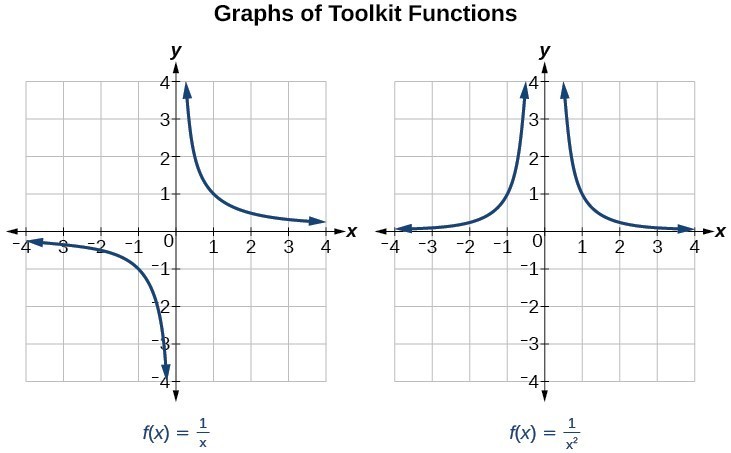We have seen the graphs of the basic reciprocal function and the squared reciprocal function from our study of toolkit functions. Examine these graphs and notice some of their features. Graphs of f(x)=1/x and f(x)=1/x^2 Several things are apparent if we examine the graph of [latex]f\left(x\right)=\dfrac{1}{x}[/latex].
  1. On the left branch of the graph, the curve approaches the [latex]x[/latex]-axis [latex]\left(y=0\right) \text{ as } x\to -\infty [/latex].
  2. As the graph approaches [latex]x=0[/latex] from the left, the curve drops, but as we approach zero from the right, the curve rises.
  3. Finally, on the right branch of the graph, the curves approaches the [latex]x[/latex]-axis [latex]\left(y=0\right) \text{ as } x\to \infty [/latex].
To summarize, we use arrow notation to show that [latex]x[/latex] or [latex]f\left(x\right)[/latex] is approaching a particular value.
Arrow Notation
Symbol Meaning
[latex]x\to {a}^{-}[/latex] [latex]x[/latex] approaches [latex]a[/latex] from the left ([latex]x<a[/latex] but close to [latex]a[/latex])
[latex]x\to {a}^{+}[/latex] [latex]x[/latex] approaches [latex]a[/latex] from the right ([latex]x<a[/latex] but close to [latex]a[/latex])
[latex]x\to \infty[/latex] [latex]x[/latex] approaches infinity ([latex]x[/latex] increases without bound)
[latex]x\to -\infty [/latex] [latex]x[/latex] approaches negative infinity ([latex]x[/latex] decreases without bound)
[latex]f\left(x\right)\to \infty [/latex] the output approaches infinity (the output increases without bound)
[latex]f\left(x\right)\to -\infty [/latex] the output approaches negative infinity (the output decreases without bound)
[latex]f\left(x\right)\to a[/latex] the output approaches [latex]a[/latex]

Local Behavior of [latex]f\left(x\right)=\frac{1}{x}[/latex]

Let’s begin by looking at the reciprocal function, [latex]f\left(x\right)=\frac{1}{x}[/latex]. We cannot divide by zero, which means the function is undefined at [latex]x=0[/latex]; so zero is not in the domain. As the input values approach zero from the left side (becoming very small, negative values), the function values decrease without bound (in other words, they approach negative infinity). We can see this behavior in the table below.
[latex]x[/latex] –0.1 –0.01 –0.001 –0.0001
[latex]f\left(x\right)=\frac{1}{x}[/latex] –10 –100 –1000 –10,000
We write in arrow notation

[latex]\text{as }x\to {0}^{-},f\left(x\right)\to -\infty [/latex]

As the input values approach zero from the right side (becoming very small, positive values), the function values increase without bound (approaching infinity). We can see this behavior in the table below.
[latex]x[/latex] 0.1 0.01 0.001 0.0001
[latex]f\left(x\right)=\frac{1}{x}[/latex] 10 100 1000 10,000
We write in arrow notation

[latex]\text{As }x\to {0}^{+}, f\left(x\right)\to \infty [/latex].

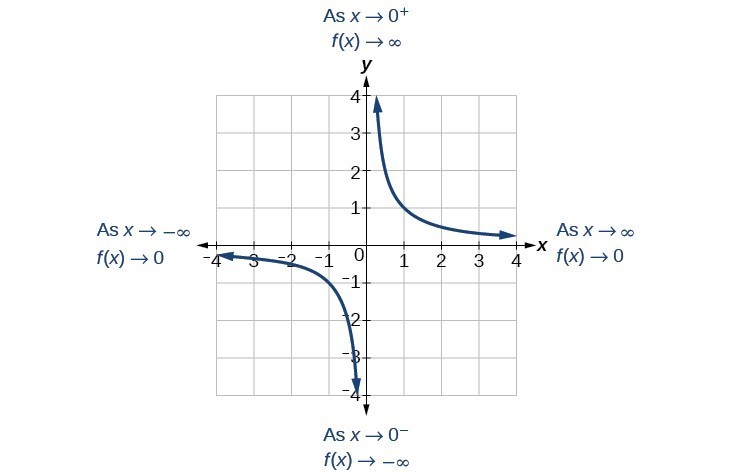Graph of f(x)=1/x which denotes the end behavior. As x goes to negative infinity, f(x) goes to 0, and as x goes to 0^-, f(x) goes to negative infinity. As x goes to positive infinity, f(x) goes to 0, and as x goes to 0^+, f(x) goes to positive infinity. This behavior creates a vertical asymptote, which is a vertical line that the graph approaches but never crosses. In this case, as the input nears zero from the left, the function value decreases without bound. As the input nears zero from the right, the function value increases without bound. The line [latex]x=0[/latex] is a vertical asymptote for the function. 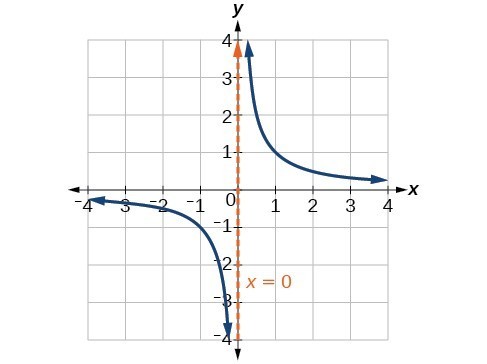Graph of f(x)=1/x with its vertical asymptote at x=0.

A General Note: Vertical Asymptote

A vertical asymptote of a graph is a vertical line [latex]x=a[/latex] where the graph tends toward positive or negative infinity as the inputs approach [latex]x[/latex]. We write [latex]\text{As }x\to a,f\left(x\right)\to \infty , \text{or as }x\to a,f\left(x\right)\to -\infty [/latex].

Asymptote.  what does that word mean?

The term asymptote has its origins from three Greek roots.

a, meaning not

sun, meaning together

ptotos, meaning to fall

These give us asymptotos, meaning not falling together, which leads to the modern term describing a line that a curve (the graph of a function) approaches but never meets.

End Behavior of [latex]f\left(x\right)=\frac{1}{x}[/latex]

As the values of [latex]x[/latex] approach infinity, the function values approach 0. As the values of [latex]x[/latex] approach negative infinity, the function values approach 0. Symbolically, using arrow notation

[latex]\text{As }x\to \infty ,f\left(x\right)\to 0,\text{and as }x\to -\infty ,f\left(x\right)\to 0[/latex].

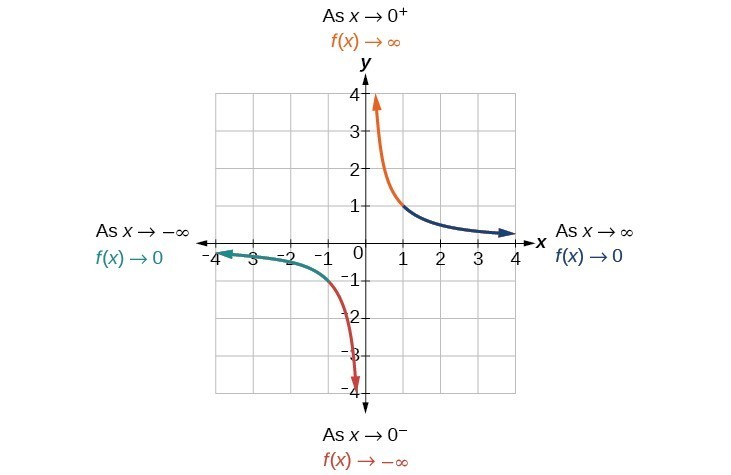Graph of f(x)=1/x which highlights the segments of the turning points to denote their end behavior. Based on this overall behavior and the graph, we can see that the function approaches 0 but never actually reaches 0; it seems to level off as the inputs become large. This behavior creates a horizontal asymptote, a horizontal line that the graph approaches as the input increases or decreases without bound. In this case, the graph is approaching the horizontal line [latex]y=0[/latex]. Graph of f(x)=1/x with its vertical asymptote at x=0 and its horizontal asymptote at y=0.

A General Note: Horizontal Asymptote

A horizontal asymptote of a graph is a horizontal line [latex]y=b[/latex] where the graph approaches the line as the inputs increase or decrease without bound. We write [latex]\text{As }x\to \infty \text{ or }x\to -\infty ,\text{ }f\left(x\right)\to b[/latex].

Example: Using Arrow Notation

Use arrow notation to describe the end behavior and local behavior of the function below.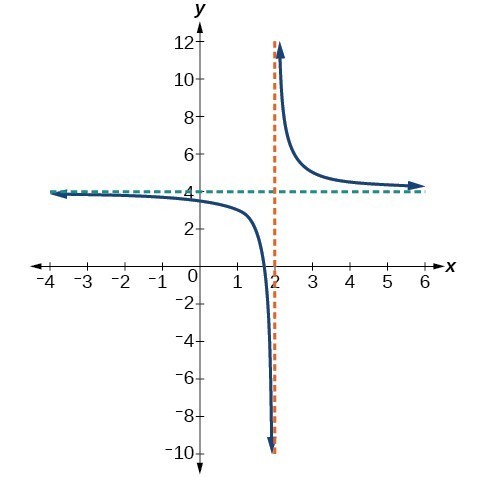 Graph of f(x)=1/(x-2)+4 with its vertical asymptote at x=2 and its horizontal asymptote at y=4.

Answer: Notice that the graph is showing a vertical asymptote at [latex]x=2[/latex], which tells us that the function is undefined at [latex]x=2[/latex].

As [latex]x\to {2}^{-},\hspace{2mm}f\left(x\right)\to -\infty[/latex], and as [latex]x\to {2}^{+},\text{ }f\left(x\right)\to \infty [/latex]

And as the inputs decrease without bound, the graph appears to be leveling off at output values of 4, indicating a horizontal asymptote at [latex]y=4[/latex]. As the inputs increase without bound, the graph levels off at 4.

As [latex]x\to \infty ,\text{ }f\left(x\right)\to 4[/latex], and as [latex]x\to -\infty ,\text{ }f\left(x\right)\to 4[/latex]

Try It

Use arrow notation to describe the end behavior and local behavior for the reciprocal squared function.

Answer: End behavior: as [latex]x\to \pm \infty , f\left(x\right)\to 0[/latex]; Local behavior: as [latex]x\to 0, f\left(x\right)\to \infty [/latex] (there are no [latex]x[/latex]- or [latex]y[/latex]-intercepts)

[ohm_question]129042[/ohm_question]

Example: Using Transformations to Graph a Rational Function

Sketch a graph of the reciprocal function shifted two units to the left and up three units. Identify the horizontal and vertical asymptotes of the graph, if any.

Answer: Shifting the graph left 2 and up 3 would result in the function

[latex]f\left(x\right)=\dfrac{1}{x+2}+3[/latex]

or equivalently, by giving the terms a common denominator,

[latex]f\left(x\right)=\dfrac{3x+7}{x+2}[/latex]

The graph of the shifted function is displayed below. 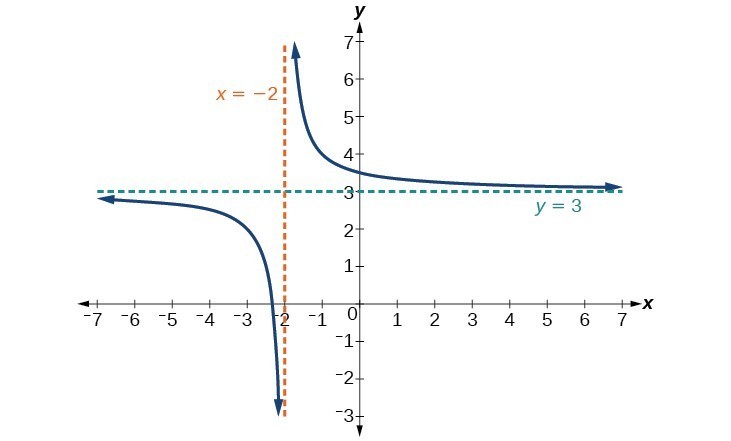Graph of f(x)=1/(x+2)+3 with its vertical asymptote at x=-2 and its horizontal asymptote at y=3. Notice that this function is undefined at [latex]x=-2[/latex], and the graph also is showing a vertical asymptote at [latex]x=-2[/latex].

As [latex]x\to -{2}^{-}, f\left(x\right)\to -\infty[/latex] , and as  [latex]x\to -{2}^{+}, f\left(x\right)\to \infty [/latex]

As the inputs increase and decrease without bound, the graph appears to be leveling off at output values of 3, indicating a horizontal asymptote at [latex]y=3[/latex].

[latex]\text{As }x\to \pm \infty , f\left(x\right)\to 3[/latex]

Analysis of the Solution

Notice that horizontal and vertical asymptotes are shifted left 2 and up 3 along with the function.

Try It

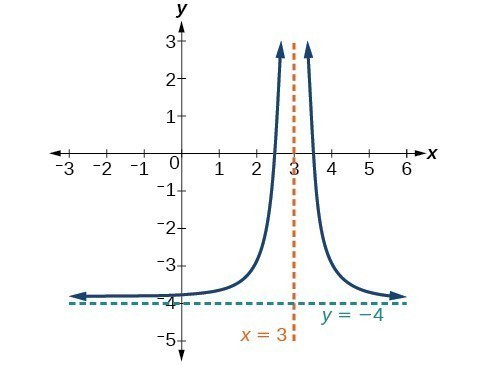
Sketch the graph, and find the horizontal and vertical asymptotes of the reciprocal squared function that has been shifted right 3 units and down 4 units.

Answer: The function and the asymptotes are shifted 3 units right and 4 units down. As [latex]x\to 3,f\left(x\right)\to \infty[/latex], and as [latex]x\to \pm \infty ,f\left(x\right)\to -4[/latex].

The function is [latex]f\left(x\right)=\dfrac{1}{{\left(x - 3\right)}^{2}}-4[/latex].

Graph of f(x)=1/(x-3)^2-4 with its vertical asymptote at x=3 and its horizontal asymptote at y=-4.

Solve Applied Problems Involving Rational Functions

In the previous example, we shifted a toolkit function in a way that resulted in the function [latex]f\left(x\right)=\dfrac{3x+7}{x+2}[/latex]. This is an example of a rational function. A rational function is a function that can be written as the quotient of two polynomial functions. Many real-world problems require us to find the ratio of two polynomial functions. Problems involving rates and concentrations often involve rational functions.

A General Note: Rational Function

A rational function is a function that can be written as the quotient of two polynomial functions [latex]P\left(x\right) \text{and} Q\left(x\right)[/latex].

[latex]f\left(x\right)=\dfrac{P\left(x\right)}{Q\left(x\right)}=\dfrac{{a}_{p}{x}^{p}+{a}_{p - 1}{x}^{p - 1}+...+{a}_{1}x+{a}_{0}}{{b}_{q}{x}^{q}+{b}_{q - 1}{x}^{q - 1}+...+{b}_{1}x+{b}_{0}},Q\left(x\right)\ne 0[/latex]

Summary: Horizontal and Vertical asymptotes

Horizontal Asymptote
  • A horizontal line of the form [latex]y=c[/latex]
  • The constant [latex]c[/latex] represents a number that the function value (output) approaches in the long run, either as the input grows very small or very large.
  • Horizontal asymptotes represent the long-run behavior (the end behavior) of the graph of the funtion.
  • A function's graph may cross a horizontal asymptote briefly, even more than once, but will eventually settle down near it, as the value of the function approaches the constant [latex]c[latex].
Vertical Asymptote
  • A vertical line of the form [latex]x=a[/latex]
  • The constant [latex]a[/latex] represents an input for which the function value (output) is undefined.
  • Substituting the value of [latex]a[/latex] into the function will result in a zero in the function's denominator.
  • The graph of the function "bends around", either increasing or decreasing without bound as the input nears [latex]a[/latex]
  • A function's graph will never cross a vertical asymptote.

Example: Solving an Applied Problem Involving a Rational Function

A large mixing tank currently contains 100 gallons of water into which 5 pounds of sugar have been mixed. A tap will open pouring 10 gallons per minute of water into the tank at the same time sugar is poured into the tank at a rate of 1 pound per minute. Find the concentration (pounds per gallon) of sugar in the tank after 12 minutes. Is that a greater concentration than at the beginning?

Answer: Let [latex]t[/latex] be the number of minutes since the tap opened. Since the water increases at 10 gallons per minute, and the sugar increases at 1 pound per minute, these are constant rates of change. This tells us the amount of water in the tank is changing linearly, as is the amount of sugar in the tank. We can write an equation independently for each:

[latex]\text{water: }W\left(t\right)=100+10t\text{ in gallons}[/latex]

[latex]\text{sugar: }S\left(t\right)=5+1t\text{ in pounds}[/latex]

The concentration, [latex]C[/latex], will be the ratio of pounds of sugar to gallons of water

[latex]C\left(t\right)=\dfrac{5+t}{100+10t}[/latex]

The concentration after 12 minutes is given by evaluating [latex]C\left(t\right)[/latex] at [latex]t=12[/latex].

[latex]\begin{align}C\left(12\right)&=\dfrac{5+12}{100+10\left(12\right)}\\&=\dfrac{17}{220}\end{align}[/latex]

This means the concentration is 17 pounds of sugar to 220 gallons of water. At the beginning the concentration is

[latex]\begin{align}C\left(0\right)&=\dfrac{5+0}{100+10\left(0\right)} \\ &=\dfrac{1}{20}\hfill \end{align}[/latex]

Since [latex]\frac{17}{220}\approx 0.08>\frac{1}{20}=0.05[/latex], the concentration is greater after 12 minutes than at the beginning.

Analysis of the Solution

To find the horizontal asymptote, divide the leading coefficient in the numerator by the leading coefficient in the denominator:

[latex]\dfrac{1}{10}=0.1[/latex]

Notice the horizontal asymptote is [latex]y=0.1[/latex]. This means the concentration, [latex]C[/latex], the ratio of pounds of sugar to gallons of water, will approach 0.1 in the long term.

Try It

There are 1,200 freshmen and 1,500 sophomores at a prep rally at noon. After 12 p.m., 20 freshmen arrive at the rally every five minutes while 15 sophomores leave the rally. Find the ratio of freshmen to sophomores at 1 p.m.

Answer: [latex-display]\dfrac{12}{11}[/latex-display]

[ohm_question]129067[/ohm_question]

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • Question ID 129042, 129067. Authored by: Day, Alyson. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
  • College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].