Express and Plot Complex Numbers
Learning Outcomes
- Express square roots of negative numbers as multiples of [latex]i[/latex].
- Plot complex numbers on the complex plane.
[latex]\sqrt{-1}=i[/latex]
So, using properties of radicals,[latex]{i}^{2}={\left(\sqrt{-1}\right)}^{2}=-1[/latex]
We can write the square root of any negative number as a multiple of [latex]i[/latex]. Consider the square root of –25.[latex]\begin{align}\sqrt{-25}&=\sqrt{25\cdot \left(-1\right)}\\&=\sqrt{25}\cdot\sqrt{-1}\\ &=5i\end{align}[/latex]
We use [latex]5i[/latex] and not [latex]-\text{5}i[/latex] because the principal root of 25 is the positive root. A complex number is the sum of a real number and an imaginary number. A complex number is expressed in standard form when written [latex]a+bi[/latex] where [latex]a[/latex] is the real part and [latex]bi[/latex] is the imaginary part. For example, [latex]5+2i[/latex] is a complex number. So, too, is [latex]3+4\sqrt{3}i[/latex].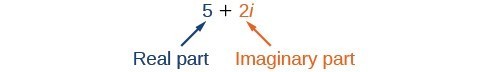 Imaginary numbers are distinguished from real numbers because a squared imaginary number produces a negative real number. Recall, when a positive real number is squared, the result is a positive real number and when a negative real number is squared, again, the result is a positive real number. Complex numbers are a combination of real and imaginary numbers.
Imaginary numbers are distinguished from real numbers because a squared imaginary number produces a negative real number. Recall, when a positive real number is squared, the result is a positive real number and when a negative real number is squared, again, the result is a positive real number. Complex numbers are a combination of real and imaginary numbers.
A General Note: Imaginary and Complex Numbers
A complex number is a number of the form [latex]a+bi[/latex] where- [latex]a[/latex] is the real part of the complex number.
- [latex]bi[/latex] is the imaginary part of the complex number.
How To: Given an imaginary number, express it in standard form.
- Write [latex]\sqrt{-a}[/latex] as [latex]\sqrt{a}\cdot\sqrt{-1}[/latex].
- Express [latex]\sqrt{-1}[/latex] as i.
- Write [latex]\sqrt{a}\cdot i[/latex] in simplest form.
recall writing square roots in simplest form
Recall that the principal square root of [latex]a[/latex] is the nonnegative number that, when multiplied by itself, equals [latex]a[/latex]. It is written as a radical expression, with a symbol called a radical over the term called the radicand: [latex]\sqrt{a}[/latex].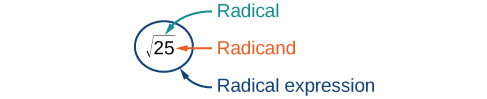 To simplify a square root, we rewrite it such that there are no perfect squares in the radicand. Use the product rule for simplifying square roots, which allows us to separate the square root of a product of two numbers into the product of two separate rational expressions. For instance, we can rewrite [latex]\sqrt{-75}[/latex] as [latex]\sqrt{25}\cdot \sqrt{3}\cdot \sqrt{-1} = 5 \sqrt{3}i[/latex].
To simplify a square root, we rewrite it such that there are no perfect squares in the radicand. Use the product rule for simplifying square roots, which allows us to separate the square root of a product of two numbers into the product of two separate rational expressions. For instance, we can rewrite [latex]\sqrt{-75}[/latex] as [latex]\sqrt{25}\cdot \sqrt{3}\cdot \sqrt{-1} = 5 \sqrt{3}i[/latex].
Example: Expressing an Imaginary Number in Standard Form
Express [latex]\sqrt{-9}[/latex] in standard form.Answer: [latex-display]\sqrt{-9}=\sqrt{9}\cdot\sqrt{-1}=3i[/latex-display] In standard form, this is [latex]0+3i[/latex].
Try It
Express [latex]\sqrt{-24}[/latex] in standard form.Answer: [latex-display]\sqrt{-24}=2i\sqrt{6}[/latex-display]
[ohm_question]61706[/ohm_question]Plot complex numbers on the complex plane
We cannot plot complex numbers on a number line as we might real numbers. However, we can still represent them graphically. To represent a complex number we need to address the two components of the number. We use the complex plane, which is a coordinate system in which the horizontal axis represents the real component and the vertical axis represents the imaginary component. Complex numbers are the points on the plane, expressed as ordered pairs [latex](a, b)[/latex], where [latex]a[/latex] represents the coordinate for the horizontal axis and [latex]b[/latex] represents the coordinate for the vertical axis.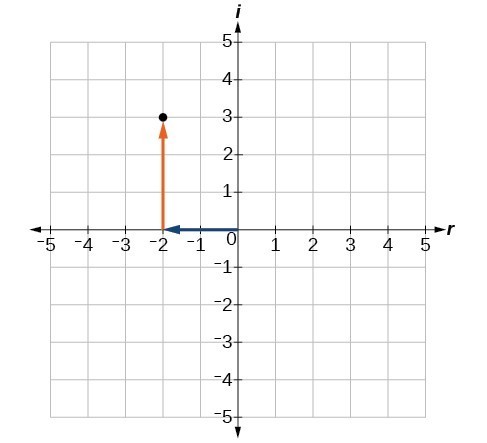 Let’s consider the number [latex]-2+3i[/latex]. The real part of the complex number is [latex]–2[/latex] and the imaginary part is [latex]3i[/latex]. We plot the ordered pair [latex]\left(-2,3\right)[/latex] to represent the complex number [latex]-2+3i[/latex].
Let’s consider the number [latex]-2+3i[/latex]. The real part of the complex number is [latex]–2[/latex] and the imaginary part is [latex]3i[/latex]. We plot the ordered pair [latex]\left(-2,3\right)[/latex] to represent the complex number [latex]-2+3i[/latex].
A General Note: Complex Plane
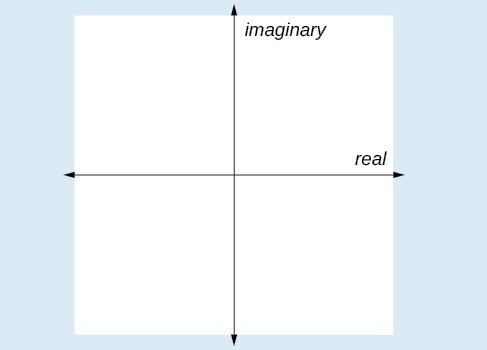 In the complex plane, the horizontal axis is the real axis, and the vertical axis is the imaginary axis.
In the complex plane, the horizontal axis is the real axis, and the vertical axis is the imaginary axis.
How To: Given a complex number, represent its components on the complex plane.
- Determine the real part and the imaginary part of the complex number.
- Move along the horizontal axis to show the real part of the number.
- Move parallel to the vertical axis to show the imaginary part of the number.
- Plot the point.
Example: Plotting a Complex Number on the Complex Plane
Plot the complex number [latex]3 - 4i[/latex] on the complex plane.Answer:
The real part of the complex number is [latex]3[/latex], and the imaginary part is [latex]–4i[/latex]. We plot the ordered pair [latex]\left(3,-4\right)[/latex].

Try It
Plot the complex number [latex]-4-i[/latex] on the complex plane. [ohm_question]65079[/ohm_question]Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- Introduction to Complex Numbers. Authored by: Sousa, James. License: CC BY: Attribution.
- Question ID 61706. Authored by: Day, Alyson. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 65709. Authored by: Kaslik,Pete, mb Lippman,David. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
CC licensed content, Specific attribution
- College Algebra. Provided by: OpenStax Authored by: OpenStax College Algebra. License: CC BY: Attribution.

