Factoring and the Square Root Property
Learning Outcomes
- Factor a quadratic equation to solve it.
- Use the square root property to solve a quadratic equation.
- Use the Pythagorean Theorem and the square root property to find the unknown length of the side of a right triangle.
Recall: identifying polynomials
Recall that a polynomial is an expression containing variable terms joined together by addition or subtraction. We can identify the degree of the polynomial by finding the term having the highest power (largest exponent on its variable). That term will be the leading term of the polynomial and its degree is the degree of the polynomial.[latex]\begin{array}{l}\left(x - 2\right)\left(x+3\right)\hfill&={x}^{2}+3x - 2x - 6\hfill \\ \hfill&={x}^{2}+x - 6\hfill \end{array}[/latex]
The product is a quadratic expression. Set equal to zero, [latex]{x}^{2}+x - 6=0[/latex] is a quadratic equation. If we were to factor the equation, we would get back the factors we multiplied.
The process of factoring a quadratic equation depends on the leading coefficient, whether it is 1 or another integer. We will look at both situations; but first, we want to confirm that the equation is written in standard form, [latex]a{x}^{2}+bx+c=0[/latex], where a, b, and c are real numbers and [latex]a\ne 0[/latex]. The equation [latex]{x}^{2}+x - 6=0[/latex] is in standard form.
recall the greatest common factor
The greatest common factor (GCF) is the largest number or variable expression that can divided evenly into two or more numbers or expressions. To find the GCF of an expression containing variable terms, first find the GCF of the coefficients, then find the GCF of the variables. The GCF of the variables will be the smallest degree of each variable that appears in each term. For example, [latex]4x[/latex] is the GCF of [latex]16x[/latex] and [latex]20{x}^{2}[/latex] because it is the largest expression that divides evenly into both [latex]16x[/latex] and [latex]20{x}^{2}[/latex]. When factoring, remember to look first for a greatest common factor. If you can find one to factor out, it will usually make your factoring task less challenging.A General Note: The Zero-Product Property and Quadratic Equations
The zero-product property states[latex]\text{If }a\cdot b=0,\text{ then }a=0\text{ or }b=0[/latex],
where a and b are real numbers or algebraic expressions.
A quadratic equation is an equation containing a second-degree polynomial; for example
[latex]a{x}^{2}+bx+c=0[/latex]
where a, b, and c are real numbers, and [latex]a\ne 0[/latex]. It is in standard form.
Solving Quadratics with a Leading Coefficient of 1
In the quadratic equation [latex]{x}^{2}+x - 6=0[/latex], the leading coefficient, or the coefficient of [latex]{x}^{2}[/latex], is 1. We have one method of factoring quadratic equations in this form.How To: Given a quadratic equation with the leading coefficient of 1, factor it
- Find two numbers whose product equals c and whose sum equals b.
- Use those numbers to write two factors of the form [latex]\left(x+k\right)\text{ or }\left(x-k\right)[/latex], where k is one of the numbers found in step 1. Use the numbers exactly as they are. In other words, if the two numbers are 1 and [latex]-2[/latex], the factors are [latex]\left(x+1\right)\left(x - 2\right)[/latex].
- Solve using the zero-product property by setting each factor equal to zero and solving for the variable.
Example: Factoring and Solving a Quadratic with Leading Coefficient of 1
Factor and solve the equation: [latex]{x}^{2}+x - 6=0[/latex].Answer: To factor [latex]{x}^{2}+x - 6=0[/latex], we look for two numbers whose product equals [latex]-6[/latex] and whose sum equals 1. Begin by looking at the possible factors of [latex]-6[/latex].
[latex]\begin{array}{l}1\cdot \left(-6\right)\hfill \\ \left(-6\right)\cdot 1\hfill \\ 2\cdot \left(-3\right)\hfill \\ 3\cdot \left(-2\right)\hfill \end{array}[/latex]
The last pair, [latex]3\cdot \left(-2\right)[/latex] sums to 1, so these are the numbers. Note that only one pair of numbers will work. Then, write the factors.
[latex]\left(x - 2\right)\left(x+3\right)=0[/latex]
To solve this equation, we use the zero-product property. Set each factor equal to zero and solve.
[latex]\begin{array}{l}\left(x - 2\right)\left(x+3\right)=0\hfill \\ \left(x - 2\right)=0\hfill \\ x=2\hfill \\ \left(x+3\right)=0\hfill \\ x=-3\hfill \end{array}[/latex]
The two solutions are [latex]x=2[/latex] and [latex]x=-3[/latex]. We can see how the solutions relate to the graph below. The solutions are the x-intercepts of the graph of [latex]{x}^{2}+x - 6[/latex].
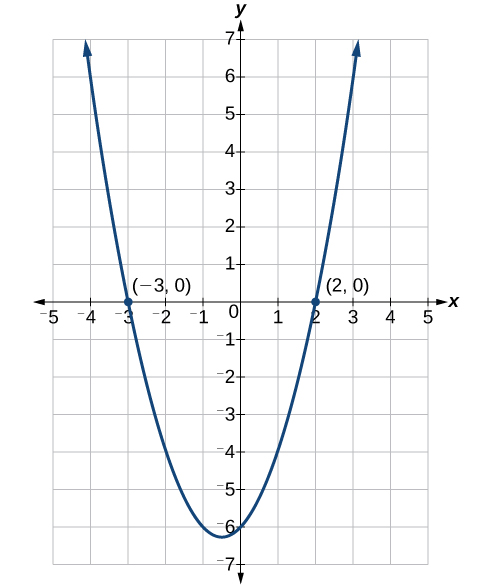
Try It
Factor and solve the quadratic equation: [latex]{x}^{2}-5x - 6=0[/latex].Answer: [latex]\left(x - 6\right)\left(x+1\right)=0;x=6,x=-1[/latex]
[ohm_question]2029[/ohm_question]Using the Square Root Property
When there is no linear term in the equation, another method of solving a quadratic equation is by using the square root property, in which we isolate the [latex]{x}^{2}[/latex] term and take the square root of the number on the other side of the equal sign. Keep in mind that sometimes we may have to manipulate the equation to isolate the [latex]{x}^{2}[/latex] term so that the square root property can be used.A General Note: The Square Root Property
With the [latex]{x}^{2}[/latex] term isolated, the square root propty states that:[latex]\text{if }{x}^{2}=k,\text{then }x=\pm \sqrt{k}[/latex]
where k is a nonzero real number.
Recall: principal square root
Recall that the princicpal square root of a number such as [latex]\sqrt{9}[/latex] is the non-negative root, [latex]3[/latex]. And note that there is a difference between [latex]\sqrt{9}[/latex] and [latex]{x}^{2}=9[/latex]. In the case of [latex]{x}^{2}=9[/latex], we seek all numbers whose square is 9, that is [latex]x=\pm 3 [/latex].How To: Given a quadratic equation with an [latex]{x}^{2}[/latex] term but no [latex]x[/latex] term, use the square root property to solve it
- Isolate the [latex]{x}^{2}[/latex] term on one side of the equal sign.
- Take the square root of both sides of the equation, putting a [latex]\pm [/latex] sign before the expression on the side opposite the squared term.
- Simplify the numbers on the side with the [latex]\pm [/latex] sign.
Example: Solving a Simple Quadratic Equation Using the Square Root Property
Solve the quadratic using the square root property: [latex]{x}^{2}=8[/latex].Answer: Take the square root of both sides, and then simplify the radical. Remember to use a [latex]\pm [/latex] sign before the radical symbol.
[latex]\begin{array}{l}{x}^{2}\hfill&=8\hfill \\ x\hfill&=\pm \sqrt{8}\hfill \\ \hfill&=\pm 2\sqrt{2}\hfill \end{array}[/latex]
The solutions are [latex]x=2\sqrt{2}[/latex], [latex]x=-2\sqrt{2}[/latex].
Example: Solving a Quadratic Equation Using the Square Root Property
Solve the quadratic equation: [latex]4{x}^{2}+1=7[/latex]Answer: First, isolate the [latex]{x}^{2}[/latex] term. Then take the square root of both sides.
[latex]\begin{array}{l}4{x}^{2}+1=7\hfill \\ 4{x}^{2}=6\hfill \\ {x}^{2}=\frac{6}{4}\hfill \\ x=\pm \frac{\sqrt{6}}{2}\hfill \end{array}[/latex]
The solutions are [latex]x=\frac{\sqrt{6}}{2}[/latex], [latex]x=-\frac{\sqrt{6}}{2}[/latex].
Try It
Solve the quadratic equation using the square root property: [latex]3{\left(x - 4\right)}^{2}=15[/latex].Answer: [latex]x=4\pm \sqrt{5}[/latex]
[ohm_question]29172[/ohm_question]Using the Pythagorean Theorem
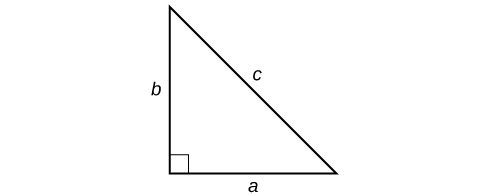
One of the most famous formulas in mathematics is the Pythagorean Theorem. It is based on a right triangle and states the relationship among the lengths of the sides as [latex]{a}^{2}+{b}^{2}={c}^{2}[/latex], where [latex]a[/latex] and [latex]b[/latex] refer to the legs of a right triangle adjacent to the [latex]90^\circ [/latex] angle, and [latex]c[/latex] refers to the hypotenuse. It has immeasurable uses in architecture, engineering, the sciences, geometry, trigonometry, and algebra, and in everyday applications. We use the Pythagorean Theorem to solve for the length of one side of a triangle when we have the lengths of the other two. Because each of the terms is squared in the theorem, when we are solving for a side of a triangle, we have a quadratic equation. We can use the methods for solving quadratic equations that we learned in this section to solve for the missing side. The Pythagorean Theorem is given as[latex]{a}^{2}+{b}^{2}={c}^{2}[/latex]
where [latex]a[/latex] and [latex]b[/latex] refer to the legs of a right triangle adjacent to the [latex]{90}^{\circ }[/latex] angle, and [latex]c[/latex] refers to the hypotenuse.
Example: Finding the Length of the Missing Side of a Right Triangle
Find the length of the missing side of the right triangle.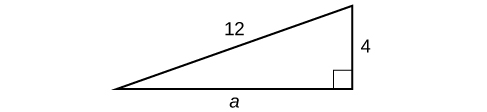
Answer: As we have measurements for side b and the hypotenuse, the missing side is a.
[latex]\begin{array}{l}{a}^{2}+{b}^{2}={c}^{2}\hfill \\ {a}^{2}+{\left(4\right)}^{2}={\left(12\right)}^{2}\hfill \\ {a}^{2}+16=144\hfill \\ {a}^{2}=128\hfill \\ a=\sqrt{128}\hfill \\ a=8\sqrt{2}\hfill \end{array}[/latex]
Try It
Use the Pythagorean Theorem to solve the right triangle problem: Leg a measures 4 units, leg b measures 3 units. Find the length of the hypotenuse.Answer: [latex]5[/latex] units
[ohm_question]48710[/ohm_question]Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
- Question ID 2029. Authored by: Lawrence Morales. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
- Question 29172. Authored by: Jim Smart. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
- Question ID 48710. Authored by: Darlene Diaz. License: CC BY: Attribution. License terms: IMathAS Community License CC- BY + GPL.
CC licensed content, Specific attribution
- College Algebra. Provided by: OpenStax Authored by: OpenStax College Algebra. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
