Methods for Solving a System of Nonlinear Equations
Learning Outcomes
- Solve a system that represents the intersection of a parabola and a line using substitution.
- Solve a system that represents the intersection of a circle and a line using substitution.
- Solve a system that represents the intersection of a circle and an ellipse using elimination.
tip for success
A quick sketch of the equations in a system can help you anticipate the possible number of solutions to the system, and can help you avoid missing solutions.Intersection of a Parabola and a Line
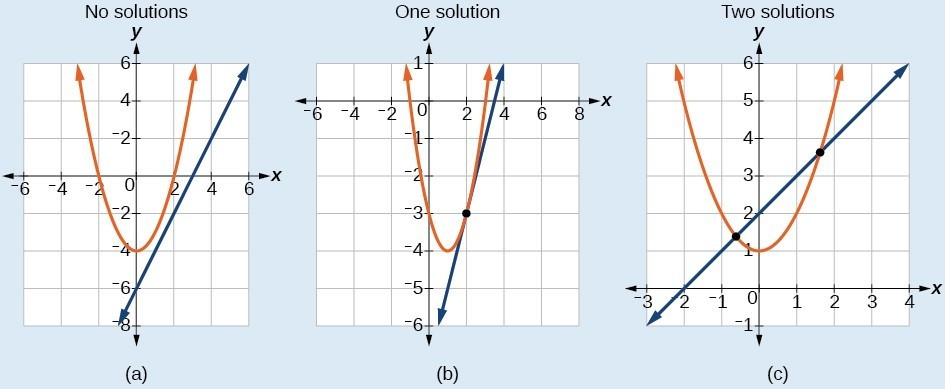
There are three possible types of solutions for a system of nonlinear equations involving a parabola and a line.A General Note: Possible Types of Solutions for Points of Intersection of a Parabola and a Line
The graphs below illustrate possible solution sets for a system of equations involving a parabola and a line.- No solution. The line will never intersect the parabola.
- One solution. The line is tangent to the parabola and intersects the parabola at exactly one point.
- Two solutions. The line crosses on the inside of the parabola and intersects the parabola at two points.
How To: Given a system of equations containing a line and a parabola, find the solution.
- Solve the linear equation for one of the variables.
- Substitute the expression obtained in step one into the parabola equation.
- Solve for the remaining variable.
- Check your solutions in both equations.
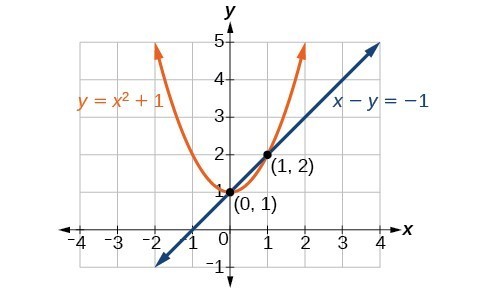
Example: Solving a System of Nonlinear Equations Representing a Parabola and a Line
Solve the system of equations.[latex]\begin{array}{l}x-y=-1\hfill \\ y={x}^{2}+1\hfill \end{array}[/latex]
Answer:
Solve the first equation for [latex]x[/latex] and then substitute the resulting expression into the second equation.
[latex]\begin{align}&x-y=-1 \\ &x=y - 1 && \text{Solve for }x. \\ \\ &y={x}^{2}+1 \\ &y={\left(y - 1\right)}^{2}+1 && \text{Substitute expression for }x. \\ &y=\left({y}^{2}-2y+1\right)+1 && \text{Expand} \\ &y={y}^{2}-2y+2 \\[3mm] &0={y}^{2}-3y+2 && \text{Set equal to 0 and solve.} \\ &0=\left(y - 2\right)\left(y - 1\right) \end{align}[/latex]
Solving for [latex]y[/latex] gives [latex]y=2[/latex] and [latex]y=1[/latex]. Next, substitute each value for [latex]y[/latex] into the first equation to solve for [latex]x[/latex]. Always substitute the value into the linear equation to check for extraneous solutions.
[latex]\begin{gathered}x-y=-1 \\ x-\left(2\right)=-1 \\ x=1 \\[3mm] x-\left(1\right)=-1 \\ x=0 \end{gathered}[/latex]
The solutions are [latex]\left(1,2\right)[/latex] and [latex]\left(0,1\right),\text{}[/latex] which can be verified by substituting these [latex]\left(x,y\right)[/latex] values into both of the original equations.
Q & A
Could we have substituted values for [latex]y[/latex] into the second equation to solve for [latex]x[/latex] in Example 1?
Yes, but because [latex]x[/latex] is squared in the second equation this could give us extraneous solutions for [latex]x[/latex]. For [latex]y=1[/latex][latex]\begin{align}&y={x}^{2}+1 \\ &y={x}^{2}+1 \\ &{x}^{2}=0 \\ &x=\pm \sqrt{0}=0 \end{align}[/latex]
This gives us the same value as in the solution.
For [latex]y=2[/latex]
[latex]\begin{align}&y={x}^{2}+1 \\ &2={x}^{2}+1 \\ &{x}^{2}=1 \\ &x=\pm \sqrt{1}=\pm 1 \end{align}[/latex]
Try It
Solve the given system of equations by substitution.[latex]\begin{gathered}3x-y=-2 \\ 2{x}^{2}-y=0 \end{gathered}[/latex]
Answer:
[latex-display]\left(-\dfrac{1}{2},\dfrac{1}{2}\right)[/latex] and [latex]\left(2,8\right)[/latex-display] [embed]Try it
Use an online graphing calculator to graph the parabola [latex]y\ =\ x^2+2x-3[/latex]. Now graph the line [latex]ax+by+c\ =\ 0[/latex] and adjust the values of [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] to find equations for lines that produce systems with the following types of solutions:- One solution
- Two solutions
- No solutions
Intersection of a Circle and a Line
Just as with a parabola and a line, there are three possible outcomes when solving a system of equations representing a circle and a line.A General Note: Possible Types of Solutions for the Points of Intersection of a Circle and a Line
The graph below illustrates possible solution sets for a system of equations involving a circle and a line.- No solution. The line does not intersect the circle.
- One solution. The line is tangent to the circle and intersects the circle at exactly one point.
- Two solutions. The line crosses the circle and intersects it at two points.

How To: Given a system of equations containing a line and a circle, find the solution.
- Solve the linear equation for one of the variables.
- Substitute the expression obtained in step one into the equation for the circle.
- Solve for the remaining variable.
- Check your solutions in both equations.
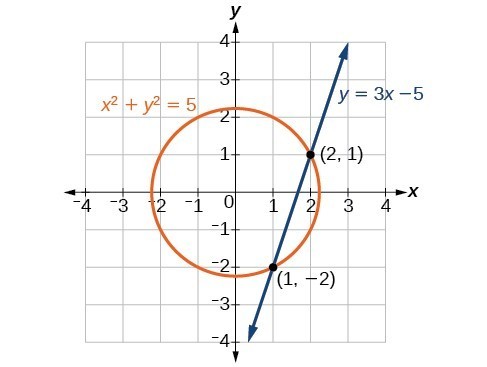
Example: Finding the Intersection of a Circle and a Line by Substitution
Find the intersection of the given circle and the given line by substitution.[latex]\begin{gathered}{x}^{2}+{y}^{2}=5 \\ y=3x - 5 \end{gathered}[/latex]
Answer:
One of the equations has already been solved for [latex]y[/latex]. We will substitute [latex]y=3x - 5[/latex] into the equation for the circle.[latex]\begin{gathered}{x}^{2}+{\left(3x - 5\right)}^{2}=5\\ {x}^{2}+9{x}^{2}-30x+25=5\\ 10{x}^{2}-30x+20=0\end{gathered}[/latex]
Now, we factor and solve for [latex]x[/latex].
[latex]\begin{gathered}10\left({x}^{2}-3x+2\right)=0 \\ 10\left(x - 2\right)\left(x - 1\right)=0 \\ x=2 \hspace{5mm} x=1 \end{gathered}[/latex]
Substitute the two x-values into the original linear equation to solve for [latex]y[/latex].
[latex]\begin{align}y&=3\left(2\right)-5 \\ &=1 \\[3mm] y&=3\left(1\right)-5 \\ &=-2 \end{align}[/latex]
The line intersects the circle at [latex]\left(2,1\right)[/latex] and [latex]\left(1,-2\right)[/latex], which can be verified by substituting these [latex]\left(x,y\right)[/latex] values into both of the original equations.

Try It
Solve the system of nonlinear equations.[latex]\begin{array}{l}{x}^{2}+{y}^{2}=10\hfill \\ x - 3y=-10\hfill \end{array}[/latex]
Answer: [latex]\left(-1,3\right)[/latex]
[embed]Solving a System of Nonlinear Equations Using Elimination
We have seen that substitution is often the preferred method when a system of equations includes a linear equation and a nonlinear equation. However, when both equations in the system have like variables of the second degree, solving them using elimination by addition is often easier than substitution. Generally, elimination is a far simpler method when the system involves only two equations in two variables (a two-by-two system), rather than a three-by-three system, as there are fewer steps. As an example, we will investigate the possible types of solutions when solving a system of equations representing a circle and an ellipse.tip for success
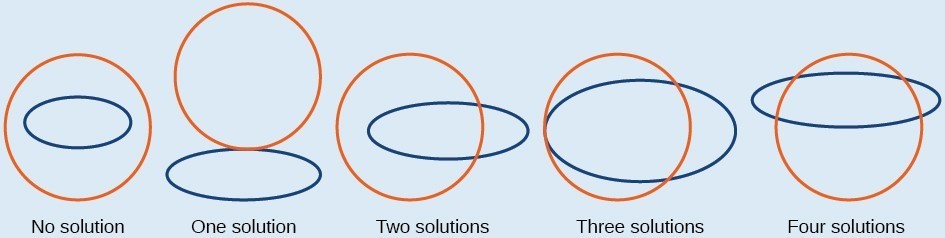
It is not necessary that you know the form of an equation given in a system in order to solve the system (such as with an ellipse or hyperbola in the examples below) . If you are unable to sketch the graph of an equation given in the system, you must be extra diligent with your algebra though to avoid missing or extraneous solutions.A General Note: Possible Types of Solutions for the Points of Intersection of a Circle and an Ellipse
The figure below illustrates possible solution sets for a system of equations involving a circle and an ellipse.- No solution. The circle and ellipse do not intersect. One shape is inside the other or the circle and the ellipse are a distance away from the other.
- One solution. The circle and ellipse are tangent to each other, and intersect at exactly one point.
- Two solutions. The circle and the ellipse intersect at two points.
- Three solutions. The circle and the ellipse intersect at three points.
- Four solutions. The circle and the ellipse intersect at four points.
Example: Solving a System of Nonlinear Equations Representing a Circle and an Ellipse
Solve the system of nonlinear equations.[latex]\begin{align} {x}^{2}+{y}^{2}=26 \hspace{5mm} \left(1\right)\\ 3{x}^{2}+25{y}^{2}=100 \hspace{5mm} \left(2\right)\end{align}[/latex]
Answer: Let’s begin by multiplying equation (1) by -3, and adding it to equation (2).
[latex]\left(-3\right)\left({x}^{2}+{y}^{2}\right)=\left(-3\right)\left(26\right)[/latex]
[latex]\begin{align}-3{x}^{2}-3{y}^{2}&=-78 \\ 3{x}^{2}+25{y}^{2}&=100 \\ \hline 22{y}^{2}&=22 \end{align}[/latex]
[latex]\begin{align}&{y}^{2}=1 \\ &y=\pm \sqrt{1}=\pm 1 \end{align}[/latex]
Substitute [latex]y=\pm 1[/latex] into one of the equations and solve for [latex]x[/latex].
[latex]\begin{align}&{x}^{2}+{\left(1\right)}^{2}=26 \\ &{x}^{2}+1=26 \\ &{x}^{2}=25 \\ &x=\pm \sqrt{25}=\pm 5 \\ \\ &{x}^{2}+{\left(-1\right)}^{2}=26 \\ &{x}^{2}+1=26 \\ &{x}^{2}=25=\pm 5 \end{align}[/latex]
There are four solutions:
[latex]\left(5,1\right),\left(-5,1\right),\left(5,-1\right),\text{and}\left(-5,-1\right)[/latex].
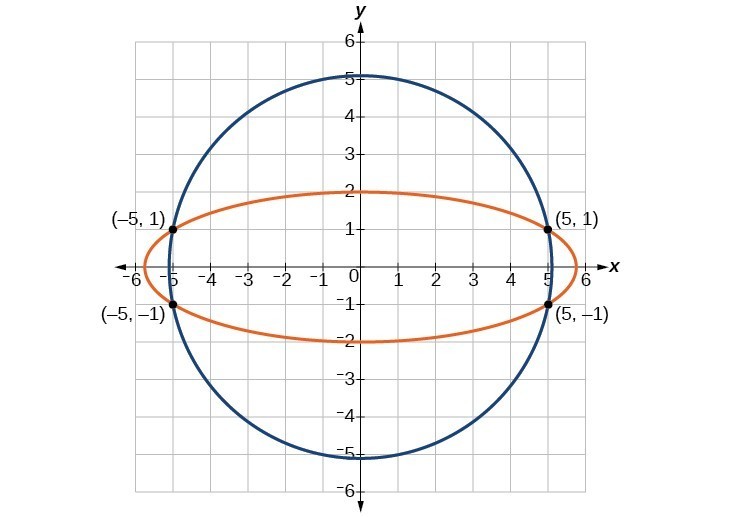
Try It
Use an online graphing calculator to find the solution to the system of equations.[latex]\begin{gathered}4{x}^{2}+{y}^{2}=13\\ {x}^{2}+{y}^{2}=10\end{gathered}[/latex]
Answer: [latex]\left\{\left(1,3\right),\left(1,-3\right),\left(-1,3\right),\left(-1,-3\right)\right\}[/latex]
Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
- Question ID 65041. Authored by: Etgen,Benjamin. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 62704. Authored by: Hidegkuti,Marta. License: CC BY: Attribution.
- Question ID 29703. Authored by: McClure, Caren. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Ex 3: Solve a System of Nonlinear Equations (Elimination) . Authored by: Sousa, James (Mathispower4u.com). License: CC BY: Attribution.
CC licensed content, Specific attribution
- Precalculus. Provided by: OpenStax Authored by: OpenStax College. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
