We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Mathematics for the Liberal Arts Corequisite

Dividing Decimals

Learning Outcomes

  • Divide a decimal by a whole number
  • Divide a decimal by another decimal
  • Divide a whole number by a decimal
Just as with multiplication, division of decimals is very much like dividing whole numbers. We just have to figure out where the decimal point must be placed. To understand decimal division, let’s consider the multiplication problem

[latex]\left(0.2\right)\left(4\right)=0.8[/latex]

Remember, a multiplication problem can be rephrased as a division problem. So we can write

[latex]0.8\div 4=0.2[/latex]

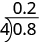We can think of this as "If we divide 8 tenths into four groups, how many are in each group?" The number line below shows that there are four groups of two-tenths in eight-tenths. So [latex]0.8\div 4=0.2[/latex]. A number line is shown with 0, 0.2, 0.4, 0.6, 0.8, and 1. There are braces showing a distance of 0.2 between each adjacent set of 2 numbers. Using long division notation, we would write A division problem is shown. 0.8 is on the inside of the division sign, 4 is on the outside. Above the division sign is 0.2. Notice that the decimal point in the quotient is directly above the decimal point in the dividend. To divide a decimal by a whole number, we place the decimal point in the quotient above the decimal point in the dividend and then divide as usual. Sometimes we need to use extra zeros at the end of the dividend to keep dividing until there is no remainder.

Divide a decimal by a whole number.

  1. Write as long division, placing the decimal point in the quotient above the decimal point in the dividend.
  2. Divide as usual.

example

Divide: [latex]0.12\div 3[/latex] Solution
[latex]0.12\div 3[/latex]
Write as long division, placing the decimal point in the quotient above the decimal point in the dividend. .
Divide as usual. Since [latex]3[/latex] does not go into [latex]0[/latex] or [latex]1[/latex] we use zeros as placeholders. .
[latex]0.12\div 3=0.04[/latex]
 

try it

[ohm_question]146600[/ohm_question]
Watch the following video to see another example of how to divide a decimal by a whole number. https://youtu.be/LkbSiL6uvtU

example

In everyday life, we divide whole numbers into decimals—money—to find the price of one item. For example, suppose a case of [latex]24[/latex] water bottles cost [latex]$3.99[/latex]. To find the price per water bottle, we would divide [latex]$3.99[/latex] by [latex]24[/latex], and round the answer to the nearest cent (hundredth). Divide: [latex]$3.99\div 24[/latex]

Answer: Solution

[latex]$3.99\div 24[/latex]
Place the decimal point in the quotient above the decimal point in the dividend. .
Divide as usual. When do we stop? Since this division involves money, we round it to the nearest cent (hundredth). To do this, we must carry the division to the thousandths place. .
Round to the nearest cent. [latex]$0.166\approx $0.17[/latex]
[latex]$3.99\div 24\approx $0.17[/latex]
This means the price per bottle is [latex]17[/latex] cents.

 

try it

[ohm_question]145993[/ohm_question]

Divide a Decimal by Another Decimal

So far, we have divided a decimal by a whole number. What happens when we divide a decimal by another decimal? Let’s look at the same multiplication problem we looked at earlier, but in a different way.

[latex]\left(0.2\right)\left(4\right)=0.8[/latex]

Remember, again, that a multiplication problem can be rephrased as a division problem. This time we ask, "How many times does [latex]0.2[/latex] go into [latex]0.8\text{?"}[/latex] Because [latex]\left(0.2\right)\left(4\right)=0.8[/latex], we can say that [latex]0.2[/latex] goes into [latex]0.8[/latex] four times. This means that [latex]0.8[/latex] divided by [latex]0.2[/latex] is [latex]4[/latex].

[latex]0.8\div 0.2=4[/latex]

A number line is shown with 0, 0.2, 0.4, 0.6, 0.8, and 1. There are braces showing a distance of 0.2 between each adjacent set of 2 numbers. We would get the same answer, [latex]4[/latex], if we divide [latex]8[/latex] by [latex]2[/latex], both whole numbers. Why is this so? Let’s think about the division problem as a fraction.

[latex]\begin{array}{c}{\Large\frac{0.8}{0.2}}\\ \\ {\Large\frac{\left(0.8\right)10}{\left(0.2\right)10}}\\ \\ {\Large\frac{8}{2}}\\ \\ 4\end{array}[/latex]

We multiplied the numerator and denominator by [latex]10[/latex] and ended up just dividing [latex]8[/latex] by [latex]4[/latex]. To divide decimals, we multiply both the numerator and denominator by the same power of [latex]10[/latex] to make the denominator a whole number. Because of the Equivalent Fractions Property, we haven’t changed the value of the fraction. The effect is to move the decimal points in the numerator and denominator the same number of places to the right. We use the rules for dividing positive and negative numbers with decimals, too. When dividing signed decimals, first determine the sign of the quotient and then divide as if the numbers were both positive. Finally, write the quotient with the appropriate sign. 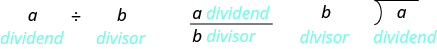It may help to review the vocabulary for division: a divided by b is shown with a labeled as the dividend and b labeled as the divisor. Then a over b is shown with a labeled as the divided and b labeled as the divisor. Then a is shown inside a division problem with b on the outside with a labeled as the dividend and b labeled as the divisor.

Divide decimal numbers

  1. Determine the sign of the quotient.
  2. Make the divisor a whole number by moving the decimal point all the way to the right. Move the decimal point in the dividend the same number of places to the right, writing zeros as needed.
  3. Divide. Place the decimal point in the quotient above the decimal point in the dividend.
  4. Write the quotient with the appropriate sign.
 

example

Divide: [latex]-2.89\div \text{(}3.4\text{)}[/latex]

Answer: Solution

Determine the sign of the quotient. The quotient will be negative.
Make the divisor the whole number by 'moving' the decimal point all the way to the right. 'Move' the decimal point in the dividend the same number of places to the right. .
Divide. Place the decimal point in the quotient above the decimal point in the dividend. Add zeros as needed until the remainder is zero. .
Write the quotient with the appropriate sign. [latex]-2.89\div \left(3.4\right)=-0.85[/latex]

 

try it

[ohm_question]146601[/ohm_question]
 

example

Divide: [latex]-25.65\div \text{(}-0.06\text{)}[/latex]

Answer: Solution

[latex]-25.65\div \left(-0.06\right)[/latex]
The signs are the same. The quotient is positive.
Make the divisor a whole number by 'moving' the decimal point all the way to the right. 'Move' the decimal point in the dividend the same number of places. .
Divide. Place the decimal point in the quotient above the decimal point in the dividend. .
Write the quotient with the appropriate sign. [latex]-25.65\div \left(-0.06\right)=427.5[/latex]

 

try it

[ohm_question]146604[/ohm_question]
In the next example we show how to divide two decimals that include negatives. https://youtu.be/o2ad4UzTlj0 Now we will divide a whole number by a decimal number.

example

Divide: [latex]4\div 0.05[/latex]

Answer: Solution

[latex]4\div 0.05[/latex]
The signs are the same. The quotient is positive.
Make the divisor a whole number by 'moving' the decimal point all the way to the right. Move the decimal point in the dividend the same number of places, adding zeros as needed. .
Divide. Place the decimal point in the quotient above the decimal point in the dividend. .
Write the quotient with the appropriate sign. [latex]4\div 0.05=80[/latex]
We can relate this example to money. How many nickels are there in four dollars? Because [latex]4\div 0.05=80[/latex], there are [latex]80[/latex] nickels in [latex]$4[/latex].

 

try it

[ohm_question]146607[/ohm_question]
The following example shows how to divide a whole number by a decimal using base ten blocks. https://youtu.be/LmWzhGvDt58

Licenses & Attributions

CC licensed content, Original

  • Question ID 146607, 146604, 146601,146600. Authored by: Lumen Learning. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.

CC licensed content, Shared previously

  • Ex: Dividing a Decimal by a Whole Number. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
  • Ex: Dividing Signed Decimals. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
  • Ex: Determine the Quotient of a Whole Number and Decimal using Base Ten Blocks. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.

CC licensed content, Specific attribution