Locating and Ordering Decimals With a Number Line
Learning Outcomes
- Locate decimals on a number line
- Order decimals using inequality notation
Since decimals are forms of fractions, locating decimals on the number line is similar to locating fractions on the number line.
Exercises
Locate
0.4 on a number line.
Solution
The decimal
0.4 is equivalent to
104, so
0.4 is located between
0 and
1. On a number line, divide the interval between
0 and
1 into
10 equal parts and place marks to separate the parts.
Label the marks
0.1,0.2,0.3,0.4,0.5,0.6,0.7,0.8,0.9,1.0. We write
0 as
0.0 and
1 as
1.0, so that the numbers are consistently in tenths. Finally, mark
0.4 on the number line.

try it
- [ohm_question]146228[/ohm_question]
- Locate 0.6 on a number line.
Answer:

example
Locate
−0.74 on a number line.
Answer:
Solution
The decimal −0.74 is equivalent to −10074, so it is located between 0 and −1. On a number line, mark off and label the multiples of −0.10 in the interval between 0 and −1 ( −0.10 , −0.20 , etc.) and mark −0.74 between −0.70 and −0.80, a little closer to −0.70 .

try it
[ohm_question]146577[/ohm_question]
[ohm_question]146578[/ohm_question]
In the next video we show more examples of how to locate a decimal on the number line.
https://youtu.be/F3LAKsOBdNA
Order Decimals
Which is larger, 0.04 or 0.40?
If you think of this as money, you know that $0.40 (forty cents) is greater than $0.04 (four cents). So,
0.40>0.04
In previous chapters, we used the number line to order numbers.
a<b , a is less than b when a is to the left of b on the number linea>b , a is greater than b when a is to the right of b on the number line
Where are 0.04 and 0.40 located on the number line?
 We see that 0.40 is to the right of 0.04. So we know 0.40>0.04.
How does 0.31 compare to 0.308? This doesn’t translate into money to make the comparison easy. But if we convert 0.31 and 0.308 to fractions, we can tell which is larger.
We see that 0.40 is to the right of 0.04. So we know 0.40>0.04.
How does 0.31 compare to 0.308? This doesn’t translate into money to make the comparison easy. But if we convert 0.31 and 0.308 to fractions, we can tell which is larger.
|
0.31 |
0.308 |
| Convert to fractions. |
10031 |
1000308 |
| We need a common denominator to compare them. |
100⋅1031⋅10 |
1000308 |
|
1000310 |
1000308 |
Because 310>308, we know that 1000310>1000308. Therefore, 0.31>0.308.
Notice what we did in converting 0.31 to a fraction—we started with the fraction 10031 and ended with the equivalent fraction 1000310. Converting 1000310 back to a decimal gives 0.310. So 0.31 is equivalent to 0.310. Writing zeros at the end of a decimal does not change its value.
10031=1000310 and 0.31=0.310
If two decimals have the same value, they are said to be equivalent decimals.
0.31=0.310
We say 0.31 and 0.310 are equivalent decimals.
Equivalent Decimals
Two decimals are equivalent decimals if they convert to equivalent fractions.
Remember, writing zeros at the end of a decimal does not change its value.
Order decimals
- Check to see if both numbers have the same number of decimal places. If not, write zeros at the end of the one with fewer digits to make them match.
- Compare the numbers to the right of the decimal point as if they were whole numbers.
- Order the numbers using the appropriate inequality sign.
example
Order the following decimals using
< or >:
- 0.64 ____ 0.6
- 0.83 ____ 0.803
Answer:
Solution
| 1. |
|
|
0.64 ____ 0.6 |
| Check to see if both numbers have the same number of decimal places. They do not, so write one zero at the right of 0.6. |
0.64 ____ 0.6 |
| Compare the numbers to the right of the decimal point as if they were whole numbers. |
64>60 |
| Order the numbers using the appropriate inequality sign. |
0.64>0.60
0.64>0.6 |
| 2. |
|
|
0.83 ____ 0.803 |
| Check to see if both numbers have the same number of decimal places. They do not, so write one zero at the right of 0.83. |
0.83 ____ 0.803 |
| Compare the numbers to the right of the decimal point as if they were whole numbers. |
830>803 |
| Order the numbers using the appropriate inequality sign. |
0.830>0.803
0.83>0.803 |
try it
[ohm_question]146232[/ohm_question]
[ohm_question]146237[/ohm_question]
[ohm_question]146238[/ohm_question]
When we order negative decimals, it is important to remember how to order negative integers. Recall that larger numbers are to the right on the number line. For example, because −2 lies to the right of −3 on the number line, we know that −2>−3. Similarly, smaller numbers lie to the left on the number line. For example, because −9 lies to the left of −6 on the number line, we know that −9<−6.
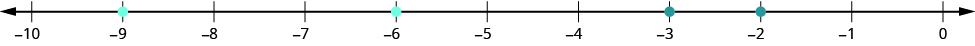 If we zoomed in on the interval between 0 and −1, we would see in the same way that −0.2>−0.3and−0.9<−0.6.
If we zoomed in on the interval between 0 and −1, we would see in the same way that −0.2>−0.3and−0.9<−0.6.
example
Use
<or>; to order.
−0.1 ____
−0.8.
Answer:
Solution:
|
−0.1 ____ −0.8 |
| Write the numbers one under the other, lining up the decimal points. |
−0.1
−0.8 |
| They have the same number of digits. |
|
| Since −1>−8,−1 tenth is greater than −8 tenths. |
−0.1>−0.8 |
try it
[ohm_question]146239[/ohm_question]
In the following video lesson we show how to order decimals using inequality notation by comparing place values, and by using fractions.
https://youtu.be/fjO3fnt3ABALicenses & Attributions
CC licensed content, Original
- Question ID 146237, 146238, 146239. Authored by: Lumen Learning. License: CC BY: Attribution.
CC licensed content, Shared previously
- Example: Identify Decimals on the Number Line. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
- Decimal Notation: Ordering Decimals. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
CC licensed content, Specific attribution
- Prealgebra. Provided by: OpenStax License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/caa57dab-41c7-455e-bd6f-f443cda5519c@9.757.



 We see that is to the right of . So we know .
How does compare to This doesn’t translate into money to make the comparison easy. But if we convert and to fractions, we can tell which is larger.
We see that is to the right of . So we know .
How does compare to This doesn’t translate into money to make the comparison easy. But if we convert and to fractions, we can tell which is larger.
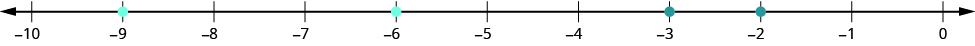 If we zoomed in on the interval between and , we would see in the same way that .
If we zoomed in on the interval between and , we would see in the same way that .
