Exponential Functions
Learning Objectives
- Define and Evaluate Exponential Functions
- Define an exponential function and it's domain and range
- Evaluate an exponential function
- Define and evaluate a compound interest formula
- Exponential Functions with Base e
- Define the number e
- Define continuous growth as an exponential function with base e
- Evaluate exponential functions with base e
- Graph Exponential Functions
- Generate a table of values for an exponential function
- Plot an exponential function on Cartesian axes
| Year | Stores, Company A | Description of Growth | Stores, Company B |
| 0 | 100 | Starting with 100 each | 100 |
| 1 | 100+50=150 | They both grow by 50 stores in the first year. | 100 + 50% of 100 100 + 0.50(100) = 150 |
| 2 | 150+50=200 | Store A grows by 50, Store B grows by 75 | 150 + 50% of 150 150 + 0.50(150) = 225 |
| 3 | 200+50=250 | Store A grows by 50, Store B grows by 112.5 | 225 + 50% of 225 225 + 0.50(225) = 337.5 |
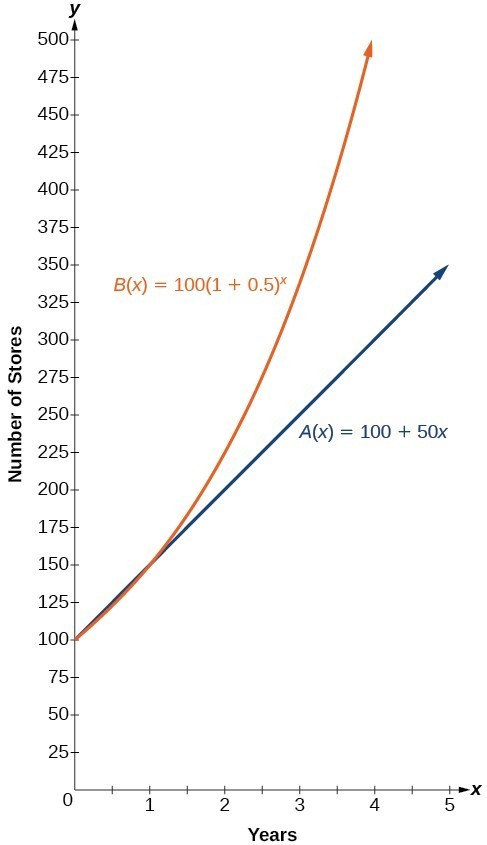 The graph shows the numbers of stores Companies A and B opened over a five-year period.
The graph shows the numbers of stores Companies A and B opened over a five-year period.Notice that the domain for both functions is [latex]\left[0,\infty \right)[/latex], and the range for both functions is [latex]\left[100,\infty \right)[/latex]. After year 1, Company B always has more stores than Company A.
Consider the function representing the number of stores for Company B
[latex]B\left(x\right)=100{\left(1+0.5\right)}^{x}[/latex]
In this exponential function, 100 represents the initial number of stores, 0.50 represents the growth rate, and [latex]1+0.5=1.5[/latex] represents the growth factor. Generalizing further, we can write this function as [latex]B\left(x\right)=100{\left(1.5\right)}^{x}[/latex], where 100 is the initial value, 1.5 is called the base, and x is called the exponent. This is an exponential function.Exponential Growth
A function that models exponential growth grows by a rate proportional to the current amount. For any real number x and any positive real numbers a and b such that [latex]b\ne 1[/latex], an exponential growth function has the form
where
- a is the initial or starting value of the function.
- b is the growth factor or growth multiplier per unit x.
To evaluate an exponential function with the form [latex]f\left(x\right)={b}^{x}[/latex], we simply substitute x with the given value, and calculate the resulting power. For example:
Let [latex]f\left(x\right)={2}^{x}[/latex]. What is [latex]f\left(3\right)[/latex]?
To evaluate an exponential function with a form other than the basic form, it is important to follow the order of operations. For example:
Let [latex]f\left(x\right)=30{\left(2\right)}^{x}[/latex]. What is [latex]f\left(3\right)[/latex]?
Note that if the order of operations were not followed, the result would be incorrect:
Example
Let [latex]f\left(x\right)=5{\left(3\right)}^{x+1}[/latex]. Evaluate [latex]f\left(2\right)[/latex] without using a calculator.Answer:
Follow the order of operations. Be sure to pay attention to the parentheses.
Example
At the beginning of this section, we learned that the population of India was about 1.25 billion in the year 2013, with an annual growth rate of about 1.2%. This situation is represented by the growth function [latex]P\left(t\right)=1.25{\left(1.012\right)}^{t}[/latex], where t is the number of years since 2013. To the nearest thousandth, what will the population of India be in 2031?Answer:
To estimate the population in 2031, we evaluate the models for t = 18, because 2031 is 18 years after 2013. Rounding to the nearest thousandth,
There will be about 1.549 billion people in India in the year 2031.
The annual percentage rate (APR) of an account, also called the nominal rate, is the yearly interest rate earned by an investment account. The term nominal is used when the compounding occurs a number of times other than once per year. In fact, when interest is compounded more than once a year, the effective interest rate ends up being greater than the nominal rate! This is a powerful tool for investing.
We can calculate the compound interest using the compound interest formula, which is an exponential function of the variables time t, principal P, APR r, and number of compounding periods in a year n:
The Compound Interest Formula
Compound interest can be calculated using the formula
where
- A(t) is the account value,
- t is measured in years,
- P is the starting amount of the account, often called the principal, or more generally present value,
- r is the annual percentage rate (APR) expressed as a decimal, and
- n is the number of compounding periods in one year.
Example
If we invest $3,000 in an investment account paying 3% interest compounded quarterly, how much will the account be worth in 10 years?Answer:
Because we are starting with $3,000, P = 3000. Our interest rate is 3%, so r = 0.03. Because we are compounding quarterly, we are compounding 4 times per year, so n = 4. We want to know the value of the account in 10 years, so we are looking for A(10), the value when t = 10.
The account will be worth about $4,045.05 in 10 years.
Example
A 529 Plan is a college-savings plan that allows relatives to invest money to pay for a child’s future college tuition; the account grows tax-free. Lily wants to set up a 529 account for her new granddaughter and wants the account to grow to $40,000 over 18 years. She believes the account will earn 6% compounded semi-annually (twice a year). To the nearest dollar, how much will Lily need to invest in the account now?Answer:
The nominal interest rate is 6%, so r = 0.06. Interest is compounded twice a year, so k = 2.
We want to find the initial investment, P, needed so that the value of the account will be worth $40,000 in 18 years. Substitute the given values into the compound interest formula, and solve for P.
Lily will need to invest $13,801 to have $40,000 in 18 years.
Exponential functions with base e
As we saw earlier, the amount earned on an account increases as the compounding frequency increases. The table below shows that the increase from annual to semi-annual compounding is larger than the increase from monthly to daily compounding. This might lead us to ask whether this pattern will continue.Examine the value of $1 invested at 100% interest for 1 year, compounded at various frequencies.
| Frequency | [latex]A\left(t\right)={\left(1+\frac{1}{n}\right)}^{n}[/latex] | Value |
|---|---|---|
| Annually | [latex]{\left(1+\frac{1}{1}\right)}^{1}[/latex] | $2 |
| Semiannually | [latex]{\left(1+\frac{1}{2}\right)}^{2}[/latex] | $2.25 |
| Quarterly | [latex]{\left(1+\frac{1}{4}\right)}^{4}[/latex] | $2.441406 |
| Monthly | [latex]{\left(1+\frac{1}{12}\right)}^{12}[/latex] | $2.613035 |
| Daily | [latex]{\left(1+\frac{1}{365}\right)}^{365}[/latex] | $2.714567 |
| Hourly | [latex]{\left(1+\frac{1}{\text{8766}}\right)}^{\text{8766}}[/latex] | $2.718127 |
| Once per minute | [latex]{\left(1+\frac{1}{\text{525960}}\right)}^{\text{525960}}[/latex] | $2.718279 |
| Once per second | [latex]{\left(1+\frac{1}{31557600}\right)}^{31557600}[/latex] | $2.718282 |
These values appear to be approaching a limit as n increases. In fact, as n gets larger and larger, the expression [latex]{\left(1+\frac{1}{n}\right)}^{n}[/latex] approaches a number used so frequently in mathematics that it has its own name: the letter [latex]e[/latex]. This value is an irrational number, which means that its decimal expansion goes on forever without repeating. Its approximation to six decimal places is shown below.
A General Note: The Number e
The letter e represents the irrational number
The letter e is used as a base for many real-world exponential models. To work with base e, we use the approximation, [latex]e\approx 2.718282[/latex]. The constant was named by the Swiss mathematician Leonhard Euler (1707–1783) who first investigated and discovered many of its properties.
Example
Calculate [latex]{e}^{3.14}[/latex]. Round to five decimal places.Answer: On a calculator, press the button labeled [latex]\left[{e}^{x}\right][/latex]. The window shows [e^(]. Type 3.14 and then close parenthesis, (]). Press [ENTER]. Rounding to 5 decimal places, [latex]{e}^{3.14}\approx 23.10387[/latex]. Caution: Many scientific calculators have an "Exp" button, which is used to enter numbers in scientific notation. It is not used to find powers of e.
Investigating Continuous Growth
So far we have worked with rational bases for exponential functions. For most real-world phenomena, however, e is used as the base for exponential functions. Exponential models that use e as the base are called continuous growth or decay models. We see these models in finance, computer science, and most of the sciences, such as physics, toxicology, and fluid dynamics.
The Continuous Growth/Decay Formula
For all real numbers r, t, and all positive numbers a, continuous growth or decay is represented by the formula
where
- a is the initial value,
- r is the continuous growth or decay rate per unit time,
- and t is the elapsed time.
If r > 0, then the formula represents continuous growth. If r < 0, then the formula represents continuous decay.
For business applications, the continuous growth formula is called the continuous compounding formula and takes the form
where
- P is the principal or the initial invested,
- r is the growth or interest rate per unit time,
- and t is the period or term of the investment.
Example
A person invested $1,000 in an account earning a nominal 10% per year compounded continuously. How much was in the account at the end of one year?Answer: Since the account is growing in value, this is a continuous compounding problem with growth rate r = 0.10. The initial investment was $1,000, so P = 1000. We use the continuous compounding formula to find the value after t = 1 year:
The account is worth $1,105.17 after one year.
How To: Given the initial value, rate of growth or decay, and time t, solve a continuous growth or decay function.
- Use the information in the problem to determine a, the initial value of the function.
- Use the information in the problem to determine the growth rate r.
- If the problem refers to continuous growth, then r > 0.
- If the problem refers to continuous decay, then r < 0.
- Use the information in the problem to determine the time t.
- Substitute the given information into the continuous growth formula and solve for A(t).
Example
Radon-222 decays at a continuous rate of 17.3% per day. How much will 100 mg of Radon-222 decay to in 3 days?Answer: Since the substance is decaying, the rate, 17.3%, is negative. So, r = –0.173. The initial amount of radon-222 was 100 mg, so a = 100. We use the continuous decay formula to find the value after t = 3 days:
So 59.5115 mg of radon-222 will remain.
Graph Exponential Functions
We learn a lot about things by seeing their pictorial representations, and that is exactly why graphing exponential equations is a powerful tool. It gives us another layer of insight for predicting future events. Before we begin graphing, it is helpful to review the behavior of exponential growth. Recall the table of values for a function of the form [latex]f\left(x\right)={b}^{x}[/latex] whose base is greater than one. We’ll use the function [latex]f\left(x\right)={2}^{x}[/latex]. Observe how the output values in the table below change as the input increases by 1.
| x | –3 | –2 | –1 | 0 | 1 | 2 | 3 |
| [latex]f\left(x\right)={2}^{x}[/latex] | [latex]\frac{1}{8}[/latex] | [latex]\frac{1}{4}[/latex] | [latex]\frac{1}{2}[/latex] | 1 | 2 | 4 | 8 |
Each output value is the product of the previous output and the base, 2. We call the base 2 the constant ratio. In fact, for any exponential function with the form [latex]f\left(x\right)=a{b}^{x}[/latex], b is the constant ratio of the function. This means that as the input increases by 1, the output value will be the product of the base and the previous output, regardless of the value of a.
Notice from the table that
- the output values are positive for all values of x;
- as x increases, the output values increase without bound; and
- as x decreases, the output values grow smaller, approaching zero.
 Notice that the graph gets close to the x-axis, but never touches it.
Notice that the graph gets close to the x-axis, but never touches it.The domain of [latex]f\left(x\right)={2}^{x}[/latex] is all real numbers, the range is [latex]\left(0,\infty \right)[/latex].
To get a sense of the behavior of exponential decay, we can create a table of values for a function of the form [latex]f\left(x\right)={b}^{x}[/latex] whose base is between zero and one. We’ll use the function [latex]g\left(x\right)={\left(\frac{1}{2}\right)}^{x}[/latex]. Observe how the output values in the table below change as the input increases by 1.
| x | –3 | –2 | –1 | 0 | 1 | 2 | 3 |
| [latex]g\left(x\right)=\left(\frac{1}{2}\right)^{x}[/latex] | 8 | 4 | 2 | 1 | [latex]\frac{1}{2}[/latex] | [latex]\frac{1}{4}[/latex] | [latex]\frac{1}{8}[/latex] |
Again, because the input is increasing by 1, each output value is the product of the previous output and the base, or constant ratio [latex]\frac{1}{2}[/latex].
Notice from the table that
- the output values are positive for all values of x;
- as x increases, the output values grow smaller, approaching zero; and
- as x decreases, the output values grow without bound.
The graph shows the exponential decay function, [latex]g\left(x\right)={\left(\frac{1}{2}\right)}^{x}[/latex].
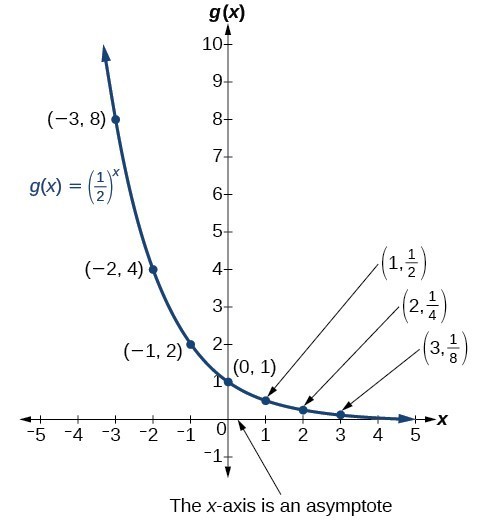
The domain of [latex]g\left(x\right)={\left(\frac{1}{2}\right)}^{x}[/latex] is all real numbers, the range is [latex]\left(0,\infty \right)[/latex]
Characteristics of the Graph of f(x) = bx
An exponential function with the form [latex]f\left(x\right)={b}^{x}[/latex], [latex]b>0[/latex], [latex]b\ne 1[/latex], has these characteristics:
- one-to-one function
- domain: [latex]\left(-\infty , \infty \right)[/latex]
- range: [latex]\left(0,\infty \right)[/latex]
- x-intercept: none
- y-intercept: [latex]\left(0,1\right)[/latex]
- increasing if [latex]b>1[/latex]
- decreasing if [latex]b<1[/latex]
Compare the graphs of exponential growth and decay functions.

Example
Sketch a graph of [latex]f\left(x\right)={0.25}^{x}[/latex]. State the domain, range.Answer:
Before graphing, identify the behavior and create a table of points for the graph.
- Since b = 0.25 is between zero and one, we know the function is decreasing, and we can verify this by creating a table of values. The left tail of the graph will increase without bound and the right tail will get really close to the x-axis.
- Create a table of points.
x –3 –2 –1 0 1 2 3 [latex]f\left(x\right)={0.25}^{x}[/latex] 64 16 4 1 0.25 0.0625 0.015625 - Plot the y-intercept, [latex]\left(0,1\right)[/latex], along with two other points. We can use [latex]\left(-1,4\right)[/latex] and [latex]\left(1,0.25\right)[/latex].
Draw a smooth curve connecting the points.

The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(0,\infty \right)[/latex].
How To: Given an exponential function of the form [latex]f\left(x\right)={b}^{x}[/latex], graph the function.
- Create a table of points.
- Plot at least 3 point from the table, including the y-intercept [latex]\left(0,1\right)[/latex].
- Draw a smooth curve through the points.
- State the domain, [latex]\left(-\infty ,\infty \right)[/latex], the range, [latex]\left(0,\infty \right)[/latex].
Example
Sketch a graph of [latex]f(x)={\sqrt{2}(\sqrt{2})}^{x}[/latex]. State the domain, range.Answer:
Before graphing, identify the behavior and create a table of points for the graph.
- Since b = [latex]\sqrt{2}[/latex] which is greater than one, we know the function is increasing, and we can verify this by creating a table of values. The left tail of the graph will get really close to the x-axis and the right tail will increase without bound.
- Create a table of points.
x –3 –2 –1 0 1 2 3 [latex]f\left(x\right)=\sqrt{2}{(\sqrt{2})}^{x}[/latex] 0.5 0.71 1 1.41 2 2.83 4 - Plot the y-intercept, [latex]\left(0,1.41\right)[/latex], along with two other points. We can use [latex]\left(-1,1\right)[/latex] and [latex]\left(1,2\right)[/latex].
Draw a smooth curve connecting the points.
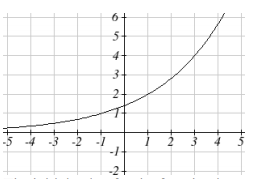 The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(0,\infty \right)[/latex].
The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(0,\infty \right)[/latex].
Summary
Licenses & Attributions
CC licensed content, Original
- Determine Exponential Function Values and Graph the Function. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Evaluate a Given Exponential Function to Predict a Future Population. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
- Determine a Continuous Exponential Decay Function and Make a Prediction. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Graph a Basic Exponential Function Using a Table of Values. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Graph an Exponential Function Using a Table of Values. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
CC licensed content, Shared previously
- Ex 1: Compounded Interest Formula - Quarterly. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: Compounded Interest Formula - Determine Deposit Needed (Present Value). Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay, et al.. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution. License terms: Download for free at : http://cnx.org/contents/[email protected]:1/Preface.
- Ex 1: Continuous Interest Formula. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
