Polynomial Equations
Learning Objectives
- Polynomial Equations
- Use factoring methods to factor polynomial equations
- Use the principle of zero products to solve polynomial equations
- Applications of Polynomial Equations
- Projectiles
- Use the Pythagorean theorem to find the lengths of a right triangle
- Geometric Applications
- Cost, Revenue, and Profit Polynomials
Not all of the techniques we use for solving linear equations will apply to solving polynomial equations. In this section we will introduce a method for solving polynomial equations that combines factoring and the zero product principle.
The Principle of Zero Products
Zero
Principle of Zero Products
The Principle of Zero Products states that if the product of two numbers is 0, then at least one of the factors is 0. If
ab=0, then either
a=0 or
b=0, or both
a and
b are 0.
Let's start with a simple example. We will factor a GCF from a binomial and apply the principle of zero products to solve a polynomial equation.
Example
Solve:
−t2+t=0
Answer:
Each term has a common factor of t, so we can factor and use the zero product principle. Rewrite each term as the product of the GCF and the remaining terms.
−t2=t(−t)t=t(1)
Rewrite the polynomial equation using the factored terms in place of the original terms.
−t2+t=0t(−t)+t(1)t(−t+1)=0
Now we have a product on one side and zero on the other, so we can set each factor equal to zero using the zero product principle.
t=0 OR −t+1=0−1−1−t=−1−1−t=−1−1t=1
Answer
t=0 OR t=1
In the following video we show two more examples of using both factoring and the principle of zero products to solve a polynomial equation.
https://youtu.be/gIwMkTAclw8
In the next video we show that you can factor a trinomial using methods previously learned to solve a quadratic equation.
https://youtu.be/bi7i_RuIGl0
You and I both know that it is rare to be given an equation to solve that has zero on one side, so let's try another one.
Example
Solve:
s2−4s=5
Answer:
First, move all the terms to one side. The goal is to try and see if we can use the zero product principle, since that is the only tool we know for solving polynomial equations.
s2−4s=5s2−4s−5=0
We now have all the terms on the left side, and zero on the other side. The polynomials2−4s−5 factors nicely, which makes this equation a good candidate for the zero product principle. (imagine that)
s2−4s−5=0(s+1)(s−5)=0
We separate our factors into two linear equations using the principle of zero products.
(s−5)=0s−5=0s=5
OR
(s+1)=0s+1=0s=−1
Answer
s=−1 OR s=5
We will work through one more example that is similar to the one above, except this example has fractions, yay!
Example
Solve
y2−5=−27y+25
Answer: We can solve this in one of two ways. One way is to eliminate the fractions like you may have done when solving linear equations, and the second is to find a common denominator and factor fractions. Eliminating fractions is easier, so we will show that way.
Start by multiplying the whole equation by 2 to eliminate fractions:
2(y2−5=−27y+25)2(y2)+2(−5)=2(−27)+2(−25)2y2−10=−7y+5
Now we can move all the terms to one side and see if this will factor so we can use the principle of zero products.
2y2−10=−7y−52y2−10+7y−5=02y2−15+7y=02y2+7y−15=0
We can now check whether this polynomial will factor, using a table we can list factors until we find two numbers with a product of 2⋅−15=−30 and a sum of 7.
| Factors of 2⋅−15=−30 |
Sum of Factors |
| 1,−30 |
−29 |
| −1,30 |
29 |
| 2,−15 |
−13 |
| −2,15 |
13 |
| 3,−10 |
−7 |
| −3,10 |
7 |
We have found the factors that will produce the middle term we want,
−3,10. We need to place the factors in a way that will lead to a term of
10y:
(2y−3)(y+5)=0
Now we can set each factor equal to zero and solve:
(2y−3)=0 OR (y+5)=02y=3 OR y=−5y=23 OR y=−5
You can always check your work to make sure your solutions are correct:
Check
y=23
(23)2−5=−27(23)+2549−5=−421+25 common denominator = 449−420=−421+410−411=−411
y=23[/latex]isindeedasolution,nowcheck [latex]y=−5
(−5)2−5=−27(−5)+2525−5=235+2520=24020=20
y=−5 is also a solution, so we must have done something right!
Answer
y=23 OR y=−5
In our last video, we show how to solve another quadratic equation that contains fractions.
https://youtu.be/kDj_qdKW-ls
Projectile Motion
Projectile motion happens when you throw a ball into the air and it comes back down because of gravity. A projectile will follow a curved path that behaves in a predictable way. This predictable motion has been studied for centuries, and in simple cases it's height from the ground at a given time, t, can be modeled with a polynomial function of the form h(t)=at2+bt+c, where h(t) = height of an object at a given time, t. Projectile motion is also called a parabolic trajectory because of the shape of the path of a projectile's motion, as in the image of water in the fountain below.

Parabolic WaterTrajectory
Example
A small toy rocket is launched from a 4-foot pedestal. The height (
h, in feet) of the rocket
t seconds after taking off is given by the function
h(t)=−2t2+7t+4. How long will it take the rocket to hit the ground?
Answer:
The rocket will be on the ground when the h(t)=0. We want to know how long, t, the rocket is in the air.
h(t)=−2t2+7t+4=00=−2t2+7t+4
We can factor the polynomial −2t2+7t+4 more easily by first factoring out a −1
0=−1(2t2−7t−4)0=−1(2t+1)(t−4)
Use the Zero Product Property. There is no need to set the constant factor −1 to zero, because −1 will never equal zero.
2t+1=0ort−4=0
Solve each equation.
t=−21ort=4
Interpret the answer. Since
t represents time, it cannot be a negative number; only
t=4 makes sense in this context.
t=4
We can check our answer:
h(4)=−2(4)2+7(4)+4=0h(4)=−2(16)+28+4=0h(4)−32+32=0h(4)=0
Answer
The rocket will hit the ground 4 seconds after being launched.
In the next example we will solve for the time that the rocket is at a given height other than zero.
Example
Use the formula for the height of the rocket in the previous example to find the time when the rocket is 4 feet from hitting the ground on it's way back down. Refer to the image.
h(t)=−2t2+7t+4
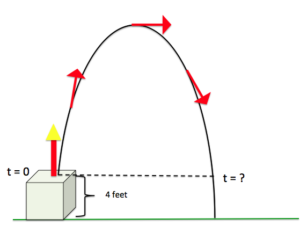
Answer:
We are given that the height of the rocket is 4 feet from the ground on it's way back down. We want to know how long it has taken the rocket to get to that point in it's path, we are going to solve for t.
Substitute h(t)=4 into the formula for height, and try to get zero on one side since we know we can use the zero product principle to solve polynomials.
Write and Solve:
h(t)=4=−2t2+7t+44=−2t2+7t+4−4−40=−2t2+7t
Now we can factor out a t from each term:
0=t(−2t+7)
Solve each equation for t using the zero product principle:
t=0 OR −2t+7=0−7−7−2−2t=−2−7t=27=3.5
It doesn't make sense for us to choose t=0 because we are interested in the amount of time that has passed when the projectile is 4 feet from hitting the ground on it's way back down. We will choose t=3.5
Check the answer on your own for practice.
Answer
t=3.5 seconds
In the following video we show another example of how to find the time when a object following a parabolic trajectory hits the ground.
https://youtu.be/hsWSzu3KcPU
In this section we introduced the concept of projectile motion, and showed that it can be modeled with polynomial function. While the models used in these examples are simple, the concepts and interpretations are the same as what would happen in "real life".
Pythagorean Theorem
The Pythagorean theorem or Pythagoras's theorem is a statement about the sides of a right triangle. One of the angles of a right triangle is always equal to 90 degrees. This angle is the right angle. The two sides next to the right angle are called the legs and the other side is called the hypotenuse. The hypotenuse is the side opposite to the right angle, and it is always the longest side. The image above shows four common kinds of triangle, including a right triangle.
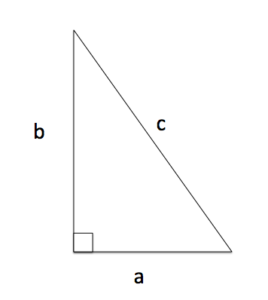
Right Triangle Labeled
The Pythagorean theorem is often used to find unknown lengths of the sides of right triangles. If the longest leg of a right triangle is labeled c, and the other two a, and b as in the image on teh left, The Pythagorean Theorem states that
a2+b2=c2
Given enough information, we can solve for an unknown length. This relationship has been used for many, many years for things such as celestial navigation and early civil engineering projects. We now have digital GPS and survey equipment that have been programmed to do the calculations for us.
In the next example we will combine the power of the Pythagorean theorem and what we know about solving quadratic equations to find unknown lengths of right triangles.
Example
A right triangle has one leg with length x, another whose length is greater by two, and the length of the hypotenuse is greater by four. Find the lengths of the sides of the triangle. Use the image below.
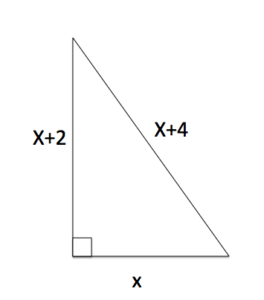
Answer:
Read and understand: We know the lengths of all the sides of a triangle in terms of one side. We also know that the Pythagorean theorem will give us a relationship between the side lengths of a right triangle.
Translate:
a2+b2=c2x2+(x+2)2=(x+4)2
Solve: If we can move all the terms to one side and factor, we can use the zero product principle to solve. Since this is the only method we know - let's hope it works!
First, multiply the binomials and simplify so we can see what we are working with.
x2+(x+2)2=(x+4)2x2+x2+4x+4=x2+8x+162x2+4x+4=x2+8x+16
Now move all the terms to one side and see if we can factor.
2x2+4x+4=x2+8x+16−x2−8x−16−x2−8x−16x2−4x−12=0
This went from a messy looking problem to something promising. We can factor using the shortcut:
−6⋅2=−12, and −6+2=−4
So we can build our binomial factors with -6 and 2:
(x−6)(x+2)=0
Set each factor equal to zero:
x−6=0,x=6
x+2=0,x=−2
Interpret: Ok, it doesn't make sense to have a length equal to -2, so we can safely throw that solution out. The lengths of the sides are as follows:
x=6
x+2=6+2=8
x+4=6+4=10
Check: Since we know the relationship between the sides of a right triangle we can check that we are correct. Sometimes it helps to draw a picture
.
We know that a2+b2=c2, so we can substitute the values we found:
62+82=10236+64=100100=100
Our solution checks out.
Answer
The lengths of the sides of the right triangle are 6, 8, and 10
This video example shows another way a quadratic equation can be used to find and unknown length of a right triangle.
https://youtu.be/xeP5pRBqsNs
Geometric Applications
In this section we will explore ways that polynomials are used in applications of area problems.
Example
The length of a rectangle is 3 more than the width. If the area is 40 square inches, what are the dimensions?
Answer:
\begin{eqnarray*}
x & & \text{ We} \text{ do} \text{ not} \text{ know} \text{ the}
\text{ width}, x.\\
x + 3 & & \text{ Length} \text{ is}\, 4 \text{ more}, \text{ or}\, x + 4,
\text{ and} \text{ area} \text{ is}\, 40.\\
x (x + 3) = 40 & & \text{ Multiply} \text{ length} \text{ by} \text{ width}
\text{ to} \text{ get} \text{ area}\\
x^2 + 3 x = 40 & & \text{ Distribute}\\
\underline{- 40 - 40} & & \text{ Make} \text{ equation} \text{ equal}
\text{ zero}\\
x^2 + 3 x - 40 = 0 & & \text{ Factor}\\
(x - 5) (x + 8) = 0 & & \text{ Set} \text{ each} \text{ factor} \text{ equal}
\text{ to} \text{ zero}\\
x - 5 = 0 \text{ or}\, x + 8 = 0 & & \text{ Solve} \text{ each}
\text{ equation}\\
x = 5 \text{ or}\, x = - 8 & & \text{ Our}\, x \text{ is} a \text{ width},
\text{ cannot} \text{ be} \text{ negative} .\\
(5) + 3 = 8 & & \text{ Length} \text{ is}\, x + 3, \text{ substitute} 5
\text{ for}\, x \text{ to} \text{ find} \text{ length}\\
5 \text{ in} \text{ by}\, 8 \text{ in} & & \text{ Our} \text{ Solution}
\end{eqnarray*}
Answer
The dimensions of the rectangle are 5 inches by 8 inches.
In the following video you are shown how to find the dimensions of a border around a rectangle.
https://www.youtube.com/watch?v=AlIoxXQ-V50
Try It
[ohm_question]74417[/ohm_question]
Cost, Revenue, and Profit Polynomials
In the systems of linear equations section, we discussed how a company's cost and revenue can be modeled with two linear equations. We found that the profit region for a company was the area between the two lines where the company would make money based on how much was produced. In this section, we will see that sometimes polynomials are used to describe cost and revenue.
Profit is typically defined in business as the difference between the amount of money earned (revenue) by producing a certain number of items and the amount of money it takes to produce that number of items. When you are in business, you definitely want to see profit, so it is important to know what your cost and revenue is.

Cell Phones
P = R-C
The example we will work with is a hypothetical cell phone manufacturer whose cost to manufacture x number of phones is C=2000x+750,000, and the Revenue generated from manufacturing x number of cell phones is R=−0.09x2+7000x.
Example
Define a Profit polynomial for the hypothetical cell phone manufacturer.
Answer:
Read and Understand: Profit is the difference between revenue and cost, so we will need to define P = R - C for the company.
Define and Translate: R=−0.09x2+7000xC=2000x+750,000
Write and Solve: Substitute the expressions for R and C into the Profit equation.
P=R−C=−0.09x2+7000x−(2000x+750,000)=−0.09x2+7000x−2000x−750,000=−0.09x2+5000x−750,000
Remember that when you subtract a polynomial, you have to subtract every term of the polynomial.
Answer
P=−0.09x2+5000x−750,000
Mathematical models are great when you use them to learn important information. The cell phone manufacturing company can use the profit equation to find out how much profit they will make given x number of phones are manufactured. In the next example, we will explore some profit values for this company.
Example
Given the following numbers of cell phones manufactured, find the profit for the cell phone manufacturer:
- x = 100 phones
- x = 25,000 phones
- x=60,000 phones
Interpret your results.
Answer:
Read and Understand: The profit polynomial defined in the previous example,P=−0.09x2+5000x−750,000, gives profit based on x number of phones manufactured. We need to substitute the given numbers of phones manufactured into the equation, then try to understand what our answer means in terms of profit and number of phones manufactured.
We will move straight into write and solve since we already have our polynomial. It is probably easiest to use a calculator since the numbers in this problem are so large.
Write and Solve:
Substitute x = 100
P=−0.09x2+5000x−750,000=−0.09(100)2+5000(100)−750,000=−900+500,000−750,000=−250,900
Interpret: When the number of phones manufactured is 100, the profit for the business is $-250,000. This is not what we want! The company must produce more than 100 phones to make a profit.
Write and Solve:
Substitute x = 25,000
P=−0.09x2+5000x−750,000=−0.09(25000)2+5000(25000)−750,000=−6120000+125,000,000−750,000=118,130,000
Interpret: When the number of phones manufactured is 25,000, the profit for the business is $118,130,000. This is more like it! If the company makes 25,000 phones it will make a profit after it pays all it's bills.
If this is true, then the company should make even more phones so it can make even more money, right? Actually, something different happens as the number of items manufactured increases without bound.
Write and Solve:
Substitute x = 60,000
P=−0.09x2+5000x−750,000=−0.09(60000)2+5000(60000)−750,000=−324,000,000+300,000,000−750,000=−24,750,000
Interpret: When the number of phones manufactured is 60,000, the profit for the business is $-24,750,000. Wait a minute! If the company makes 60,000 phones it will lose money, what happened? At some point, the cost to manufacture the phones will overcome the amount of profit that the business can make. If this is interesting to you, you may enjoy reading about Economics and Business models.
In the video that follows, we present another example of finding a polynomial profit equation.
https://youtu.be/-TWjDC4g9dULicenses & Attributions
CC licensed content, Original
- Solve a Quadratic Equations with Fractions by Factoring (a not 1). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
- Parabolic motion description and example. Provided by: Lumen Learning License: CC BY: Attribution.
- Factoring Application - Find the Time When a Projectile Hits and Ground. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Pythagorean Theorem, Description and Examples. Provided by: Lumen Learning License: CC BY: Attribution.
- Screenshot: Thumbsdown. Provided by: Lumen Learning License: CC BY: Attribution.
- Screenshot PI. Provided by: Lumen Learning License: CC BY: Attribution.
- Polynomial Multiplication Application - Volume of a Cylinder. . Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Polynomial Subtracton App - Profit Equation from Revene and Cost.. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning.. License: CC BY: Attribution.
- Screenshot: Cell Phones.. Authored by: Lumen Learning. License: CC BY: Attribution.
- Profit Polynomial Examples. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- Ex: Factor and Solve Quadratic Equation - Greatest Common Factor Only. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: Factor and Solve Quadratic Equations When A equals 1. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Unit 12: Factoring, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology Located at: https://www.nroc.org/. License: CC BY: Attribution.
- Parabolic water trajectory. Authored by: By GuidoB. Located at: https://commons.wikimedia.org/w/index.php?curid=8015696. License: CC BY-SA: Attribution-ShareAlike.
- Celestial Navigation Math. Authored by: TabletClass Math. License: All Rights Reserved. License terms: Standard YouTube License.
- Pythagorean Theorem. Provided by: Wikipedia Located at: https://en.wikipedia.org/wiki/Pythagorean_theorem. License: CC BY-SA: Attribution-ShareAlike.
- Ex: Polynomial Addition Application - Perimeter.. Authored by: James Sousa (Mathispower4u.com). . License: CC BY: Attribution.
- Ex: Find the Area of a Rectangle Using a Polynomial.. Authored by: James Sousa (Mathispower4u.com) .. License: CC BY: Attribution.
- Unit 11: Exponents and Polynomials, from Developmental Math: An Open Program. . Provided by: Monterey Institute of Technology and Education Located at: https://www.nroc.org/. License: CC BY: Attribution.
- Applied Optimization Problems. Provided by: OpenStax Located at: https://cnx.org/contents/svyieFe9@2/Applied-Optimization-Problems. License: CC BY-NC-SA: Attribution-NonCommercial-ShareAlike. License terms: Download for free at http://cnx.org/contents/b2fca278-57bd-421d-aa85-21f539b4cc6f@2.
- Applications of Quadratic Equations. Authored by: James Sousa. License: CC BY: Attribution.
- Quadratics - Rectangle. Authored by: Tyler Wallace. Located at: http://www.wallace.ccfaculty.org/book/book.html. License: Public Domain: No Known Copyright.
- Question ID#74417. Authored by: Nearing,Daniel. License: CC BY: Attribution.
 Zero
Zero Parabolic WaterTrajectory
Parabolic WaterTrajectory
 Right Triangle Labeled
Right Triangle Labeled

 Cell Phones
Cell Phones