We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

学習ガイド > College Algebra: Co-requisite Course

Real Numbers Review

Learning Objectives

  • Real numbers
  • Add and subtract real numbers
    • Add real numbers with the same and different signs
    • Subtract real numbers with the same and different signs
    • Simplify combinations that require both addition and subtraction of real numbers.
  • Multiply and divide real numbers
    • Multiply two or more real numbers.
    • Divide real numbers
    • Simplify expressions with both multiplication and division
  • Properties of real numbers
 

Real Numbers

Given any number n, we know that n is either rational or irrational. It cannot be both. The sets of rational and irrational numbers together make up the set of real numbers. As we saw with integers, the real numbers can be divided into three subsets: negative real numbers, zero, and positive real numbers. Each subset includes fractions, decimals, and irrational numbers according to their algebraic sign (+ or –). Zero is considered neither positive nor negative. The real numbers can be visualized on a horizontal number line with an arbitrary point chosen as 0, with negative numbers to the left of 0 and positive numbers to the right of 0. A fixed unit distance is then used to mark off each integer (or other basic value) on either side of 0. Any real number corresponds to a unique position on the number line.The converse is also true: Each location on the number line corresponds to exactly one real number. This is known as a one-to-one correspondence. We refer to this as the real number line.
A number line that is marked from negative five to five The real number line

Example: Classifying Real Numbers

Classify each number as either positive or negative and as either rational or irrational. Does the number lie to the left or the right of 0 on the number line?
  1. [latex]-\frac{10}{3}[/latex]
  2. [latex]-6\pi[/latex]
  3. [latex]0.616161\dots[/latex]
  4. [latex] 0.13 [/latex]

Answer:

  1. [latex]-\frac{10}{3}[/latex] is negative and rational. It lies to the left of 0 on the number line.
  2. [latex]-6\pi [/latex] is negative and irrational. It lies to the left of 0.
  3. [latex]0.616161\dots [/latex] is a repeating decimal so it is rational and positive. It lies to the right of 0.
  4. [latex] 0.13 [/latex] is a finite decimal and may be written as 13/100.  So it is rational and positive.

Try It

Classify each number as either positive or negative and as either rational or irrational. Does the number lie to the left or the right of 0 on the number line?
  1. [latex]2\pi[/latex]
  2. [latex]-11.411411411\dots [/latex]
  3. [latex]\frac{47}{19}[/latex]
  4. [latex]6.210735[/latex]

Answer:

  1. positive, irrational; right
  2. negative, rational; left
  3. positive, rational; right
  4. positive, rational; right

Sets of Numbers as Subsets

Beginning with the natural numbers, we have expanded each set to form a larger set, meaning that there is a subset relationship between the sets of numbers we have encountered so far. These relationships become more obvious when seen as a diagram.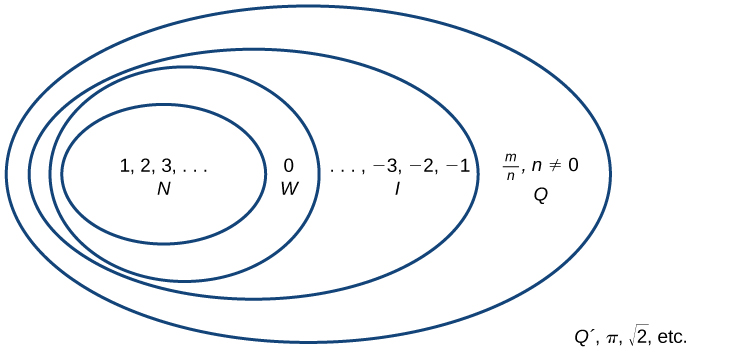
A large box labeled: Real Numbers encloses five circles. Four of these circles enclose each other and the other is separate from the rest. The innermost circle contains: 1, 2, 3… N. The circle enclosing that circle contains: 0 W. The circle enclosing that circle contains: …, -3, -2, -1 I. The outermost circle contains: m/n, n not equal to zero Q. The separate circle contains: pi, square root of two, etc Q´. Sets of numbers.   N: the set of natural numbers   W: the set of whole numbers   I: the set of integers   Q: the set of rational numbers   Q´: the set of irrational numbers

A General Note: Sets of Numbers

The set of natural numbers includes the numbers used for counting: [latex]\{1,2,3,\dots\}[/latex]. The set of whole numbers is the set of natural numbers plus zero: [latex]\{0,1,2,3,\dots\}[/latex]. The set of integers adds the negative natural numbers to the set of whole numbers: [latex]\{\dots,-3,-2,-1,0,1,2,3,\dots\}[/latex]. The set of rational numbers includes fractions written as [latex]\{\frac{m}{n}|m\text{ and }n\text{ are integers and }n\ne 0\}[/latex]. The set of irrational numbers is the set of numbers that are not rational, are nonrepeating, and are nonterminating: [latex]\{h|h\text{ is not a rational number}\}[/latex].
The ability to work comfortably with negative numbers is essential to success in algebra. For this reason we will do a quick review of adding, subtracting, multiplying and dividing integers. Integers are all the positive whole numbers, zero, and their opposites (negatives). As this is intended to be a review of integers, the descriptions and examples will not be as detailed as a normal lesson.

Adding and Subtracting Real Numbers

When adding integers we have two cases to consider. The first case is whether the signs match (both positive or both negative). If the signs match, we will add the numbers together and keep the sign. If the signs don’t match (one positive and one negative number) we will subtract the numbers (as if they were all positive) and then use the sign from the larger number. This means if the larger number is positive, the answer is positive. If the larger number is negative, the answer is negative.

To add two numbers with the same sign (both positive or both negative)

  • Add their absolute values (without the [latex]+[/latex] or [latex]-[/latex] sign)
  • Give the sum the same sign.

To add two numbers with different signs (one positive and one negative)

  • Find the difference of their absolute values. (Note that when you find the difference of the absolute values, you always subtract the lesser absolute value from the greater one.)
  • Give the sum the same sign as the number with the greater absolute value.

Example

Find [latex]23–73[/latex].

Answer: You can't use your usual method of subtraction because 73 is greater than 23. Rewrite the subtraction as adding the opposite.

[latex]23+\left(−73\right)[/latex]

The addends have different signs, so find the difference of their absolute values.

[latex]\begin{array}{c}\left|23\right|=23\,\,\,\text{and}\,\,\,\left|−73\right|=73\\73-23=50\end{array}[/latex]

Since [latex]\left|−73\right|>\left|23\right|[/latex], the final answer is negative.

Answer

[latex-display]23–73=−50[/latex-display]

  Another way to think about subtracting is to think about the distance between the two numbers on the number line. In the example below, [latex]382[/latex] is to the right of 0 by [latex]382[/latex] units, and [latex]−93[/latex] is to the left of 0 by 93 units. The distance between them is the sum of their distances to 0: [latex]382+93[/latex]. A number line from negative 93 to 382. Negative 93 is 93 units from 0 and 382 is 382 units from 0. The total distance from negative 93 to 382 can be found by adding them together. 382+93=475 units.

Example

Find [latex]382–\left(−93\right)[/latex].

Answer: You are subtracting a negative, so think of this as taking the negative sign away. This becomes an addition problem. [latex]-93[/latex] becomes [latex]+93[/latex]

[latex]382+93=475[/latex]

Answer

[latex-display]382–(−93)=475[/latex-display]

The following video explains how to subtract two signed integers. https://youtu.be/ciuIKFCtWWU

Example

Find [latex]-\frac{3}{7}-\frac{6}{7}+\frac{2}{7}[/latex]

Answer: Add the first two and give the result a negative sign: Since the signs of the first two are the same, find the sum of the absolute values of the fractions Since both numbers are negative, the sum is negative. If you owe money, then borrow more, the amount you owe becomes larger.

[latex]\left| -\frac{3}{7} \right|=\frac{3}{7}[/latex] and [latex]\left| -\frac{6}{7} \right|=\frac{6}{7}[/latex]

[latex]\begin{array}{c}\frac{3}{7}+\frac{6}{7}=\frac{9}{7}\\\\-\frac{3}{7}-\frac{6}{7} =-\frac{9}{7}\end{array}[/latex]

Now add the third number. The signs are different, so find the difference of their absolute values.

[latex] \left| -\frac{9}{7} \right|=\frac{9}{7}[/latex] and [latex] \left| \frac{2}{7} \right|=\frac{2}{7}[/latex]

[latex] \frac{9}{7}-\frac{2}{7}=\frac{7}{7}[/latex]

Since [latex]\left|\frac{-9}{7}\right|>\left|\frac{2}{7}\right|[/latex], the sign of the final sum is the same as the sign of [latex]-\frac{9}{7}[/latex].

[latex] -\frac{9}{7}+\frac{2}{7}=-\frac{7}{7}[/latex]

Answer

[latex-display]-\frac{3}{7}+\left(-\frac{6}{7}\right)+\frac{2}{7}=-\frac{7}{7}[/latex-display]

In the following video you will see an example of how to add three fractions with a common denominator that have different signs. https://youtu.be/P972VVbR98k

Example

Evaluate [latex]27.832+(−3.06)[/latex]. When you add decimals, remember to line up the decimal points so you are adding tenths to tenths, hundredths to hundredths, and so on.

Answer: Since the addends have different signs, subtract their absolute values.

[latex] \begin{array}{r}\underline{\begin{array}{r}27.832\\-\text{ }3.06\,\,\,\end{array}}\\24.772\end{array}[/latex]

[latex]\left|-3.06\right|=3.06[/latex]

The sum has the same sign as 27.832 whose absolute value is greater.

Answer

[latex-display]27.832+\left(-3.06\right)=24.772[/latex-display]

In the following video are examples of adding and subtracting decimals with different signs. https://youtu.be/3FHZQ5iKcpI

Multiplying and Dividing Real Numbers

Multiplication and division are inverse operations, just as addition and subtraction are. You may recall that when you divide fractions, you multiply by the reciprocal. Inverse operations "undo" each other.

Multiply Real Numbers

Multiplying real numbers is not that different from multiplying whole numbers and positive fractions. However, you haven't learned what effect a negative sign has on the product. With whole numbers, you can think of multiplication as repeated addition. Using the number line, you can make multiple jumps of a given size. For example, the following picture shows the product [latex]3\cdot4[/latex] as 3 jumps of 4 units each.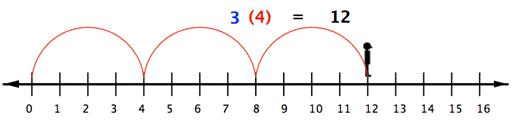 A number line showing 3 times 4 is 12. From the 0, the right-facing person jumps 4 units at a time, and jumps 3 times. The person lands on 12. So to multiply [latex]3(−4)[/latex], you can face left (toward the negative side) and make three “jumps” forward (in a negative direction). A number line representing 3 times negative 4 equals negative 12. A left-facing person jumps left 4 spaces 3 times so that the person lands on negative 12. The product of a positive number and a negative number (or a negative and a positive) is negative.

The Product of a Positive Number and a Negative Number

To multiply a positive number and a negative number, multiply their absolute values. The product is negative.

Example

Find [latex]−3.8(0.6)[/latex].

Answer: Multiply the absolute values as you normally would. Place the decimal point by counting place values. 3.8 has 1 place after the decimal point, and 0.6 has 1 place after the decimal point, so the product has [latex]1+1[/latex] or 2 places after the decimal point.

[latex]\begin{array}{r}3.8\\\underline{\times\,\,\,0.6}\\2.28\end{array}[/latex]

The product of a negative and a positive is negative.

Answer

[latex-display]−3.8(0.6)=−2.28[/latex-display]

The following video contains examples of how to multiply decimal numbers with different signs. https://youtu.be/7gY0S3LUUyQ

The Product of Two Numbers with the Same Sign (both positive or both negative)

To multiply two positive numbers, multiply their absolute values. The product is positive. To multiply two negative numbers, multiply their absolute values. The product is positive.

Example

Find [latex] ~\left( -\frac{3}{4} \right)\left( -\frac{2}{5} \right)[/latex]

Answer: Multiply the absolute values of the numbers. First, multiply the numerators together to get the product's numerator. Then, multiply the denominators together to get the product's denominator. Rewrite in lowest terms, if needed.

[latex] \left( \frac{3}{4} \right)\left( \frac{2}{5} \right)=\frac{6}{20}=\frac{3}{10}[/latex]

The product of two negative numbers is positive.

Answer

[latex-display] \left( -\frac{3}{4} \right)\left( -\frac{2}{5} \right)=\frac{3}{10}[/latex-display]

The following video shows examples of multiplying two signed fractions, including simplification of the answer. https://youtu.be/yUdJ46pTblo To summarize:
  • positive [latex]\cdot[/latex] positive: The product is positive.
  • negative [latex]\cdot[/latex] negative: The product is positive.
  • negative [latex]\cdot[/latex] positive: The product is negative.
  • positive [latex]\cdot[/latex] negative: The product is negative.
You can see that the product of two negative numbers is a positive number. So, if you are multiplying more than two numbers, you can count the number of negative factors.

Multiplying More Than Two Negative Numbers

If there are an even number (0, 2, 4, ...) of negative factors to multiply, the product is positive. If there are an odd number (1, 3, 5, ...) of negative factors, the product is negative.

Example

Find [latex]3(−6)(2)(−3)(−1)[/latex].

Answer: Multiply the absolute values of the numbers.

[latex]\begin{array}{l}3(6)(2)(3)(1)\\18(2)(3)(1)\\36(3)(1)\\108(1)\\108\end{array}[/latex]

Count the number of negative factors. There are three [latex]\left(−6,−3,−1\right)[/latex].

[latex]3(−6)(2)(−3)(−1)[/latex]

Since there are an odd number of negative factors, the product is negative.

Answer

[latex-display]3(−6)(2)(−3)(−1)=−108[/latex-display]

The following video contains examples of multiplying more than two signed integers. https://youtu.be/rx8F9SPd0HE

Divide Real Numbers

You may remember that when you divided fractions, you multiplied by the reciprocal. Reciprocal is another name for the multiplicative inverse (just as opposite is another name for additive inverse). An easy way to find the multiplicative inverse is to just “flip” the numerator and denominator as you did to find the reciprocal. Here are some examples:
  • The reciprocal of [latex]\frac{4}{9}[/latex] is [latex] \frac{9}{4}[/latex]because [latex]\frac{4}{9}\left(\frac{9}{4}\right)=\frac{36}{36}=1[/latex].
  • The reciprocal of 3 is [latex]\frac{1}{3}[/latex] because [latex]\frac{3}{1}\left(\frac{1}{3}\right)=\frac{3}{3}=1[/latex].
  • The reciprocal of [latex]-\frac{5}{6}[/latex] is [latex]\frac{-6}{5}[/latex] because [latex]-\frac{5}{6}\left( -\frac{6}{5} \right)=\frac{30}{30}=1[/latex].
  • The reciprocal of 1 is 1 as [latex]1(1)=1[/latex].
When you divided by positive fractions, you learned to multiply by the reciprocal. You also do this to divide real numbers. Think about dividing a bag of 26 marbles into two smaller bags with the same number of marbles in each. You can also say each smaller bag has one half of the marbles.

[latex] 26\div 2=26\left( \frac{1}{2} \right)=13[/latex]

Notice that 2 and [latex] \frac{1}{2}[/latex] are reciprocals. Try again, dividing a bag of 36 marbles into smaller bags.
Number of bags Dividing by number of bags Multiplying by reciprocal
3 [latex]\frac{36}{3}=12[/latex] [latex] 36\left( \frac{1}{3} \right)=\frac{36}{3}=\frac{12(3)}{3}=12[/latex]
4 [latex]\frac{36}{4}=9[/latex] [latex]36\left(\frac{1}{4}\right)=\frac{36}{4}=\frac{9\left(4\right)}{4}=9[/latex]
6 [latex]\frac{36}{6}=6[/latex] [latex]36\left(\frac{1}{6}\right)=\frac{36}{6}=\frac{6\left(6\right)}{6}=6[/latex]
Dividing by a number is the same as multiplying by its reciprocal. (That is, you use the reciprocal of the divisor, the second number in the division problem.)

Example

Find [latex] 28\div \frac{4}{3}[/latex]

Answer: Rewrite the division as multiplication by the reciprocal. The reciprocal of [latex] \frac{4}{3}[/latex] is [latex]\frac{3}{4} [/latex].

[latex] 28\div \frac{4}{3}=28\left( \frac{3}{4} \right)[/latex]

Multiply.

[latex]\frac{28}{1}\left(\frac{3}{4}\right)=\frac{28\left(3\right)}{4}=\frac{4\left(7\right)\left(3\right)}{4}=7\left(3\right)=21[/latex]

Answer

[latex-display]28\div\frac{4}{3}=21[/latex-display]

Now let's see what this means when one or more of the numbers is negative. A number and its reciprocal have the same sign. Since division is rewritten as multiplication using the reciprocal of the divisor, and taking the reciprocal doesn’t change any of the signs, division follows the same rules as multiplication.

Rules of Division

When dividing, rewrite the problem as multiplication using the reciprocal of the divisor as the second factor. When one number is positive and the other is negative, the quotient is negative. When both numbers are negative, the quotient is positive. When both numbers are positive, the quotient is positive.

Example

Find [latex]24\div\left(-\frac{5}{6}\right)[/latex].

Answer: Rewrite the division as multiplication by the reciprocal.

[latex] 24\div \left( -\frac{5}{6} \right)=24\left( -\frac{6}{5} \right)[/latex]

Multiply. Since one number is positive and one is negative, the product is negative.

[latex] \frac{24}{1}\left( -\frac{6}{5} \right)=-\frac{144}{5}[/latex]

Answer

[latex-display] 24\div \left( -\frac{5}{6} \right)=-\frac{144}{5}[/latex-display]

Example

Find [latex] 4\,\left( -\frac{2}{3} \right)\,\div \left( -6 \right)[/latex]

Answer: Rewrite the division as multiplication by the reciprocal.

[latex] \frac{4}{1}\left( -\frac{2}{3} \right)\left( -\frac{1}{6} \right)[/latex]

Multiply. There is an even number of negative numbers, so the product is positive.

[latex]\frac{4\left(2\right)\left(1\right)}{3\left(6\right)}=\frac{8}{18}[/latex]

Write the fraction in lowest terms.

Answer

[latex-display] 4\left( -\frac{2}{3} \right)\div \left( -6 \right)=\frac{4}{9}[/latex-display]

The following video explains how to divide signed fractions. https://youtu.be/OPHdadhDJoI
traffic-sign-160659 Remember that a fraction bar also indicates division, so a negative sign in front of a fraction goes with the numerator, the denominator, or the whole fraction: [latex]-\frac{3}{4}=\frac{-3}{4}=\frac{3}{-4}[/latex]. In each case, the overall fraction is negative because there's only one negative in the division.

Properties of Real Numbers

For some activities we perform, the order of certain operations does not matter, but the order of other operations does. For example, it does not make a difference if we put on the right shoe before the left or vice-versa. However, it does matter whether we put on shoes or socks first. The same thing is true for operations in mathematics.

Commutative Properties

The commutative property of addition states that numbers may be added in any order without affecting the sum.
[latex]a+b=b+a[/latex]
We can better see this relationship when using real numbers.
[latex]\left(-2\right)+7=5\text{ and }7+\left(-2\right)=5[/latex]
Similarly, the commutative property of multiplication states that numbers may be multiplied in any order without affecting the product.
[latex]a\cdot b=b\cdot a[/latex]
Again, consider an example with real numbers.
[latex]\left(-11\right)\cdot\left(-4\right)=44\text{ and }\left(-4\right)\cdot\left(-11\right)=44[/latex]
It is important to note that neither subtraction nor division is commutative. For example, [latex]17 - 5[/latex] is not the same as [latex]5 - 17[/latex]. Similarly, [latex]20\div 5\ne 5\div 20[/latex].

Associative Properties

The associative property of multiplication tells us that it does not matter how we group numbers when multiplying. We can move the grouping symbols to make the calculation easier, and the product remains the same.
[latex]a\left(bc\right)=\left(ab\right)c[/latex]
Consider this example.
[latex]\left(3\cdot4\right)\cdot5=60\text{ and }3\cdot\left(4\cdot5\right)=60[/latex]
The associative property of addition tells us that numbers may be grouped differently without affecting the sum.
[latex]a+\left(b+c\right)=\left(a+b\right)+c[/latex]
This property can be especially helpful when dealing with negative integers. Consider this example.
[latex][15+\left(-9\right)]+23=29\text{ and }15+[\left(-9\right)+23]=29[/latex]
Are subtraction and division associative? Review these examples.
[latex]\begin{array}\text{ }8-\left(3-15\right) \hfill& \stackrel{?}{=}\left(8-3\right)-15 \\ 8-\left(-12\right) \hfill& =5-15 \\ 20 \hfill& \neq 20-10 \\ \text{ }\end{array}[/latex]
[latex]\begin{array}\text{ }64\div\left(8\div4\right)\hfill&\stackrel{?}{=}\left(64\div8\right)\div4 \\ 64\div2 \hfill& \stackrel{?}{=}8\div4 \\ 32 \hfill& \neq 2\end{array}[/latex]
As we can see, neither subtraction nor division is associative.

Distributive Property

The distributive property states that the product of a factor times a sum is the sum of the factor times each term in the sum.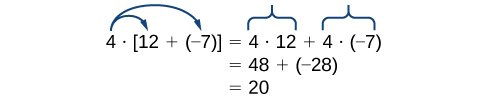
[latex]a\cdot \left(b+c\right)=a\cdot b+a\cdot c[/latex]
This property combines both addition and multiplication (and is the only property to do so). Let us consider an example. The number four is separated by a multiplication symbol from a bracketed expression reading: twelve plus negative seven. Arrows extend from the four pointing to the twelve and negative seven separately. This expression equals four times twelve plus four times negative seven. Under this line the expression reads forty eight plus negative twenty eight. Under this line the expression reads twenty as the answer. Note that 4 is outside the grouping symbols, so we distribute the 4 by multiplying it by 12, multiplying it by –7, and adding the products. To be more precise when describing this property, we say that multiplication distributes over addition. The reverse is not true, as we can see in this example.
[latex]\begin{array}{ccc}\hfill 6+\left(3\cdot 5\right)& \stackrel{?}{=}& \left(6+3\right)\cdot \left(6+5\right) \\ \hfill 6+\left(15\right)& \stackrel{?}{=}& \left(9\right)\cdot \left(11\right)\hfill \\ \hfill 21& \ne & \text{ }99\hfill \end{array}[/latex]
Multiplication does not distribute over subtraction, and division distributes over neither addition nor subtraction. A special case of the distributive property occurs when a sum of terms is subtracted.
[latex]a-b=a+\left(-b\right)[/latex]
For example, consider the difference [latex]12-\left(5+3\right)[/latex]. We can rewrite the difference of the two terms 12 and [latex]\left(5+3\right)[/latex] by turning the subtraction expression into addition of the opposite. So instead of subtracting [latex]\left(5+3\right)[/latex], we add the opposite.
[latex]12+\left(-1\right)\cdot \left(5+3\right)[/latex]
Now, distribute [latex]-1[/latex] and simplify the result.
[latex]\begin{array}12-\left(5+3\right) \hfill& =12+\left(-1\right)\cdot\left(5+3\right) \\ \hfill& =12+[\left(-1\right)\cdot5+\left(-1\right)\cdot3] \\ \hfill& =12+\left(-8\right) \\ \hfill& =4 \end{array}[/latex]
This seems like a lot of trouble for a simple sum, but it illustrates a powerful result that will be useful once we introduce algebraic terms. To subtract a sum of terms, change the sign of each term and add the results. With this in mind, we can rewrite the last example.
[latex]\begin{array}12-\left(5+3\right) \hfill& =12+\left(-5-3\right) \\ \hfill& =12+\left(-8\right) \\ \hfill& =4\end{array}[/latex]

Identity Properties

The identity property of addition states that there is a unique number, called the additive identity (0) that, when added to a number, results in the original number.
[latex]a+0=a[/latex]
The identity property of multiplication states that there is a unique number, called the multiplicative identity (1) that, when multiplied by a number, results in the original number.
[latex]a\cdot 1=a[/latex]
For example, we have [latex]\left(-6\right)+0=-6[/latex] and [latex]23\cdot 1=23[/latex]. There are no exceptions for these properties; they work for every real number, including 0 and 1.

Inverse Properties

The inverse property of addition states that, for every real number a, there is a unique number, called the additive inverse (or opposite), denoted−a, that, when added to the original number, results in the additive identity, 0.
[latex]a+\left(-a\right)=0[/latex]
For example, if [latex]a=-8[/latex], the additive inverse is 8, since [latex]\left(-8\right)+8=0[/latex]. The inverse property of multiplication holds for all real numbers except 0 because the reciprocal of 0 is not defined. The property states that, for every real number a, there is a unique number, called the multiplicative inverse (or reciprocal), denoted [latex]\frac{1}{a}[/latex], that, when multiplied by the original number, results in the multiplicative identity, 1.
[latex]a\cdot \frac{1}{a}=1[/latex]
For example, if [latex]a=-\frac{2}{3}[/latex], the reciprocal, denoted [latex]\frac{1}{a}[/latex], is [latex]-\frac{3}{2}[/latex] because
[latex]a\cdot \frac{1}{a}=\left(-\frac{2}{3}\right)\cdot \left(-\frac{3}{2}\right)=1[/latex]

A General Note: Properties of Real Numbers

The following properties hold for real numbers a, b, and c.
Addition Multiplication
Commutative Property [latex]a+b=b+a[/latex] [latex]a\cdot b=b\cdot a[/latex]
Associative Property [latex]a+\left(b+c\right)=\left(a+b\right)+c[/latex] [latex]a\left(bc\right)=\left(ab\right)c[/latex]
Distributive Property [latex]a\cdot \left(b+c\right)=a\cdot b+a\cdot c[/latex]
Identity Property There exists a unique real number called the additive identity, 0, such that, for any real number a
[latex]a+0=a[/latex]
 There exists a unique real number called the multiplicative identity, 1, such that, for any real number a
[latex]a\cdot 1=a[/latex]
Inverse Property Every real number a has an additive inverse, or opposite, denoted –a, such that
[latex]a+\left(-a\right)=0[/latex]
 Every nonzero real number a has a multiplicative inverse, or reciprocal, denoted [latex]\frac{1}{a}[/latex], such that
[latex]a\cdot \left(\frac{1}{a}\right)=1[/latex]

Example: Using Properties of Real Numbers

Use the properties of real numbers to rewrite and simplify each expression. State which properties apply.
  1. [latex]3\cdot 6+3\cdot 4[/latex]
  2. [latex]\left(5+8\right)+\left(-8\right)[/latex]
  3. [latex]6-\left(15+9\right)[/latex]
  4. [latex]\frac{4}{7}\cdot \left(\frac{2}{3}\cdot \frac{7}{4}\right)[/latex]
  5. [latex]100\cdot \left[0.75+\left(-2.38\right)\right][/latex]

Answer:

  1. [latex]\begin{array}\text{ }3\cdot6+3\cdot4 \hfill& =3\cdot\left(6+4\right) \hfill& \text{Distributive property} \\ \hfill& =3\cdot10 \hfill& \text{Simplify} \\ \hfill& =30 \hfill& \text{Simplify}\end{array}[/latex]
  2. [latex]\begin{array}\text{ }\left(5+8\right)+\left(-8\right) \hfill& =5+\left[8+\left(-8\right)\right] \hfill& \text{Associative property of addition} \\ &\hfill =5+0 \hfill& \text{Inverse property of addition} \\ \hfill& =5 \hfill& \text{Identity property of addition}\end{array}[/latex]
  3. [latex]\begin{array}6-\left(15+9\right) \hfill& =6+[15\left(-15\right)+\left(-9\right)] \hfill& \text{Distributive property} \\ \hfill& =6+\left(-24\right) \hfill& \text{Simplify} \\ \hfill& =-18 \hfill& \text{Simplify}\end{array}[/latex]
  4. [latex]\begin{array}\text{ }\frac{4}{7}\cdot\left(\frac{2}{3}\cdot\frac{7}{4}\right) \hfill& =\frac{4}{7} \cdot\left(\frac{7}{4}\cdot\frac{2}{3}\right) \hfill& \text{Commutative property of multiplication} \\ \hfill& =\left(\frac{4}{7}\cdot\frac{7}{4}\right)\cdot\frac{2}{3}\hfill& \text{Associative property of multiplication} \\ \hfill& =1\cdot\frac{2}{3} \hfill& \text{Inverse property of multiplication} \\ \hfill& =\frac{2}{3} \hfill& \text{Identity property of multiplication}\end{array}[/latex]
  5. [latex]\begin{array}\text{ }100\cdot[0.75+\left(-2.38\right)] \hfill& =100\cdot0.75+100\cdot\left(-2.38\right)\hfill& \text{Distributive property} \\ \hfill& =75+\left(-238\right) \hfill& \text{Simplify} \\ \hfill& =-163 \hfill& \text{Simplify}\end{array}[/latex]

Try It

Use the properties of real numbers to rewrite and simplify each expression. State which properties apply.
  1. [latex]\left(-\frac{23}{5}\right)\cdot \left[11\cdot \left(-\frac{5}{23}\right)\right][/latex]
  2. [latex]5\cdot \left(6.2+0.4\right)[/latex]
  3. [latex]18-\left(7 - 15\right)[/latex]
  4. [latex]6\cdot \left(-3\right)+6\cdot 3[/latex]

Answer:

  1. commutative property of multiplication, associative property of multiplication, inverse property of multiplication, identity property of multiplication;
  2. 33, distributive property;
  3. 26, distributive property;
  4. 0, distributive property, inverse property of addition, identity property of addition

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • Ex 2: Subtracting Integers (Two Digit Integers). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
  • Find the Sum and Difference of Three Signed Fractions (Common Denom). Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
  • Unit 9: Real Numbers, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology and Education Located at: https://www.nroc.org/. License: CC BY: Attribution.
  • Ex: Multiplying Three or More Integers. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
  • Ex: Multiplying Signed Fractions . Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
  • Ex 1: Dividing Signed Fractions. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
  • College Algebra. Provided by: OpenStax Authored by: College Algebra. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
  • Identifying Sets of Real Numbers. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.