The Parabola*
Did you know that the Olympic torch is lit several months before the start of the games? The ceremonial method for lighting the flame is the same as in ancient times. The ceremony takes place at the Temple of Hera in Olympia, Greece, and is rooted in Greek mythology, paying tribute to Prometheus, who stole fire from Zeus to give to all humans. One of eleven acting priestesses places the torch at the focus of a parabolic mirror, which focuses light rays from the sun to ignite the flame. The Olympic torch concludes its journey around the world when it is used to light the Olympic cauldron during the opening ceremony. (credit: Ken Hackman, U.S. Air Force)
The Olympic torch concludes its journey around the world when it is used to light the Olympic cauldron during the opening ceremony. (credit: Ken Hackman, U.S. Air Force)Parabolas with Vertices at the Origin
In The Ellipse, we saw that an ellipse is formed when a plane cuts through a right circular cone. If the plane is parallel to the edge of the cone, an unbounded curve is formed. This curve is a parabola.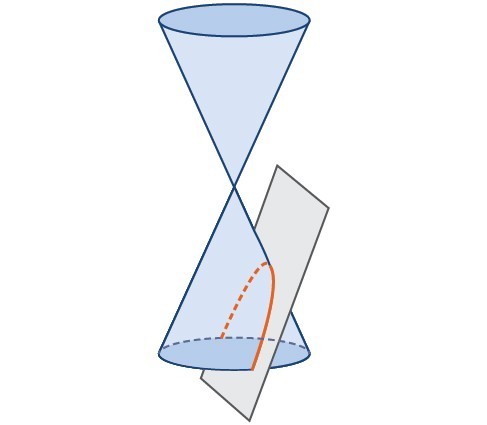 Parabola
Parabola Key features of the parabola
Key features of the parabola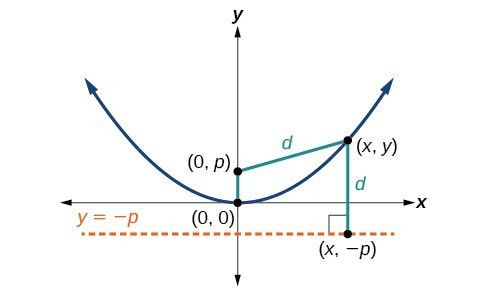 Let [latex]\left(x,y\right)[/latex] be a point on the parabola with vertex [latex]\left(0,0\right)[/latex], focus [latex]\left(0,p\right)[/latex], and directrix [latex]y= -p[/latex] as shown in Figure 4. The distance [latex]d[/latex] from point [latex]\left(x,y\right)[/latex] to point [latex]\left(x,-p\right)[/latex] on the directrix is the difference of the y-values: [latex]d=y+p[/latex]. The distance from the focus [latex]\left(0,p\right)[/latex] to the point [latex]\left(x,y\right)[/latex] is also equal to [latex]d[/latex] and can be expressed using the distance formula.
Let [latex]\left(x,y\right)[/latex] be a point on the parabola with vertex [latex]\left(0,0\right)[/latex], focus [latex]\left(0,p\right)[/latex], and directrix [latex]y= -p[/latex] as shown in Figure 4. The distance [latex]d[/latex] from point [latex]\left(x,y\right)[/latex] to point [latex]\left(x,-p\right)[/latex] on the directrix is the difference of the y-values: [latex]d=y+p[/latex]. The distance from the focus [latex]\left(0,p\right)[/latex] to the point [latex]\left(x,y\right)[/latex] is also equal to [latex]d[/latex] and can be expressed using the distance formula.
[latex]\begin{array}{l}d=\sqrt{{\left(x - 0\right)}^{2}+{\left(y-p\right)}^{2}}\hfill \\ =\sqrt{{x}^{2}+{\left(y-p\right)}^{2}}\hfill \end{array}[/latex]
Set the two expressions for [latex]d[/latex] equal to each other and solve for [latex]y[/latex] to derive the equation of the parabola. We do this because the distance from [latex]\left(x,y\right)[/latex] to [latex]\left(0,p\right)[/latex] equals the distance from [latex]\left(x,y\right)[/latex] to [latex]\left(x, -p\right)[/latex]. [latex-display]\sqrt{{x}^{2}+{\left(y-p\right)}^{2}}=y+p[/latex-display] We then square both sides of the equation, expand the squared terms, and simplify by combining like terms.[latex]\begin{array}{c}{x}^{2}+{\left(y-p\right)}^{2}={\left(y+p\right)}^{2}\\ {x}^{2}+{y}^{2}-2py+{p}^{2}={y}^{2}+2py+{p}^{2}\\ {x}^{2}-2py=2py\\ \text{ }{x}^{2}=4py\end{array}[/latex]
The equations of parabolas with vertex [latex]\left(0,0\right)[/latex] are [latex]{y}^{2}=4px[/latex] when the x-axis is the axis of symmetry and [latex]{x}^{2}=4py[/latex] when the y-axis is the axis of symmetry. These standard forms are given below, along with their general graphs and key features.A General Note: Standard Forms of Parabolas with Vertex (0, 0)
The table below summarizes the standard features of parabolas with a vertex at the origin.| Axis of Symmetry | Equation | Focus | Directrix | Endpoints of Focal Diameter |
| x-axis | [latex]{y}^{2}=4px[/latex] | [latex]\left(p,\text{ }0\right)[/latex] | [latex]x=-p[/latex] | [latex]\left(p,\text{ }\pm 2p\right)[/latex] |
| y-axis | [latex]{x}^{2}=4py[/latex] | [latex]\left(0,\text{ }p\right)[/latex] | [latex]y=-p[/latex] | [latex]\left(\pm 2p,\text{ }p\right)[/latex] |
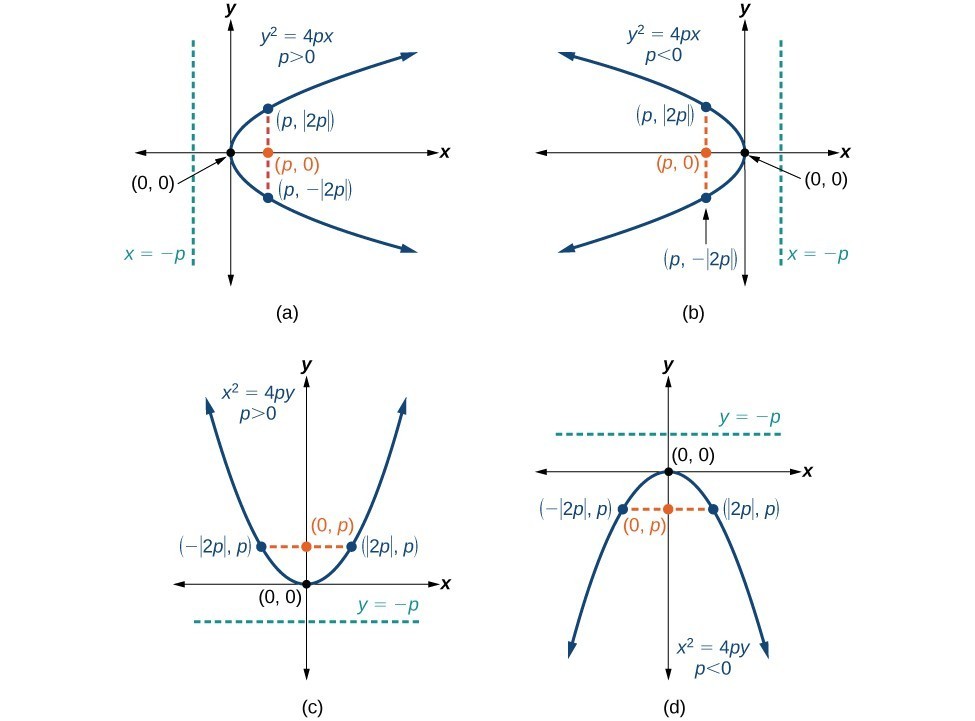 (a) When [latex]p>0[/latex] and the axis of symmetry is the x-axis, the parabola opens right. (b) When [latex]p<0[/latex] and the axis of symmetry is the x-axis, the parabola opens left. (c) When [latex]p<0[/latex] and the axis of symmetry is the y-axis, the parabola opens up. (d) When [latex]\text{ }p<0\text{ }[/latex] and the axis of symmetry is the y-axis, the parabola opens down.
(a) When [latex]p>0[/latex] and the axis of symmetry is the x-axis, the parabola opens right. (b) When [latex]p<0[/latex] and the axis of symmetry is the x-axis, the parabola opens left. (c) When [latex]p<0[/latex] and the axis of symmetry is the y-axis, the parabola opens up. (d) When [latex]\text{ }p<0\text{ }[/latex] and the axis of symmetry is the y-axis, the parabola opens down.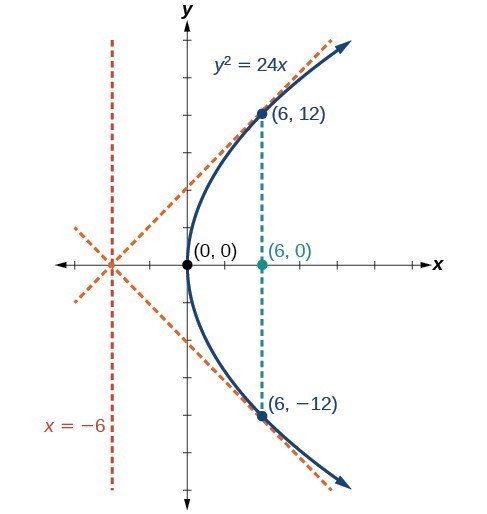
How To: Given a standard form equation for a parabola centered at (0, 0), sketch the graph.
- Determine which of the standard forms applies to the given equation: [latex]{y}^{2}=4px[/latex] or [latex]{x}^{2}=4py[/latex].
- Use the standard form identified in Step 1 to determine the axis of symmetry, focus, equation of the directrix, and endpoints of the focal diameter.
- If the equation is in the form [latex]{y}^{2}=4px[/latex], then
- the axis of symmetry is the x-axis, [latex]y=0[/latex]
- set [latex]4p[/latex] equal to the coefficient of x in the given equation to solve for [latex]p[/latex]. If [latex]p>0[/latex], the parabola opens right. If [latex]p<0[/latex], the parabola opens left.
- use [latex]p[/latex] to find the coordinates of the focus, [latex]\left(p,0\right)[/latex]
- use [latex]p[/latex] to find the equation of the directrix, [latex]x=-p[/latex]
- use [latex]p[/latex] to find the endpoints of the focal diameter, [latex]\left(p,\pm 2p\right)[/latex]. Alternately, substitute [latex]x=p[/latex] into the original equation.
- If the equation is in the form [latex]{x}^{2}=4py[/latex], then
- the axis of symmetry is the y-axis, [latex]x=0[/latex]
- set [latex]4p[/latex] equal to the coefficient of y in the given equation to solve for [latex]p[/latex]. If [latex]p>0[/latex], the parabola opens up. If [latex]p<0[/latex], the parabola opens down.
- use [latex]p[/latex] to find the coordinates of the focus, [latex]\left(0,p\right)[/latex]
- use [latex]p[/latex] to find equation of the directrix, [latex]y=-p[/latex]
- use [latex]p[/latex] to find the endpoints of the focal diameter, [latex]\left(\pm 2p,p\right)[/latex]
- If the equation is in the form [latex]{y}^{2}=4px[/latex], then
- Plot the focus, directrix, and focal diameter, and draw a smooth curve to form the parabola.
Example: Graphing a Parabola with Vertex (0, 0) and the x-axis as the Axis of Symmetry
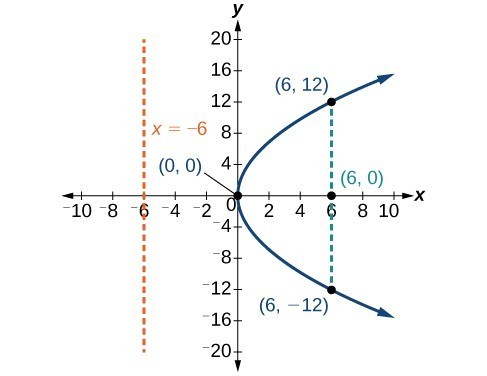
Graph [latex]{y}^{2}=24x[/latex]. Identify and label the focus, directrix, and endpoints of the focal diameter.Answer: The standard form that applies to the given equation is [latex]{y}^{2}=4px[/latex]. Thus, the axis of symmetry is the x-axis. It follows that:
- [latex]24=4p[/latex], so [latex]p=6[/latex]. Since [latex]p>0[/latex], the parabola opens right the coordinates of the focus are [latex]\left(p,0\right)=\left(6,0\right)[/latex]
- the equation of the directrix is [latex]x=-p=-6[/latex]
- the endpoints of the focal diameter have the same x-coordinate at the focus. To find the endpoints, substitute [latex]x=6[/latex] into the original equation: [latex]\left(6,\pm 12\right)[/latex]
Try It
Graph [latex]{y}^{2}=-16x[/latex]. Identify and label the focus, directrix, and endpoints of the focal diameter.Answer:
Focus: [latex]\left(-4,0\right)[/latex]; Directrix: [latex]x=4[/latex]; Endpoints of the latus rectum: [latex]\left(-4,\pm 8\right)[/latex]

Example: Graphing a Parabola with Vertex (0, 0) and the y-axis as the Axis of Symmetry
Graph [latex]{x}^{2}=-6y[/latex]. Identify and label the focus, directrix, and endpoints of the focal diameter.Answer: The standard form that applies to the given equation is [latex]{x}^{2}=4py[/latex]. Thus, the axis of symmetry is the y-axis. It follows that:
- [latex]-6=4p[/latex], so [latex]p=-\frac{3}{2}[/latex]. Since [latex]p<0[/latex], the parabola opens down.
- the coordinates of the focus are [latex]\left(0,p\right)=\left(0,-\frac{3}{2}\right)[/latex]
- the equation of the directrix is [latex]y=-p=\frac{3}{2}[/latex]
- the endpoints of the focal diameter can be found by substituting [latex]\text{ }y=\frac{3}{2}\text{ }[/latex] into the original equation, [latex]\left(\pm 3,-\frac{3}{2}\right)[/latex]

Try It
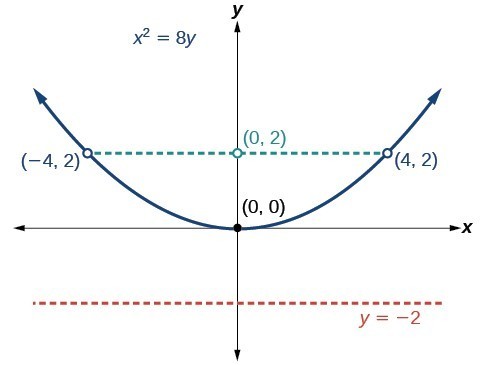
Graph [latex]{x}^{2}=8y[/latex]. Identify and label the focus, directrix, and endpoints of the focal diameter.Answer: Focus: [latex]\left(0,2\right)[/latex]; Directrix: [latex]y=-2[/latex]; Endpoints of the latus rectum: [latex]\left(\pm 4,2\right)[/latex].
Writing Equations of Parabolas in Standard Form
In the previous examples, we used the standard form equation of a parabola to calculate the locations of its key features. We can also use the calculations in reverse to write an equation for a parabola when given its key features.How To: Given its focus and directrix, write the equation for a parabola in standard form.
- Determine whether the axis of symmetry is the x- or y-axis.
- If the given coordinates of the focus have the form [latex]\left(p,0\right)[/latex], then the axis of symmetry is the x-axis. Use the standard form [latex]{y}^{2}=4px[/latex].
- If the given coordinates of the focus have the form [latex]\left(0,p\right)[/latex], then the axis of symmetry is the y-axis. Use the standard form [latex]{x}^{2}=4py[/latex].
- Multiply [latex]4p[/latex].
- Substitute the value from Step 2 into the equation determined in Step 1.
Example: Writing the Equation of a Parabola in Standard Form Given its Focus and Directrix
What is the equation for the parabola with focus [latex]\left(-\frac{1}{2},0\right)[/latex] and directrix [latex]x=\frac{1}{2}?[/latex]Answer: The focus has the form [latex]\left(p,0\right)[/latex], so the equation will have the form [latex]{y}^{2}=4px[/latex]. Multiplying [latex]4p[/latex], we have [latex]4p=4\left(-\frac{1}{2}\right)=-2[/latex]. Substituting for [latex]4p[/latex], we have [latex]{y}^{2}=4px=-2x[/latex]. Therefore, the equation for the parabola is [latex]{y}^{2}=-2x[/latex].
Try It
What is the equation for the parabola with focus [latex]\left(0,\frac{7}{2}\right)[/latex] and directrix [latex]y=-\frac{7}{2}[/latex]?Answer: [latex]{x}^{2}=14y[/latex]
Parabolas with Vertices Not at the Origin
Like other graphs we’ve worked with, the graph of a parabola can be translated. If a parabola is translated [latex]h[/latex] units horizontally and [latex]k[/latex] units vertically, the vertex will be [latex]\left(h,k\right)[/latex]. This translation results in the standard form of the equation we saw previously with [latex]x[/latex] replaced by [latex]\left(x-h\right)[/latex] and [latex]y[/latex] replaced by [latex]\left(y-k\right)[/latex]. To graph parabolas with a vertex [latex]\left(h,k\right)[/latex] other than the origin, we use the standard form [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex] for parabolas that have an axis of symmetry parallel to the x-axis, and [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex] for parabolas that have an axis of symmetry parallel to the y-axis. These standard forms are given below, along with their general graphs and key features.A General Note: Standard Forms of Parabolas with Vertex (h, k)
The table summarizes the standard features of parabolas with a vertex at a point [latex]\left(h,k\right)[/latex].| Axis of Symmetry | Equation | Focus | Directrix | Endpoints of focal diameter |
| [latex]y=k[/latex] | [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex] | [latex]\left(h+p,\text{ }k\right)[/latex] | [latex]x=h-p[/latex] | [latex]\left(h+p,\text{ }k\pm 2p\right)[/latex] |
| [latex]x=h[/latex] | [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex] | [latex]\left(h,\text{ }k+p\right)[/latex] | [latex]y=k-p[/latex] | [latex]\left(h\pm 2p,\text{ }k+p\right)[/latex] |
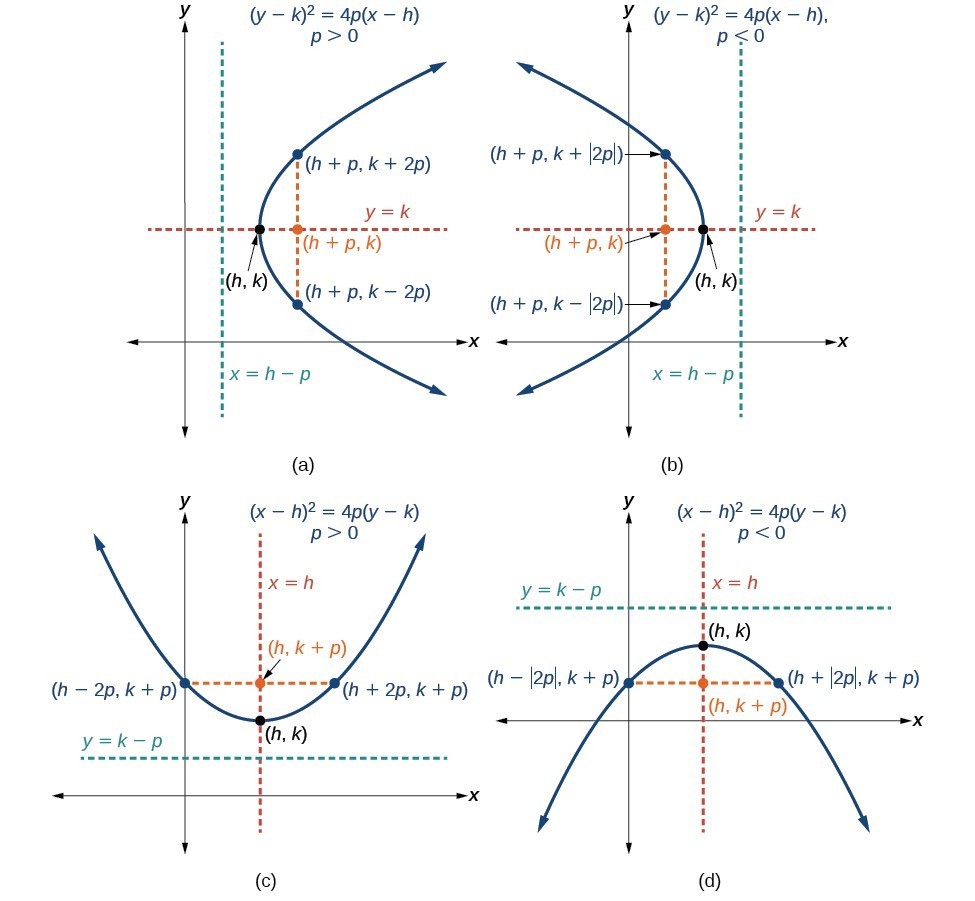 (a) When [latex]p>0[/latex], the parabola opens right. (b) When [latex]p<0[/latex], the parabola opens left. (c) When [latex]p>0[/latex], the parabola opens up. (d) When [latex]p<0[/latex], the parabola opens down.
(a) When [latex]p>0[/latex], the parabola opens right. (b) When [latex]p<0[/latex], the parabola opens left. (c) When [latex]p>0[/latex], the parabola opens up. (d) When [latex]p<0[/latex], the parabola opens down.How To: Given a standard form equation for a parabola centered at (h, k), sketch the graph.
- Determine which of the standard forms applies to the given equation: [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex] or [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex].
- Use the standard form identified in Step 1 to determine the vertex, axis of symmetry, focus, equation of the directrix, and endpoints of the focal diameter.
- If the equation is in the form [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex], then:
- use the given equation to identify [latex]h[/latex] and [latex]k[/latex] for the vertex, [latex]\left(h,k\right)[/latex]
- use the value of [latex]k[/latex] to determine the axis of symmetry, [latex]y=k[/latex]
- set [latex]4p[/latex] equal to the coefficient of [latex]\left(x-h\right)[/latex] in the given equation to solve for [latex]p[/latex]. If [latex]p>0[/latex], the parabola opens right. If [latex]p<0[/latex], the parabola opens left.
- use [latex]h,k[/latex], and [latex]p[/latex] to find the coordinates of the focus, [latex]\left(h+p,\text{ }k\right)[/latex]
- use [latex]h[/latex] and [latex]p[/latex] to find the equation of the directrix, [latex]x=h-p[/latex]
- use [latex]h,k[/latex], and [latex]p[/latex] to find the endpoints of the focal diameter, [latex]\left(h+p,k\pm 2p\right)[/latex]
- If the equation is in the form [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex], then:
- use the given equation to identify [latex]h[/latex] and [latex]k[/latex] for the vertex, [latex]\left(h,k\right)[/latex]
- use the value of [latex]h[/latex] to determine the axis of symmetry, [latex]x=h[/latex]
- set [latex]4p[/latex] equal to the coefficient of [latex]\left(y-k\right)[/latex] in the given equation to solve for [latex]p[/latex]. If [latex]p>0[/latex], the parabola opens up. If [latex]p<0[/latex], the parabola opens down.
- use [latex]h,k[/latex], and [latex]p[/latex] to find the coordinates of the focus, [latex]\left(h,\text{ }k+p\right)[/latex]
- use [latex]k[/latex] and [latex]p[/latex] to find the equation of the directrix, [latex]y=k-p[/latex]
- use [latex]h,k[/latex], and [latex]p[/latex] to find the endpoints of the focal diameter, [latex]\left(h\pm 2p,\text{ }k+p\right)[/latex]
- If the equation is in the form [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex], then:
- Plot the vertex, axis of symmetry, focus, directrix, and focal diameter, and draw a smooth curve to form the parabola.
Example: Graphing a Parabola with Vertex (h, k) and Axis of Symmetry Parallel to the x-axis
Graph [latex]{\left(y - 1\right)}^{2}=-16\left(x+3\right)[/latex]. Identify and label the vertex, axis of symmetry, focus, directrix, and endpoints of the focal diameter.Answer: The standard form that applies to the given equation is [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex]. Thus, the axis of symmetry is parallel to the x-axis. It follows that:
- the vertex is [latex]\left(h,k\right)=\left(-3,1\right)[/latex]
- the axis of symmetry is [latex]y=k=1[/latex]
- [latex]-16=4p[/latex], so [latex]p=-4[/latex]. Since [latex]p<0[/latex], the parabola opens left.
- the coordinates of the focus are [latex]\left(h+p,k\right)=\left(-3+\left(-4\right),1\right)=\left(-7,1\right)[/latex]
- the equation of the directrix is [latex]x=h-p=-3-\left(-4\right)=1[/latex]
- the endpoints of the focal diameter are [latex]\left(h+p,k\pm 2p\right)=\left(-3+\left(-4\right),1\pm 2\left(-4\right)\right)[/latex], or [latex]\left(-7,-7\right)[/latex] and [latex]\left(-7,9\right)[/latex]

Try It
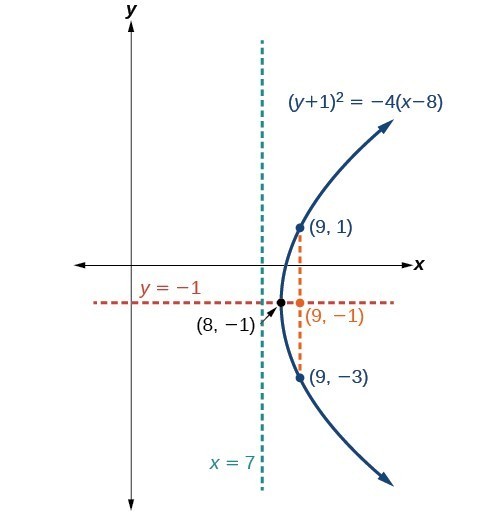
Graph [latex]{\left(y+1\right)}^{2}=4\left(x - 8\right)[/latex]. Identify and label the vertex, axis of symmetry, focus, directrix, and endpoints of the focal diameter.Answer:
Vertex: [latex]\left(8,-1\right)[/latex]; Axis of symmetry: [latex]y=-1[/latex]; Focus: [latex]\left(9,-1\right)[/latex]; Directrix: [latex]x=7[/latex]; Endpoints of the latus rectum: [latex]\left(9,-3\right)[/latex] and [latex]\left(9,1\right)[/latex].
Example: Graphing a Parabola from an Equation Given in General Form
Graph [latex]{x}^{2}-8x - 28y - 208=0[/latex]. Identify and label the vertex, axis of symmetry, focus, directrix, and endpoints of the focal diameter.Answer: Start by writing the equation of the parabola in standard form. The standard form that applies to the given equation is [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex]. Thus, the axis of symmetry is parallel to the y-axis. To express the equation of the parabola in this form, we begin by isolating the terms that contain the variable [latex]x[/latex] in order to complete the square.
[latex]\begin{array}{l}{x}^{2}-8x - 28y - 208=0\hfill \\ \text{ }{x}^{2}-8x=28y+208\hfill \\ \text{ }{x}^{2}-8x+16=28y+208+16\hfill \\ \text{ }{\left(x - 4\right)}^{2}=28y+224\hfill \\ \text{ }{\left(x - 4\right)}^{2}=28\left(y+8\right)\hfill \\ \text{ }{\left(x - 4\right)}^{2}=4\cdot 7\cdot \left(y+8\right)\hfill \end{array}[/latex]
It follows that:- the vertex is [latex]\left(h,k\right)=\left(4,-8\right)[/latex]
- the axis of symmetry is [latex]x=h=4[/latex]
- since [latex]p=7,p>0[/latex] and so the parabola opens up
- the coordinates of the focus are [latex]\left(h,k+p\right)=\left(4,-8+7\right)=\left(4,-1\right)[/latex]
- the equation of the directrix is [latex]y=k-p=-8 - 7=-15[/latex]
- the endpoints of the focal diameter are [latex]\left(h\pm 2p,k+p\right)=\left(4\pm 2\left(7\right),-8+7\right)[/latex], or [latex]\left(-10,-1\right)[/latex] and [latex]\left(18,-1\right)[/latex]

Try It
Graph [latex]{\left(x+2\right)}^{2}=-20\left(y - 3\right)[/latex]. Identify and label the vertex, axis of symmetry, focus, directrix, and endpoints of the focal diameter.Answer: Vertex: [latex]\left(-2,3\right)[/latex]; Axis of symmetry: [latex]x=-2[/latex]; Focus: [latex]\left(-2,-2\right)[/latex]; Directrix: [latex]y=8[/latex]; Endpoints of the latus rectum: [latex]\left(-12,-2\right)[/latex] and [latex]\left(8,-2\right)[/latex].

Solving Applied Problems Involving Parabolas
As we mentioned at the beginning of the section, parabolas are used to design many objects we use every day, such as telescopes, suspension bridges, microphones, and radar equipment. Parabolic mirrors, such as the one used to light the Olympic torch, have a very unique reflecting property. When rays of light parallel to the parabola’s axis of symmetry are directed toward any surface of the mirror, the light is reflected directly to the focus. This is why the Olympic torch is ignited when it is held at the focus of the parabolic mirror. Reflecting property of parabolas
Reflecting property of parabolasExample: Solving Applied Problems Involving Parabolas
A cross-section of a design for a travel-sized solar fire starter. The sun’s rays reflect off the parabolic mirror toward an object attached to the igniter. Because the igniter is located at the focus of the parabola, the reflected rays cause the object to burn in just seconds.- Find the equation of the parabola that models the fire starter. Assume that the vertex of the parabolic mirror is the origin of the coordinate plane.
- Use the equation found in part (a) to find the depth of the fire starter.
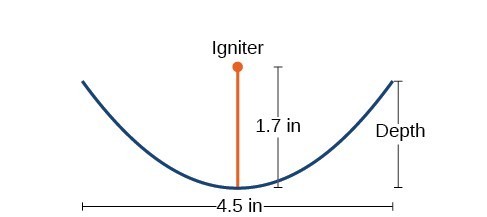 Cross-section of a travel-sized solar fire starter
Cross-section of a travel-sized solar fire starterAnswer:
- The vertex of the dish is the origin of the coordinate plane, so the parabola will take the standard form [latex]{x}^{2}=4py[/latex], where [latex]p>0[/latex]. The igniter, which is the focus, is 1.7 inches above the vertex of the dish. Thus we have [latex]p=1.7[/latex]. [latex]\begin{array}{ll}{x}^{2}=4py\hfill & \begin{array}{cccc}& & & \end{array}\text{Standard form of upward-facing parabola with vertex (0,0)}\hfill \\ {x}^{2}=4\left(1.7\right)y\hfill & \begin{array}{cccc}& & & \end{array}\text{Substitute 1}\text{.7 for }p.\hfill \\ {x}^{2}=6.8y\hfill & \begin{array}{cccc}& & & \end{array}\text{Multiply}.\hfill \end{array}[/latex]
- The dish extends [latex]\frac{4.5}{2}=2.25[/latex] inches on either side of the origin. We can substitute 2.25 for [latex]x[/latex] in the equation from part (a) to find the depth of the dish. [latex-display]\begin{array}{ll}\text{ }{x}^{2}=6.8y\hfill & \text{Equation found in part (a)}.\hfill \\ {\left(2.25\right)}^{2}=6.8y\hfill & \text{Substitute 2}\text{.25 for }x.\hfill \\ \text{ }y\approx 0.74 \hfill & \text{Solve for }y.\hfill \end{array}[/latex-display] The dish is about 0.74 inches deep.
Try It
Balcony-sized solar cookers have been designed for families living in India. The top of a dish has a diameter of 1600 mm. The sun’s rays reflect off the parabolic mirror toward the "cooker," which is placed 320 mm from the base.- Find an equation that models a cross-section of the solar cooker. Assume that the vertex of the parabolic mirror is the origin of the coordinate plane, and that the parabola opens to the right (i.e., has the x-axis as its axis of symmetry).
- Use the equation found in part (a) to find the depth of the cooker.
Answer:
- [latex]{y}^{2}=1280x[/latex]
- The depth of the cooker is 500 mm
Key Equations
| Parabola, vertex at origin, axis of symmetry on x-axis | [latex]{y}^{2}=4px[/latex] |
| Parabola, vertex at origin, axis of symmetry on y-axis | [latex]{x}^{2}=4py[/latex] |
| Parabola, vertex at [latex]\left(h,k\right)[/latex], axis of symmetry on x-axis | [latex]{\left(y-k\right)}^{2}=4p\left(x-h\right)[/latex] |
| Parabola, vertex at [latex]\left(h,k\right)[/latex], axis of symmetry on y-axis | [latex]{\left(x-h\right)}^{2}=4p\left(y-k\right)[/latex] |
Key Concepts
- A parabola is the set of all points [latex]\left(x,y\right)[/latex] in a plane that are the same distance from a fixed line, called the directrix, and a fixed point (the focus) not on the directrix.
- The standard form of a parabola with vertex [latex]\left(0,0\right)[/latex] and the x-axis as its axis of symmetry can be used to graph the parabola. If [latex]p>0[/latex], the parabola opens right. If [latex]p<0[/latex], the parabola opens left.
- The standard form of a parabola with vertex [latex]\left(0,0\right)[/latex] and the y-axis as its axis of symmetry can be used to graph the parabola. If [latex]p>0[/latex], the parabola opens up. If [latex]p<0[/latex], the parabola opens down.
- When given the focus and directrix of a parabola, we can write its equation in standard form.
- The standard form of a parabola with vertex [latex]\left(h,k\right)[/latex] and axis of symmetry parallel to the x-axis can be used to graph the parabola. If [latex]p>0[/latex], the parabola opens right. If [latex]p<0[/latex], the parabola opens left.
- The standard form of a parabola with vertex [latex]\left(h,k\right)[/latex] and axis of symmetry parallel to the y-axis can be used to graph the parabola. If [latex]p>0[/latex], the parabola opens up. If [latex]p<0[/latex], the parabola opens down.
- Real-world situations can be modeled using the standard equations of parabolas. For instance, given the diameter and focus of a cross-section of a parabolic reflector, we can find an equation that models its sides.
Glossary
directrix a line perpendicular to the axis of symmetry of a parabola; a line such that the ratio of the distance between the points on the conic and the focus to the distance to the directrix is constant focus (of a parabola) a fixed point in the interior of a parabola that lies on the axis of symmetry focal diameter (latus rectum) the line segment that passes through the focus of a parabola parallel to the directrix, with endpoints on the parabola parabola the set of all points [latex]\left(x,y\right)[/latex] in a plane that are the same distance from a fixed line, called the directrix, and a fixed point (the focus) not on the directrixLicenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
- Question ID 23511, 86148, 86124, 87082. Authored by: Roy Shahbazian. License: CC BY: Attribution.
- Question ID 23711. Authored by: Caren, McClure. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
CC licensed content, Specific attribution
- Precalculus. Provided by: OpenStax Authored by: OpenStax College. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
