Piecewise Linear Functions*
−x−3[/latex], erasing the part where x is greater than -3. Place an open circle at (-3,0).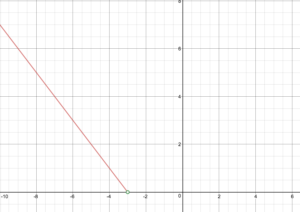 Now place the line on the graph, starting at the point (-3,0). Note that for this portion of the graph, the point (-3,0) is included, so you can remove the open circle.
Now place the line on the graph, starting at the point (-3,0). Note that for this portion of the graph, the point (-3,0) is included, so you can remove the open circle.
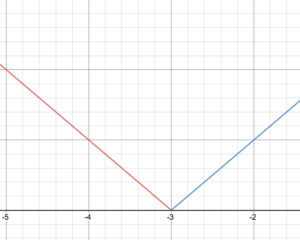 The two graphs meet at the point (-3,0)
The domain of this function is all real numbers because (-3,0)is not included as the endpoint of , but it is included as the endpoint for .
The range of this function starts at and includes 0, and goes to infinity, so we would write this as [/hidden-answer]
In the next example, we will graph a piecewise defined function that models the cost of shipping for an online comic book retailer.
The two graphs meet at the point (-3,0)
The domain of this function is all real numbers because (-3,0)is not included as the endpoint of , but it is included as the endpoint for .
The range of this function starts at and includes 0, and goes to infinity, so we would write this as [/hidden-answer]
In the next example, we will graph a piecewise defined function that models the cost of shipping for an online comic book retailer.
Example
An on-line comic book retailer charges shipping costs according to the following formula Draw a graph of the cost function.Answer: First, draw the line . We can use transformations: this is a vertical stretch of the identity by a factor of 1.5, and a vertical shift by 2.5.
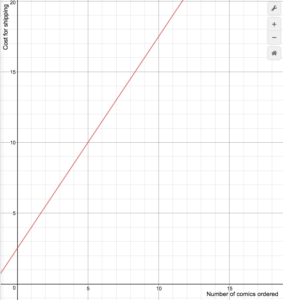 S(n)=1.5n+2.5
S(n)=1.5n+2.5 S(n) = 1.5n+2.5 for 1=n=14
S(n) = 1.5n+2.5 for 1=n=14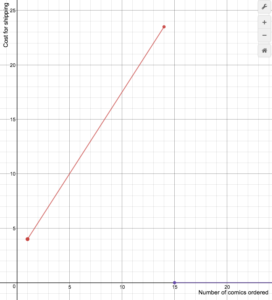
Summary
- A piecewise function is a function in which more than one formula is used to define the output over different pieces of the domain.
- Evaluating a piecewise function means you need to pay close attention to the correct expression used for the given input
