We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

학습 가이드 > ALGEBRA / TRIG I

Problem Set: Decimals

Writing and Identifying Characteristics of Decimals

Name Decimals

In the following exercises, name each decimal.
  1. [latex]5.5[/latex]

    Answer: five and five tenths

  2. [latex]7.8[/latex]
  3. [latex]5.01[/latex]

    Answer: five and one hundredth

  4. [latex]14.02[/latex]
  5. [latex]8.71[/latex]

    Answer: eight and seventy-one hundredths

  6. [latex]2.64[/latex]
  7. [latex]0.002[/latex]

    Answer: two thousandths

  8. [latex]0.005[/latex]
  9. [latex]0.381[/latex]

    Answer: three hundred eighty-one thousandths

  10. [latex]0.479[/latex]
  11. [latex]-17.9[/latex]

    Answer: negative seventeen and nine tenths

  12. [latex]-31.4[/latex]

Write Decimals

In the following exercises, translate the name into a decimal number.
  1. Eight and three hundredths

    Answer: 8.03

  2. Nine and seven hundredths
  3. Twenty-nine and eighty-one hundredths

    Answer: 29.81

  4. Sixty-one and seventy-four hundredths
  5. Seven tenths

    Answer: 0.7

  6. Six tenths
  7. One thousandth

    Answer: 0.001

  8. Nine thousandths
  9. Twenty-nine thousandths

    Answer: 0.029

  10. Thirty-five thousandths
  11. Negative eleven and nine ten-thousandths

    Answer: −11.0009

  12. Negative fifty-nine and two ten-thousandths
  13. Thirteen and three hundred ninety-five ten thousandths

    Answer: 13.0395

  14. Thirty and two hundred seventy-nine thousandths

Convert Decimals to Fractions or Mixed Numbers

In the following exercises, convert each decimal to a fraction or mixed number.
  1. [latex]1.99[/latex]

    Answer: [latex]1\Large\frac{99}{100}[/latex]

  2. [latex]5.83[/latex]
  3. [latex]15.7[/latex]

    Answer: [latex]15\Large\frac{7}{10}[/latex]

  4. [latex]18.1[/latex]
  5. [latex]0.239[/latex]

    Answer: [latex]\Large\frac{239}{1000}[/latex]

  6. [latex]0.373[/latex]
  7. [latex]0.13[/latex]

    Answer: [latex]\Large\frac{13}{100}[/latex]

  8. [latex]0.19[/latex]
  9. [latex]0.011[/latex]

    Answer: [latex]\Large\frac{11}{1000}[/latex]

  10. [latex]0.049[/latex]
  11. [latex]-0.00007[/latex]

    Answer: [latex]-\Large\frac{7}{100000}[/latex]

  12. [latex]-0.00003[/latex]
  13. [latex]6.4[/latex]

    Answer: [latex]6\Large\frac{2}{5}[/latex]

  14. [latex]5.2[/latex]
  15. [latex]7.05[/latex]

    Answer: [latex]7\Large\frac{1}{20}[/latex]

  16. [latex]9.04[/latex]
  17. [latex]4.006[/latex]

    Answer: [latex]4\Large\frac{3}{500}[/latex]

  18. [latex]2.008[/latex]
  19. [latex]10.25[/latex]

    Answer: [latex]10\Large\frac{1}{4}[/latex]

  20. [latex]12.75[/latex]
  21. [latex]1.324[/latex]

    Answer: [latex]1\Large\frac{81}{250}[/latex]

  22. [latex]2.482[/latex]
  23. [latex]14.125[/latex]

    Answer: [latex]14\Large\frac{1}{8}[/latex]

  24. [latex]20.375[/latex]

Locate Decimals on the Number Line

In the following exercises, locate each number on a number line.
  1. [latex]0.8[/latex]

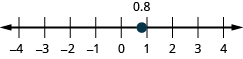
    Answer: There is a number line shown with integers from negative 4 to 4. There is a red dot between 0 and 1 labeled 0.8.

  2. [latex]0.3[/latex]
  3. [latex]-0.2[/latex]

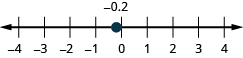
    Answer: There is a number line shown with integers from negative 4 to 4. There is a red dot between negative 1 and 0 labeled negative 0.2.

  4. [latex]-0.9[/latex]
  5. [latex]3.1[/latex]

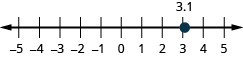
    Answer: This is an image of a number line. It spans from negative 5 on the left to 5 on the right. To the right of 0 are tick marks with the numbers 1, 2, 3, 4, 5 on the number line. To the left of the zero are tick marks with the numbers negative 1, negative 2, negative 3, negative 4, and negative 5. A point is plotted at 3.1.

  6. [latex]2.7[/latex]
  7. [latex]-2.5[/latex]

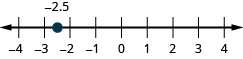
    Answer: There is a number line shown with integers from negative 4 to 4. There is a red dot between negative 3 and negative 2 labeled negative 2.5.

  8. [latex]-1.6[/latex]

Order Decimals

In the following exercises, order each of the following pairs of numbers, using [latex]<\text{or}>[/latex];.
  1. [latex]0.9\text{__}0.6[/latex]

    Answer: >

  2. [latex]0.7\text{__}0.8[/latex]
  3. [latex]0.37\text{__}0.63[/latex]

    Answer: <

  4. [latex]0.86\text{__}0.69[/latex]
  5. [latex]0.6\text{__}0.59[/latex]

    Answer: >

  6. [latex]0.27\text{__}0.3[/latex]
  7. [latex]0.91\text{__}0.901[/latex]

    Answer: >

  8. [latex]0.415\text{__}0.41[/latex]
  9. [latex]-0.5\text{__} - 0.3[/latex]

    Answer: <

  10. [latex]-0.1\text{__} - 0.4[/latex]
  11. [latex]-0.62\text{__} - 0.619[/latex]

    Answer: <

  12. [latex]-7.31\text{__} - 7.3[/latex]

Round Decimals

In the following exercises, round each number to the nearest tenth.
  1. [latex]0.67[/latex]

    Answer: 0.7

  2. [latex]0.49[/latex]
  3. [latex]2.84[/latex]

    Answer: 2.8

  4. [latex]4.63[/latex]
In the following exercises, round each number to the nearest hundredth.
  1. [latex]0.845[/latex]

    Answer: 0.85

  2. [latex]0.761[/latex]
  3. [latex]5.7932[/latex]

    Answer: 5.79

  4. [latex]3.6284[/latex]
  5. [latex]0.299[/latex]

    Answer: 0.30

  6. [latex]0.697[/latex]
  7. [latex]4.098[/latex]

    Answer: 4.10

  8. [latex]7.096[/latex]
In the following exercises, round each number to the nearest ⓐ hundredth ⓑ tenth ⓒ whole number.
  1. [latex]5.781[/latex]

    Answer: ⓐ 5.78 ⓑ 5.8 ⓒ 6

  2. [latex]1.638[/latex]
  3. [latex]63.479[/latex]

    Answer: ⓐ 63.48 ⓑ 63.5 ⓒ 63

  4. [latex]84.281[/latex]

Everyday Math

Salary Increase

Danny got a raise and now makes [latex]{$58,965.95}[/latex] a year. Round this number to the nearest: ⓐ dollar ⓑ thousand dollars ⓒ ten thousand dollars.

Answer: ⓐ $58,966 ⓑ $59,000 ⓒ $60,000

New Car Purchase

Selena’s new car cost [latex]{$23,795.95}[/latex]. Round this number to the nearest:
  1.  dollar
  2. thousand dollars
  3. ten thousand dollars.

Sales Tax

Hyo Jin lives in San Diego. She bought a refrigerator for [latex]{$1624.99}[/latex] and when the clerk calculated the sales tax it came out to exactly [latex]{$142.186625}[/latex]. Round the sales tax to the nearest ⓐ penny ⓑ dollar.

Answer: ⓐ $142.19 ⓑ $142

Sales Tax

Jennifer bought a [latex]{$1,038.99}[/latex] dining room set for her home in Cincinnati. She calculated the sales tax to be exactly [latex]{$67.53435}[/latex]. Round the sales tax to the nearest ⓐ penny ⓑ dollar.

Writing Exercises

How does your knowledge of money help you learn about decimals [practice-area rows="4"][/practice-area] Explain how you write "three and nine hundredths" as a decimal. [practice-area rows="4"][/practice-area] Jim ran a [latex]100-meter[/latex] race in [latex]\text{12.32 seconds}[/latex]. Tim ran the same race in [latex]\text{12.3 seconds}[/latex]. Who had the faster time, Jim or Tim? How do you know? [practice-area rows="4"][/practice-area]

Answer: Tim had the faster time. 12.3 is less than 12.32, so Tim had the faster time.

Gerry saw a sign advertising postcards marked for sale at "10 for 0.99¢"What is wrong with the advertised price? [practice-area rows="4"][/practice-area]

Operations on Decimals

Add and Subtract Decimals

In the following exercises, add or subtract.
  1. [latex]16.92+7.56[/latex]

    Answer: 24.48

  2. [latex]18.37+9.36[/latex]
  3. [latex]256.37 - 85.49[/latex]

    Answer: 170.88

  4. [latex]248.25 - 91.29[/latex]
  5. [latex]21.76 - 30.99[/latex]

    Answer: −9.23

  6. [latex]15.35 - 20.88[/latex]
  7. [latex]37.5+12.23[/latex]

    Answer: 49.73

  8. [latex]38.6+13.67[/latex]
  9. [latex]-16.53 - 24.38[/latex]

    Answer: −40.91

  10. [latex]-19.47 - 32.58[/latex]
  11. [latex]-38.69+31.47[/latex]

    Answer: −7.22

  12. [latex]-29.83+19.76[/latex]
  13. [latex]-4.2+\left(-9.3\right)[/latex]

    Answer: −13.5

  14. [latex]-8.6+\left(-8.6\right)[/latex]
  15. [latex]100 - 64.2[/latex]

    Answer: 35.8

  16. [latex]100 - 65.83[/latex]
  17. [latex]72.5 - 100[/latex]

    Answer: −27.5

  18. [latex]86.2 - 100[/latex]
  19. [latex]15+0.73[/latex]

    Answer: 15.73

  20. [latex]27+0.87[/latex]
  21. [latex]2.51+40[/latex]

    Answer: 42.51

  22. [latex]9.38+60[/latex]
  23. [latex]91.75-\left(-10.462\right)[/latex]

    Answer: 102.212

  24. [latex]94.69-\left(-12.678\right)[/latex]
  25. [latex]55.01 - 3.7[/latex]

    Answer: 51.31

  26. [latex]59.08 - 4.6[/latex]
  27. [latex]2.51 - 7.4[/latex]

    Answer: −4.89

  28. [latex]3.84 - 6.1[/latex]

Multiply Decimals

In the following exercises, multiply.
  1. [latex]\left(0.3\right)\left(0.4\right)[/latex]

    Answer: 0.12

  2. [latex]\left(0.6\right)\left(0.7\right)[/latex]
  3. [latex]\left(0.24\right)\left(0.6\right)[/latex]

    Answer: 0.144

  4. [latex]\left(0.81\right)\left(0.3\right)[/latex]
  5. [latex]\left(5.9\right)\left(7.12\right)[/latex]

    Answer: 42.008

  6. [latex]\left(2.3\right)\left(9.41\right)[/latex]
  7. [latex]\left(8.52\right)\left(3.14\right)[/latex]

    Answer: 26.7528

  8. [latex]\left(5.32\right)\left(4.86\right)[/latex]
  9. [latex]\text{(-4.3)(2.71)}[/latex]

    Answer: −11.653

  10. [latex]\left(-8.5\right)\left(1.69\right)[/latex]
  11. [latex]\text{(-5.18)(-65.23)}[/latex]

    Answer: 337.8914

  12. [latex]\left(-9.16\right)\left(-68.34\right)[/latex]
  13. [latex]\left(0.09\right)\left(24.78\right)[/latex]

    Answer: 2.2302

  14. [latex]\left(0.04\right)\left(36.89\right)[/latex]
  15. [latex]\left(0.06\right)\left(21.75\right)[/latex]

    Answer: 1.305

  16. [latex]\left(0.08\right)\left(52.45\right)[/latex]
  17. [latex]\left(9.24\right)\left(10\right)[/latex]

    Answer: 92.4

  18. [latex]\left(6.531\right)\left(10\right)[/latex]
  19. [latex]\left(55.2\right)\left(1,000\right)[/latex]

    Answer: 55,200

  20. [latex]\left(99.4\right)\left(1,000\right)[/latex]

Divide Decimals

In the following exercises, divide.
  1. [latex]0.15\div 5[/latex]

    Answer: 0.03

  2. [latex]0.27\div 3[/latex]
  3. [latex]4.75\div 25[/latex]

    Answer: 0.19

  4. [latex]12.04\div 43[/latex]
  5. [latex]{$8.49}\div 12[/latex]

    Answer: $0.71

  6. [latex]{$16.99}\div 9[/latex]
  7. [latex]{$117.25}\div 48[/latex]

    Answer: $2.44

  8. [latex]{$109.24}\div 36[/latex]
  9. [latex]0.6\div 0.2[/latex]

    Answer: 3

  10. [latex]0.8\div 0.4[/latex]
  11. [latex]1.44\div \left(-0.3\right)[/latex]

    Answer: −4.8

  12. [latex]1.25\div \left(-0.5\right)[/latex]
  13. [latex]-1.75\div \left(-0.05\right)[/latex]

    Answer: 35

  14. [latex]-1.15\div \left(-0.05\right)[/latex]
  15. [latex]5.2\div 2.5[/latex]

    Answer: 2.08

  16. [latex]6.5\div 3.25[/latex]
  17. [latex]12\div 0.08[/latex]

    Answer: 150

  18. [latex]5\div 0.04[/latex]
  19. [latex]11\div 0.55[/latex]

    Answer: 20

  20. [latex]14\div 0.35[/latex]

Mixed Practice

In the following exercises, simplify.
  1. [latex]6\left(12.4 - 9.2\right)[/latex]

    Answer: 19.2

  2. [latex]3\left(15.7 - 8.6\right)[/latex]
  3. [latex]24\left(0.5\right)+{\left(0.3\right)}^{2}[/latex]

    Answer: 12.09

  4. [latex]35\left(0.2\right)+{\left(0.9\right)}^{2}[/latex]
  5. [latex]1.15\left(26.83+1.61\right)[/latex]

    Answer: 32.706

  6. [latex]1.18\left(46.22+3.71\right)[/latex]
  7. [latex]{$45}+0.08\left({$45}\right)[/latex]

    Answer: $48.60

  8. [latex]{$63}+0.18\left({$63}\right)[/latex]
  9. [latex]18\div \left(0.75+0.15\right)[/latex]

    Answer: 20

  10. [latex]27\div \left(0.55+0.35\right)[/latex]
  11. [latex]\left(1.43+0.27\right)\div \left(0.9 - 0.05\right)[/latex]

    Answer: 2

  12. [latex]\left(1.5 - 0.06\right)\div \left(0.12+0.24\right)[/latex]
  13. [latex]\left[{$75.42}+0.18\left({$75.42}\right)\right]\div 5[/latex]

    Answer: $17.80

  14. [latex]\left[{$56.31}+0.22\left({$56.31}\right)\right]\div 4[/latex]

Use Decimals in Money Applications

In the following exercises, use the strategy for applications to solve.

Spending money

Brenda got [latex]{$40}[/latex] from the ATM. She spent [latex]{$15.11}[/latex] on a pair of earrings. How much money did she have left?

Answer: $24.89

Spending money

Marissa found [latex]{$20}[/latex] in her pocket. She spent [latex]{$4.82}[/latex] on a smoothie. How much of the [latex]{$20}[/latex] did she have left?

Shopping

Adam bought a t-shirt for [latex]{$18.49}[/latex] and a book for [latex]{$8.92}[/latex] The sales tax was [latex]{$1.65}[/latex]. How much did Adam spend?

Answer: $29.06

Restaurant

Roberto’s restaurant bill was [latex]{$20.45}[/latex] for the entrée and [latex]{$3.15}[/latex] for the drink. He left a [latex]{$4.40}[/latex] tip. How much did Roberto spend?

Coupon

Emily bought a box of cereal that cost [latex]{$4.29}[/latex]. She had a coupon for [latex]{$0.55}[/latex] off, and the store doubled the coupon. How much did she pay for the box of cereal?

Answer: $3.19

Coupon

Diana bought a can of coffee that cost [latex]{$7.99}[/latex]. She had a coupon for [latex]{$0.75}[/latex] off, and the store doubled the coupon. How much did she pay for the can of coffee?

Diet

Leo took part in a diet program. He weighed [latex]190[/latex] pounds at the start of the program. During the first week, he lost [latex]4.3[/latex] pounds. During the second week, he had lost [latex]2.8[/latex] pounds. The third week, he gained [latex]0.7[/latex] pounds. The fourth week, he lost [latex]1.9[/latex] pounds. What did Leo weigh at the end of the fourth week?

Answer: 181.7 pounds

Snowpack

On April [latex]1[/latex], the snowpack at the ski resort was [latex]4[/latex] meters deep, but the next few days were very warm. By April [latex]5[/latex], the snow depth was [latex]1.6[/latex] meters less. On April [latex]8[/latex], it snowed and added [latex]2.1[/latex] meters of snow. What was the total depth of the snow?

Coffee

Noriko bought [latex]4[/latex] coffees for herself and her co-workers. Each coffee was [latex]{$3.75}[/latex]. How much did she pay for all the coffees?

Answer: $15.00

Subway Fare

Arianna spends [latex]{$4.50}[/latex] per day on subway fare. Last week she rode the subway [latex]6[/latex] days. How much did she spend for the subway fares?

Income

Mayra earns [latex]{$9.25}[/latex] per hour. Last week she worked [latex]32[/latex] hours. How much did she earn?

Answer: $296.00

Income

Peter earns [latex]{$8.75}[/latex] per hour. Last week he worked [latex]19[/latex] hours. How much did he earn?

Hourly Wage

Alan got his first paycheck from his new job. He worked [latex]30[/latex] hours and earned [latex]{$382.50}[/latex]. How much does he earn per hour?

Answer: $12.75

Hourly Wage

Maria got her first paycheck from her new job. She worked [latex]25[/latex] hours and earned [latex]{$362.50}[/latex]. How much does she earn per hour?

Restaurant

Jeannette and her friends love to order mud pie at their favorite restaurant. They always share just one piece of pie among themselves. With tax and tip, the total cost is [latex]\$6.00[/latex]. How much does each girl pay if the total number sharing the mud pie is
  1. [latex]2?[/latex]

    Answer: $3

  2. [latex]3?[/latex]

    Answer: $2

  3. [latex]4?[/latex]

    Answer: $1.50

  4. [latex]5?[/latex]

    Answer: $1.20

  5. [latex]6?[/latex]

    Answer: $1

Pizza

Alex and his friends go out for pizza and video games once a week. They share the cost of a [latex]\$15.60[/latex] pizza equally. How much does each person pay if the total number sharing the pizza is
  1.  [latex]2?[/latex]
  2. [latex]3?[/latex]
  3. [latex]4?[/latex]
  4. [latex]5?[/latex]
  5.  [latex]6?[/latex]

Fast Food

At their favorite fast food restaurant, the Carlson family orders [latex]4[/latex] burgers that cost [latex]{$3.29}[/latex] each and [latex]2[/latex] orders of fries at [latex]{$2.74}[/latex] each. What is the total cost of the order?

Answer: $18.64

Home Goods

Chelsea needs towels to take with her to college. She buys [latex]2[/latex] bath towels that cost [latex]{$9.99}[/latex] each and [latex]6[/latex] washcloths that cost [latex]{$2.99}[/latex] each. What is the total cost for the bath towels and washcloths?

Zoo

The Lewis and Chousmith families are planning to go to the zoo together. Adult tickets cost [latex]{$29.95}[/latex] and children’s tickets cost [latex]{$19.95}[/latex]. What will the total cost be for [latex]4[/latex] adults and [latex]7[/latex] children?

Answer: $259.45

Ice Skating

Jasmine wants to have her birthday party at the local ice skating rink. It will cost [latex]{$8.25}[/latex] per child and [latex]{$12.95}[/latex] per adult. What will the total cost be for [latex]12[/latex] children and [latex]3[/latex] adults?

Everyday Math

Paycheck

Annie has two jobs. She gets paid [latex]{$14.04}[/latex] per hour for tutoring at City College and [latex]{$8.75}[/latex] per hour at a coffee shop. Last week she tutored for [latex]8[/latex] hours and worked at the coffee shop for [latex]15[/latex] hours.
  1. How much did she earn?

    Answer: $243.57

  2. If she had worked all [latex]23[/latex] hours as a tutor instead of working both jobs, how much more would she have earned?

    Answer: $79.35

Paycheck Jake has two jobs. He gets paid [latex]\$7.95[/latex] per hour at the college cafeteria and [latex]\$20.25[/latex] at the art gallery. Last week he worked [latex]12[/latex] hours at the cafeteria and [latex]5[/latex] hours at the art gallery.
  1.  How much did he earn?
  2. If he had worked all [latex]17[/latex] hours at the art gallery instead of working both jobs, how much more would he have earned?

Writing Exercises

In the 2010 winter Olympics, two skiers took the silver and bronze medals in the Men's Super-G ski event. The silver medalist's time was [latex]1[/latex] minute [latex]30.62[/latex] seconds and bronze medalist's time was [latex]1[/latex] minute [latex]30.65[/latex] seconds. Whose time was faster? Find the difference in their times and then write the name of that decimal. [practice-area rows="4"][/practice-area]

Answer: The difference: 0.03 seconds. Three hundredths of a second.

Find the quotient of [latex]0.12\div 0.04[/latex] and explain in words all the steps taken. [practice-area rows="4"][/practice-area]

Exploring The Relationship Between Decimals and Fractions

Convert Fractions to Decimals

In the following exercises, convert each fraction to a decimal.
  1. [latex]\Large\frac{2}{5}[/latex]

    Answer: 0.4

  2. [latex]\Large\frac{4}{5}[/latex]
  3. [latex]-\Large\frac{3}{8}[/latex]

    Answer: −0.375

  4. [latex]-\Large\frac{5}{8}[/latex]
  5. [latex]\Large\frac{17}{20}[/latex]

    Answer: 0.85

  6. [latex]\Large\frac{13}{20}[/latex]
  7. [latex]\Large\frac{11}{4}[/latex]

    Answer: 2.75

  8. [latex]\Large\frac{17}{4}[/latex]
  9. [latex]-\Large\frac{310}{25}[/latex]

    Answer: −12.4

  10. [latex]-\Large\frac{284}{25}[/latex]
  11. [latex]\Large\frac{5}{9}[/latex]

    Answer: [latex]0.\stackrel{-}{5}[/latex]

  12. [latex]\Large\frac{2}{9}[/latex]
  13. [latex]\Large\frac{15}{11}[/latex]

    Answer: [latex]1.\stackrel{\text{-}}{36}[/latex]

  14. [latex]\Large\frac{18}{11}[/latex]
  15. [latex]\Large\frac{15}{111}[/latex]

    Answer: [latex]0.\stackrel{\text{-}}{135}[/latex]

  16. [latex]\Large\frac{25}{111}[/latex]

Convert Fractions to Decimals and Simplify

In the following exercises, simplify the expression.
  1. [latex]\Large\frac{1}{2}\normalsize+6.5[/latex]

    Answer: 7

  2. [latex]\Large\frac{1}{4}\normalsize+10.75[/latex]
  3. [latex]2.4+\Large\frac{5}{8}[/latex]

    Answer: 3.025

  4. [latex]3.9+\Large\frac{9}{20}[/latex]
  5. [latex]9.73+\Large\frac{17}{20}[/latex]

    Answer: 10.58

  6. [latex]6.29+\Large\frac{21}{40}[/latex]

Order Decimals and Fractions

In the following exercises, order each pair of numbers, using [latex]<[/latex]; or [latex]\text{>.}[/latex]
  1. [latex]\Large\frac{1}{8}\normalsize\text{___}0.8[/latex]

    Answer: <

  2. [latex]\Large\frac{1}{4}\normalsize\text{___}0.4[/latex]
  3. [latex]\Large\frac{2}{5}\normalsize\text{___}0.25[/latex]

    Answer: >

  4. [latex]\Large\frac{3}{5}\normalsize\text{___}0.35[/latex]
  5. [latex]\text {0.725___}\Large\frac{3}{4}[/latex]

    Answer: <

  6. [latex]\text {0.92___}\Large\frac{7}{8}[/latex]
  7. [latex]\text {0.66___}\Large\frac{2}{3}[/latex]

    Answer: <

  8. [latex]\text {0.83___}\Large\frac{5}{6}[/latex]
  9. [latex]\text {-0.75___}-\Large\frac{4}{5}[/latex]

    Answer: >

  10. [latex]\text {-0.44___}-\Large\frac{9}{20}[/latex]
  11. [latex]-\Large\frac{3}{4}\normalsize\text{___} - 0.925[/latex]

    Answer: >

  12. [latex]-\Large\frac{2}{3}\normalsize\text{___} - 0.632[/latex]
In the following exercises, write each set of numbers in order from least to greatest.
  1. [latex]\Large\frac{3}{5}\normalsize ,\Large\frac{9}{16}\normalsize,0.55[/latex]

    Answer: [latex]0.55,\Large\frac{9}{16}\normalsize ,\Large\frac{3}{5}[/latex]

  2. [latex]\Large\frac{3}{8}\normalsize ,\Large\frac{7}{20}\normalsize ,0.36[/latex]
  3. [latex]0.702,\Large\frac{13}{20}\normalsize ,\Large\frac{5}{8}[/latex]

    Answer: [latex]\Large\frac{5}{8}\normalsize ,\Large\frac{13}{20}\normalsize ,0.702[/latex]

  4. [latex]0.15,\Large\frac{3}{16}\normalsize ,\Large\frac{1}{5}[/latex]
  5. [latex]-0.3,-\Large\frac{1}{3}\normalsize ,-\Large\frac{7}{20}[/latex]

    Answer: [latex]-\Large\frac{7}{20}\normalsize ,-\Large\frac{1}{3}\normalsize,-0.3[/latex]

  6. [latex]-0.2,-\Large\frac{3}{20}\normalsize ,-\Large\frac{1}{6}[/latex]
  7. [latex]-\Large\frac{3}{4}\normalsize ,-\Large\frac{7}{9}\normalsize,-0.7[/latex]

    Answer: [latex]-\Large\frac{7}{9}\normalsize ,-\Large\frac{3}{4}\normalsize ,-0.7[/latex]

  8. [latex]-\Large\frac{8}{9}\normalsize ,-\Large\frac{4}{5}\normalsize,-0.9[/latex]

Simplify Expressions Using the Order of Operations

In the following exercises, simplify.
  1. [latex]10\left(25.1 - 43.8\right)[/latex]

    Answer: −187

  2. [latex]30\left(18.1 - 32.5\right)[/latex]
  3. [latex]62\left(9.75 - 4.99\right)[/latex]

    Answer: 295.12

  4. [latex]42\left(8.45 - 5.97\right)[/latex]
  5. [latex]\Large\frac{3}{4}\normalsize\left(12.4 - 4.2\right)[/latex]

    Answer: 6.15

  6. [latex]\Large\frac{4}{5}\normalsize\left(8.6+3.9\right)[/latex]
  7. [latex]\Large\frac{5}{12}\normalsize\left(30.58+17.9\right)[/latex]

    Answer: 20.2

  8. [latex]\Large\frac{9}{16}\normalsize\left(21.96 - 9.8\right)[/latex]
  9. [latex]10\div 0.1+\left(1.8\right)4-{\left(0.3\right)}^{2}[/latex]

    Answer: 107.11

  10. [latex]5\div 0.5+\left(3.9\right)6-{\left(0.7\right)}^{2}[/latex]
  11. [latex]\left(37.1+52.7\right)\div \left(12.5\div 62.5\right)[/latex]

    Answer: 449

  12. [latex]\left(11.4+16.2\right)\div \left(18\div 60\right)[/latex]
  13. [latex]{\Large\left(\frac{1}{5}\right)}^{2}\normalsize+\left(1.4\right)\left(6.5\right)[/latex]

    Answer: 9.14

  14. [latex]{\Large\left(\frac{1}{2}\right)}^{2}\normalsize+\left(2.1\right)\left(8.3\right)[/latex]
  15. [latex]-\Large\frac{9}{10}\normalsize\cdot\Large\frac{8}{15}\normalsize+0.25[/latex]

    Answer: −0.23

  16. [latex]-\Large\frac{3}{8}\normalsize\cdot\Large\frac{14}{15}\normalsize+0.72[/latex]

Mixed Practice

In the following exercises, simplify. Give the answer as a decimal.
  1. [latex]3\Large\frac{1}{4}\normalsize -6.5[/latex]

    Answer: −3.25

  2. [latex]5\Large\frac{2}{5}\normalsize -8.75[/latex]
  3. [latex]10.86\div\Large\frac{2}{3}[/latex]

    Answer: 16.29

  4. [latex]5.79\div\Large\frac{3}{4}[/latex]
  5. [latex]\Large\frac{7}{8}\normalsize\left(103.48\right)+1\Large\frac{1}{2}\normalsize\left(361\right)[/latex]

    Answer: 632.045

  6. [latex]\Large\frac{5}{16}\normalsize\left(117.6\right)+2\Large\frac{1}{3}\normalsize\left(699\right)[/latex]
  7. [latex]3.6\left(\Large\frac{9}{8}\normalsize-2.72\right)[/latex]

    Answer: −5.742

  8. [latex]5.1\left(\Large\frac{12}{5}\normalsize-3.91\right)[/latex]

Find the Circumference and Area of Circles

In the following exercises, approximate the a) circumference and b) area of each circle. If measurements are given in fractions, leave answers in fraction form.
  1. [latex]\text{radius}=\text{5 in.}[/latex]

    Answer: ⓐ 31.4 in ⓑ 78.5 sq.in.

  2. [latex]\text{radius}=\text{20 in.}[/latex]
  3. [latex]\text{radius}=\text{9 ft.}[/latex]

    Answer: ⓐ 56.52.ft. ⓑ 254.34 sq.ft.

  4. [latex]\text{radius}=\text{4 ft.}[/latex]
  5. [latex]\text{radius}=\text{46 cm}[/latex]

    Answer: ⓐ 288.88 cm ⓑ 6644.24 sq.cm

  6. [latex]\text{radius}=\text{38 cm}[/latex]
  7. [latex]\text{radius}=\text{18.6 m}[/latex]

    Answer: ⓐ 116.808 m ⓑ 1086.3144 sq.m

  8. [latex]\text{radius}=\text{57.3 m}[/latex]
  9. [latex]\text{radius}=\Large\frac{7}{10}\normalsize\text{mile}[/latex]

    Answer: ⓐ [latex]\Large\frac{22}{5}\normalsize\text{mile}[/latex] ⓑ [latex]\Large\frac{77}{50}\normalsize\text{sq.mile}[/latex]

  10. [latex]\text{radius}=\Large\frac{7}{11}\normalsize\text{mile}[/latex]
  11. [latex]\text{radius}=\Large\frac{3}{8}\normalsize\text{yard}[/latex]

    Answer: ⓐ [latex]\Large\frac{33}{14}\normalsize\text{yard}[/latex] ⓑ [latex]\Large\frac{99}{224}\normalsize\text{sq.yard}[/latex]

  12. [latex]\text{radius}=\Large\frac{5}{12}\normalsize\text{yard}[/latex]
  13. [latex]\text{diameter}=\Large\frac{5}{6}\normalsize\text{m}[/latex]

    Answer: ⓐ [latex]\Large\frac{55}{21}\normalsize\text{m}[/latex] ⓑ [latex]\Large\frac{275}{504}\normalsize\text{sq.m}[/latex]

  14. [latex]\text{diameter}=\Large\frac{3}{4}\normalsize\text{m}[/latex]

Everyday Math

Shopping Trip

Kelly wants to buy a pair of boots that are on sale for [latex]\Large\frac{2}{3}[/latex] of the original price. The original price of the boots is [latex]{$84.99}[/latex]. What is the sale price of the shoes?

Answer: $56.66

Creating a Mosaic

An architect is planning to put a circular mosaic in the entry of a new building. The mosaic will be in the shape of a circle with radius of [latex]6[/latex] feet. How many square feet of tile will be needed for the mosaic? (Round your answer up to the next whole number.)

Writing Exercises

Is it easier for you to convert a decimal to a fraction or a fraction to a decimal? Explain. [practice-area rows="4"][/practice-area] Describe a situation in your life in which you might need to find the area or circumference of a circle. [practice-area rows="4"][/practice-area]

Solving Equations with Decimals

Determine Whether a Decimal is a Solution of an Equation

In the following exercises, determine whether each number is a solution of the given equation. [latex]x - 0.8=2.3[/latex]

ⓐ [latex]x=2[/latex] ⓑ [latex]x=-1.5[/latex] ⓒ [latex]x=3.1[/latex]

Answer: ⓐ no ⓑ no ⓒ yes

[latex]y+0.6=-3.4[/latex]

ⓐ [latex]y=-4[/latex] ⓑ [latex]y=-2.8[/latex] ⓒ [latex]y=2.6[/latex]

[latex]\Large\frac{h}{1.5}\normalsize =-4.3[/latex]

ⓐ [latex]h=6.45[/latex] ⓑ [latex]h=-6.45[/latex] ⓒ [latex]h=-2.1[/latex]

Answer: ⓐ no ⓑ yes ⓒ no

[latex]0.75k=-3.6[/latex]

ⓐ [latex]k=-0.48[/latex] ⓑ [latex]k=-4.8[/latex] ⓒ [latex]k=-2.7[/latex]

Solve Equations with Decimals

In the following exercises, solve the equation.

  1. [latex]y+2.9=5.7[/latex]

    Answer: y = 2.8

  2. [latex]m+4.6=6.5[/latex]
  3. [latex]f+3.45=2.6[/latex]

    Answer: f = −0.85

  4. [latex]h+4.37=3.5[/latex]
  5. [latex]a+6.2=-1.7[/latex]

    Answer: a = −7.9

  6. [latex]b+5.8=-2.3[/latex]
  7. [latex]c+1.15=-3.5[/latex]

    Answer: c = −4.65

  8. [latex]d+2.35=-4.8[/latex]
  9. [latex]n - 2.6=1.8[/latex]

    Answer: n = 4.4

  10. [latex]p - 3.6=1.7[/latex]
  11. [latex]x - 0.4=-3.9[/latex]

    Answer: x = −3.5

  12. [latex]y - 0.6=-4.5[/latex]
  13. [latex]j - 1.82=-6.5[/latex]

    Answer: j = −4.68

  14. [latex]k - 3.19=-4.6[/latex]
  15. [latex]m - 0.25=-1.67[/latex]

    Answer: m = −1.42

  16. [latex]q - 0.47=-1.53[/latex]
  17. [latex]0.5x=3.5[/latex]

    Answer: x = 7

  18. [latex]0.4p=9.2[/latex]
  19. [latex]-1.7c=8.5[/latex]

    Answer: c = −5

  20. [latex]-2.9x=5.8[/latex]
  21. [latex]-1.4p=-4.2[/latex]

    Answer: p = 3

  22. [latex]-2.8m=-8.4[/latex]
  23. [latex]-120=1.5q[/latex]

    Answer: q = −80

  24. [latex]-75=1.5y[/latex]
  25. [latex]0.24x=4.8[/latex]

    Answer: x = 20

  26. [latex]0.18n=5.4[/latex]
  27. [latex]-3.4z=-9.18[/latex]

    Answer: z = 2.7

  28. [latex]-2.7u=-9.72[/latex]
  29. [latex]\Large\frac{a}{0.4}\normalsize =-20[/latex]

    Answer: a = −8

  30. [latex]\Large\frac{b}{0.3}\normalsize =-9[/latex]
  31. [latex]\Large\frac{x}{0.7}\normalsize =-0.4[/latex]

    Answer: x = −0.28

  32. [latex]\Large\frac{y}{0.8}\normalsize =-0.7[/latex]
  33. [latex]\Large\frac{p}{-5}\normalsize =-1.65[/latex]

    Answer: p = 8.25

  34. [latex]\Large\frac{q}{-4}\normalsize =-5.92[/latex]
  35. [latex]\Large\frac{r}{-1.2}\normalsize =-6[/latex]

    Answer: r = 7.2

  36. [latex]\Large\frac{s}{-1.5}\normalsize =-3[/latex]

Mixed Practice

In the following exercises, solve the equation. Then check your solution.
  1. [latex]x - 5=-11[/latex]

    Answer: x = −6

  2. [latex]-\Large\frac{2}{5}\normalsize =x+\Large\frac{3}{4}[/latex]
  3. [latex]p+8=-2[/latex]

    Answer: p = −10

  4. [latex]p+\Large\frac{2}{3}\normalsize =\Large\frac{1}{12}[/latex]
  5. [latex]-4.2m=-33.6[/latex]

    Answer: m = 8

  6. [latex]q+9.5=-14[/latex]
  7. [latex]q+\Large\frac{5}{6}\normalsize =\Large\frac{1}{12}[/latex]

Answer: [latex]q=-\Large\frac{3}{4}[/latex]

[latex]\Large\frac{8.6}{15}\normalsize =-d[/latex]

[latex]\Large\frac{7}{8}\normalsize m=\Large\frac{1}{10}[/latex]

[latex]m=\Large\frac{4}{35}[/latex]

[latex]\Large\frac{j}{-6.2}\normalsize =-3[/latex]

[latex]-\Large\frac{2}{3}\normalsize =y+\Large\frac{3}{8}[/latex]

[latex]y=-\Large\frac{25}{24}[/latex]

[latex]s - 1.75=-3.2[/latex]

[latex]\Large\frac{11}{20}\normalsize =-f[/latex]

[latex]f=-\Large\frac{11}{20}[/latex]

[latex]-3.6b=2.52[/latex]

[latex]-4.2a=3.36[/latex]

a = −0.8

[latex]-9.1n=-63.7[/latex]

[latex]r - 1.25=-2.7[/latex]

r = −1.45

[latex]\Large\frac{1}{4}\normalsize n=\Large\frac{7}{10}[/latex]

[latex]\Large\frac{h}{-3}\normalsize =-8[/latex]

h = 24

[latex]y - 7.82=-16[/latex]

Translate to an Equation and Solve

In the following exercises, translate and solve.

  1. The difference of [latex]n[/latex] and [latex]1.9[/latex] is [latex]3.4[/latex].

    Answer: [latex]n - 1.9=3.4;5.3[/latex]

  2. The difference [latex]n[/latex] and [latex]1.5[/latex] is [latex]0.8[/latex].
  3. The product of [latex]-6.2[/latex] and [latex]x[/latex] is [latex]-4.96[/latex].

    Answer: −6.2x = −4.96; 0.8

  4. The product of [latex]-4.6[/latex] and [latex]x[/latex] is [latex]-3.22[/latex].
  5. The quotient of [latex]y[/latex] and [latex]-1.7[/latex] is [latex]-5[/latex].

    Answer: [latex]\Large\frac{y}{-1.7}\normalsize =-5;8.5[/latex]

  6. The quotient of [latex]z[/latex] and [latex]-3.6[/latex] is [latex]3[/latex].
  7. The sum of [latex]n[/latex] and [latex]-7.3[/latex] is [latex]2.4[/latex].

    Answer: n + (−7.3) = 2.4; 9.7

  8. The sum of [latex]n[/latex] and [latex]-5.1[/latex] is [latex]3.8[/latex].
 

Everyday math

  1. Shawn bought a pair of shoes on sale for [latex]$78[/latex] . Solve the equation [latex]0.75p=78[/latex] to find the original price of the shoes, [latex]p[/latex].

    Answer: $104

  2. Mary bought a new refrigerator. The total price including sales tax was [latex]{$1,350}[/latex]. Find the retail price, [latex]r[/latex], of the refrigerator before tax by solving the equation [latex]1.08r=1,350[/latex].
 

Writing Exercises

  • Think about solving the equation [latex]1.2y=60[/latex], but do not actually solve it. Do you think the solution should be greater than [latex]60[/latex] or less than [latex]60?[/latex] Explain your reasoning. Then solve the equation to see if your thinking was correct. Answers will vary.
  • Think about solving the equation [latex]0.8x=200[/latex], but do not actually solve it. Do you think the solution should be greater than [latex]200[/latex] or less than [latex]200?[/latex] Explain your reasoning. Then solve the equation to see if your thinking was correct.

Chapter Review Exercises

Decimals

Name Decimals

In the following exercises, name each decimal.
  1. [latex]0.8[/latex]
  2. [latex]0.375[/latex]

    Answer: three hundred seventy-five thousandths

  3. [latex]0.007[/latex]
  4. [latex]5.24[/latex]

    Answer: five and twenty-four hundredths

  5. [latex]-12.5632[/latex]
  6. [latex]-4.09[/latex]

    Answer: negative four and nine hundredths

Write Decimals In the following exercises, write as a decimal.
  1. three tenths
  2. nine hundredths

    Answer: 0.09

  3. twenty-seven hundredths
  4. ten and thirty-five thousandths

    Answer: 10.035

  5. negative twenty and three tenths
  6. negative five hundredths

    Answer: −0.05

Convert Decimals to Fractions or Mixed Numbers

In the following exercises, convert each decimal to a fraction. Simplify the answer if possible.
  1. [latex]0.43[/latex]
  2. [latex]0.825[/latex]

    Answer: [latex]\Large\frac{33}{40}[/latex]

  3. [latex]9.7[/latex]
  4. [latex]3.64[/latex]

    Answer: [latex]3\Large\frac{16}{25}[/latex]

Locate Decimals on the Number Line

  1. [latex]0.6[/latex]
  2. [latex]-0.9[/latex]
  3. [latex]2.2[/latex]
  4. [latex]-1.3[/latex]
Order Decimals In the following exercises, order each of the following pairs of numbers, using [latex]<[/latex]; or [latex]\text{>.}[/latex]
  1. [latex]0.6\text{___}0.8[/latex]

    Answer: <

  2. [latex]0.2\text{___}0.15[/latex]
  3. [latex]0.803\text{____}0.83[/latex]

    Answer: <

  4. [latex]-0.56\text{____} - 0.562[/latex]
Round Decimals In the following exercises, round each number to the nearest: ⓐ hundredth ⓑ tenth ⓒ whole number.
  1. [latex]12.529[/latex]

    Answer: ⓐ 12.53 ⓑ 12.5 ⓒ 13

  2. [latex]4.8447[/latex]
  3. [latex]5.897[/latex]

    Answer: ⓐ 5.90 ⓑ 5.9 ⓒ 6

Decimal Operations

Add and Subtract Decimals In the following exercises, add or subtract.
  1. [latex]5.75+8.46[/latex]
  2. [latex]32.89 - 8.22[/latex]

    Answer: 24.67

  3. [latex]24 - 19.31[/latex]
  4. [latex]10.2+14.631[/latex]

    Answer: 24.831

  5. [latex]-6.4+\left(-2.9\right)[/latex]
  6. [latex]1.83 - 4.2[/latex]

    Answer: −2.37

Multiply Decimals

In the following exercises, multiply.
  1. [latex]\left(0.3\right)\left(0.7\right)[/latex]
  2. [latex]\left(-6.4\right)\left(0.25\right)[/latex]

    Answer: −1.6

  3. [latex]\left(-3.35\right)\left(-12.7\right)[/latex]
  4. [latex]\left(15.4\right)\left(1000\right)[/latex]

    Answer: 15,400

Divide Decimals

In the following exercises, divide.
  1. [latex]0.48\div 6[/latex]
  2. [latex]4.32\div 24[/latex]

    Answer: 0.18

  3. [latex]{$6.29}\div 12[/latex]
  4. [latex]\left(-0.8\right)\div \left(-0.2\right)[/latex]

    Answer: 4

  5. [latex]1.65\div 0.15[/latex]
  6. [latex]9\div 0.045[/latex]

    Answer: 200

Use Decimals in Money Applications

In the following exercises, use the strategy for applications to solve.
  1. Miranda got [latex]{$40}[/latex] from her ATM. She spent [latex]{$9.32}[/latex] on lunch and [latex]{$16.99}[/latex] on a book. How much money did she have left? Round to the nearest cent if necessary.
  2. Jessie put [latex]8[/latex] gallons of gas in her car. One gallon of gas costs [latex]{$3.528.}[/latex] How much did Jessie owe for all the gas?

    Answer: $28.22

  3. A pack of [latex]16[/latex] water bottles cost [latex]{$6.72.}[/latex] How much did each bottle cost?
  4. Alice bought a roll of paper towels that cost [latex]{$2.49.}[/latex] She had a coupon for [latex]{$0.35}[/latex] off, and the store doubled the coupon. How much did Alice pay for the paper towels?

    Answer: $1.79

Decimals and Fractions

Convert Fractions to Decimals In the following exercises, convert each fraction to a decimal.
  1. [latex]\Large\frac{3}{5}[/latex]
  2. [latex]\Large\frac{7}{8}[/latex]

    Answer: 0.875

  3. [latex]-\Large\frac{19}{20}[/latex]
  4. [latex]-\Large\frac{21}{4}[/latex]

    Answer: −5.25

  5. [latex]\Large\frac{1}{3}[/latex]
  6. [latex]\Large\frac{6}{11}[/latex]

    Answer: [latex]0.\stackrel{\text{-}}{54}[/latex]

Order Decimals and Fractions

In the following exercises, order each pair of numbers, using [latex]<[/latex]; or [latex]\text{>.}[/latex]
  1. [latex]\Large\frac{1}{2}\text{___}\normalsize 0.2[/latex]
  2. [latex]\Large\frac{3}{5}\text{___}\normalsize 0[/latex].

    Answer: >

  3. [latex]-\Large\frac{7}{8}\text{___}\normalsize - 0.84[/latex]
  4. [latex]-\Large\frac{5}{12}\text{___}\normalsize - 0.42[/latex]

    Answer: >

  5. [latex]0.625\text{___}\Large\frac{13}{20}[/latex]
  6. [latex]0.33\text{___}\Large\frac{5}{16}[/latex]

    Answer: >

In the following exercises, write each set of numbers in order from least to greatest.
  1. [latex]\Large\frac{2}{3}\normalsize ,\Large\frac{17}{20}\normalsize ,0.65[/latex]
  2. [latex]\Large\frac{7}{9}\normalsize ,0.75\normalsize ,\Large\frac{11}{15}[/latex]

    Answer: [latex]\Large\frac{11}{15}\normalsize ,0.75,\Large\frac{7}{9}[/latex]

Simplify Expressions Using the Order of Operations In the following exercises, simplify
  1. [latex]4\left(10.3 - 5.8\right)[/latex]
  2. [latex]\Large\frac{3}{4}\normalsize\left(15.44 - 7.4\right)[/latex]

    Answer: 6.03

  3. [latex]30\div \left(0.45+0.15\right)[/latex]
  4. [latex]1.6+\Large\frac{3}{8}[/latex]

    Answer: 1.975

  5. [latex]52\left(0.5\right)+{\left(0.4\right)}^{2}[/latex]
  6. [latex]-\Large\frac{2}{5}\normalsize\cdot\Large\frac{9}{10}\normalsize +0.14[/latex]

    Answer: −0.22

Find the Circumference and Area of Circles

In the following exercises, approximate the ⓐ circumference and ⓑ area of each circle.
  1. [latex]\text{radius}=\text{6 in.}[/latex]
  2. [latex]\text{radius}=\text{3.5 ft.}[/latex]

    Answer: ⓐ 21.98 ft. ⓑ 38.465 sq.ft.

  3. [latex]\text{radius}=\Large\frac{7}{33}\normalsize\text{m}[/latex]
  4. [latex]\text{diameter}=\text{11 cm}[/latex]

    Answer: ⓐ 34.54 cm ⓑ 379.94 sq.cm

Chapter Practice Test

  1. Write six and thirty-four thousandths as a decimal.
  2. Write [latex]1.73[/latex] as a fraction.

    Answer: [latex]1\Large\frac{73}{100}[/latex]

  3. Write [latex]\Large\frac{5}{8}[/latex] as a decimal.
  4. Round [latex]16.749[/latex] to the nearest ⓐ tenth ⓑ hundredth ⓒ whole number

    Answer: ⓐ 16.7 ⓑ 16.75 ⓒ 17

  5. Write the numbers [latex]\Large\frac{4}{5}\normalsize ,-0.1,0.804,\Large\frac{2}{9}\normalsize ,-7.4,0.21[/latex] in order from smallest to largest.
In the following exercises, simplify each expression.
  1. [latex]15.4+3.02[/latex]

    Answer: 18.42

  2. [latex]20 - 5.71[/latex]
  3. [latex]\left(0.64\right)\left(0.3\right)[/latex]

    Answer: 0.192

  4. [latex]\left(-4.2\right)\left(100\right)[/latex]
  5. [latex]0.96\div \left(-12\right)[/latex]

    Answer: −0.08

  6. [latex]-5\div 0.025[/latex]
  7. [latex]-0.6\div \left(-0.3\right)[/latex]

    Answer: 2

  8. [latex]{\left(0.7\right)}^{2}[/latex]
  9. [latex]24\div \left(0.1+0.02\right)[/latex]

    Answer: 200

  10. [latex]4\left(10.3 - 5.8\right)[/latex]
  11. [latex]1.6+\Large\frac{3}{8}[/latex]

    Answer: 1.975

  12. [latex]\Large\frac{2}{3}\normalsize\left(14.65 - 4.6\right)[/latex]
  In the following exercises, solve.
  1. [latex]m+3.7=2.5[/latex]

    Answer: −1.2

  2. [latex]\Large\frac{h}{0.5}\normalsize =4.38[/latex]
  3. [latex]-6.5y=-57.2[/latex]

    Answer: 8.8

  4. [latex]1.94=a - 2.6[/latex]
  5. Three friends went out to dinner and agreed to split the bill evenly. The bill was [latex]{$79.35}[/latex]. How much should each person pay?

    Answer: $26.45

  6. A circle has radius [latex]12[/latex]. Find the ⓐ circumference and ⓑ area. [latex]\text{[}\text{Use}3.14\text{for}\pi \text{.]}[/latex]
  7. The ages, in months, of [latex]10[/latex] children in a preschool class are:[latex]55[/latex] , [latex]55[/latex] , [latex]50[/latex] , [latex]51[/latex] , [latex]52[/latex] , [latex]50[/latex] , [latex]53[/latex] , [latex]51[/latex] , [latex]55[/latex] , [latex]49[/latex]Find the ⓐ mean ⓑ median ⓒ mode

    Answer: ⓐ 59 ⓑ 51.5 ⓒ 55

  8. Of the [latex]16[/latex] nurses in Doreen’s department, [latex]12[/latex] are women and [latex]4[/latex] are men. One of the nurses will be assigned at random to work an extra shift next week. ⓐ Find the probability a woman nurse will be assigned the extra shift. ⓑ Convert the fraction to a decimal.
Find each unit price and then the better buy. Laundry detergent: [latex]64[/latex] ounces for [latex]{$10.99}[/latex] or [latex]48[/latex] ounces for [latex]{$8.49}[/latex]

Answer: 64 ounces for $10.99 is the better buy

  In the following exercises, simplify.
  1. [latex]\sqrt{36+64}[/latex]
  2. [latex]\sqrt{144{n}^{2}}[/latex]

    Answer: 12n

  3. Estimate [latex]\sqrt{54}[/latex] to between two whole numbers.
  4. Yanet wants a square patio in her backyard. She has [latex]225[/latex] square feet of tile. How long can a side of the patio be?

    Answer: 15 feet

 
 

Contribute!

Did you have an idea for improving this content? We’d love your input.

Licenses & Attributions

CC licensed content, Specific attribution