We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > ALGEBRA / TRIG I

Graphing a Line Using Slope and a Point

Learning Outcomes

  • Draw the graph of a line using slope and a point on the line
  • Write the equation of a line using slope and y-intercept
When graphing a line we found one method we could use is to make a table of values.  We also learned how to graph lines by plotting points, by using intercepts, and by recognizing horizontal and vertical lines.  However, if we can identify some properties of the line, we may be able to make a graph much quicker and easier.

Graphing a Line Using Slope and a Point on the Line

Another method we can use to graph lines is the point-slope method. Sometimes, we will be given one point and the slope of the line, instead of its equation. When this happens, we use the definition of slope to draw the graph of the line.

example

Graph the line passing through the point [latex]\left(1,-1\right)[/latex] whose slope is [latex]m=\Large\frac{3}{4}[/latex]. Solution Plot the given point, [latex]\left(1,-1\right)[/latex]. The graph shows the x y-coordinate plane. The x-axis runs from -1 to 7. The y-axis runs from -3 to 4. A labeled point is drawn at Use the slope formula [latex]m=\Large\frac{\text{rise}}{\text{run}}[/latex] to identify the rise and the run.

[latex]\begin{array}{}\\ \\ m=\frac{3}{4}\hfill \\ \frac{\text{rise}}{\text{run}}=\frac{3}{4}\hfill \\ \\ \\ \text{rise}=3\hfill \\ \text{run}=4\hfill \end{array}[/latex]

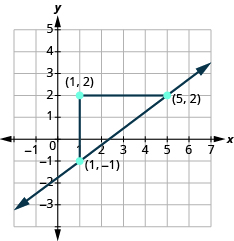Starting at the point we plotted, count out the rise and run to mark the second point. We count [latex]3[/latex] units up and [latex]4[/latex] units right. The graph shows the x y-coordinate plane. Both axes run from -5 to 5. Two line segments are drawn. A vertical line segment connects the points Then we connect the points with a line and draw arrows at the ends to show it continues. The graph shows the x y-coordinate plane. The x-axis runs from -3 to 5. The y-axis runs from -1 to 7. Two unlabeled points are drawn at We can check our line by starting at any point and counting up [latex]3[/latex] and to the right [latex]4[/latex]. We should get to another point on the line.
 

try it

[ohm_question]147024[/ohm_question]

Graph a line given a point and a slope

    1. Plot the given point.
    2. Use the slope formula to identify the rise and the run.
    3. Starting at the given point, count out the rise and run to mark the second point.
    4. Connect the points with a line.
 

example

Graph the line passing through the point [latex]\left(-1,-3\right)[/latex] whose slope is [latex]m=4[/latex]

Answer: Solution Plot the given point. The graph shows the x y-coordinate plane. Both axes run from -5 to 5. The point

Identify the rise and the run. [latex]m=4[/latex]
Write [latex]4[/latex] as a fraction. [latex]\Large\frac{\text{rise}}{\text{run}}\normalsize=\Large\frac{4}{1}[/latex]
[latex]\text{rise}=4\text{ run}=1[/latex]
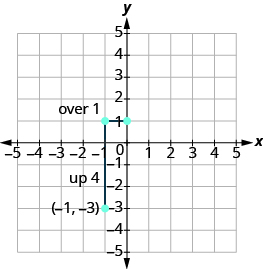Count the rise and run. The graph shows the x y-coordinate plane. Both axes run from -5 to 5. The y-axis runs from -4 to 2. A vertical line segment connects points at Mark the second point. Connect the two points with a line. The graph shows the x y-coordinate plane. Both axes run from -5 to 5. Two labeled points are drawn at

 

try it

[ohm_question]147026[/ohm_question]
You can watch the video below for another example of how to graph a line given a point and a slope. https://youtu.be/ngpAgpMjozw A special case for graphing using the point-slope method is when the given point is the y-intercept. We will give some examples here and then we will show below why this case is so important.

example

Graph the line with [latex]y[/latex] -intercept [latex]\left(0,2\right)[/latex] and slope [latex]m=-\Large\frac{2}{3}[/latex]

Answer: Solution Plot the given point, the [latex]y[/latex] -intercept [latex]\left(0,2\right)[/latex]. The graph shows the x y-coordinate plane. The x-axis runs from -1 to 4. The y-axis runs from -1 to 3. The point Use the slope formula [latex]m=\Large\frac{\text{rise}}{\text{run}}[/latex] to identify the rise and the run.

[latex]\begin{array}{}\\ \\ m=-\frac{2}{3}\hfill \\ \frac{\text{rise}}{\text{run}}=\frac{-2}{3}\hfill \\ \\ \\ \text{rise}=-2\hfill \\ \text{run}=3\hfill \end{array}[/latex]

Starting at [latex]\left(0,2\right)[/latex], count the rise and the run and mark the second point.

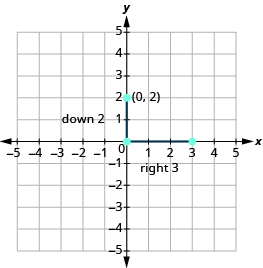The graph shows the x y-coordinate plane. Both axes run from -5 to 5. A vertical line segment connects points at Connect the points with a line. The graph shows the x y-coordinate plane. Both axes run from -5 to 5. Two labeled points are drawn at

 

try it

[ohm_question]147025[/ohm_question]
 

Slope-Intercept Form

We will now show you what is so special about the case in which the given point is the y-intercept.  The slope can be represented by m and the y-intercept, where it crosses the axis and [latex]x=0[/latex], can be represented by [latex](0,b)[/latex] where b is the value where the graph crosses the vertical y-axis. Any other point on the line can be represented by [latex](x,y)[/latex].

Slope-Intercept Form of a Linear Equation

In the equation [latex]y=mx+b[/latex],
  • m is the slope of the graph.
  • b is the y value of the y-intercept of the graph.

This formula is known as the slope-intercept equation. If we know the slope and the y-intercept we can easily find the equation that represents the line.

Example

Write the equation of the line that has a slope of [latex] \displaystyle \frac{1}{2}[/latex] and a y-intercept of [latex]−5[/latex].

Answer: Substitute the slope (m) into [latex]y=mx+b[/latex].

[latex] \displaystyle y=\frac{1}{2}x+b[/latex]

Substitute the [latex]y[/latex]-intercept (b) into the equation.

[latex] \displaystyle y=\frac{1}{2}x-5[/latex]

Answer

[latex-display]y=\frac{1}{2}x-5[/latex-display]

We can also easily find the equation by looking at a graph and finding the slope and y-intercept.

Example

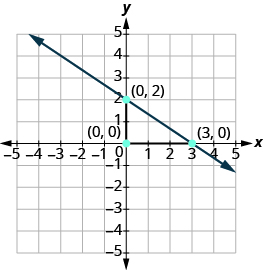
Write the equation of the line in the graph by identifying the slope and y-intercept.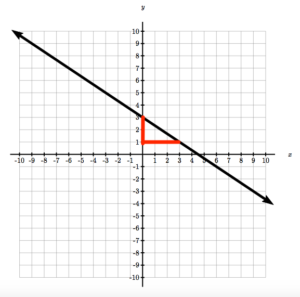 SVG_Grapher

Answer: Identify the point where the graph crosses the y-axis [latex](0,3)[/latex]. This means the [latex]y[/latex]-intercept is [latex]3[/latex]. Identify one other point and draw a slope triangle to find the slope. The slope is [latex]\frac{-2}{3}[/latex] Substitute the slope and y value of the intercept into the slope-intercept equation.

[latex]y=mx+b\\y=\frac{-2}{3}x+b\\y=\frac{-2}{3}x+3[/latex]

Answer

[latex-display]y=\frac{-2}{3}x+3[/latex-display]

We can also move in the opposite direction.  When we are given an equation in the slope-intercept form of [latex]y=mx+b[/latex], we can easily identify the slope and y-intercept and graph the equation from this information.    When we have an equation in slope-intercept form we can graph it by first plotting the y-intercept, then using the slope, find a second point and connecting the dots.

Example

Graph [latex]y=\frac{1}{2}x-4[/latex] using the slope-intercept equation.

Answer: First, plot the 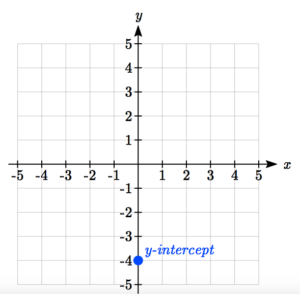[latex]y[/latex]-intercept. The y-intercept plotted at negative 4 on the y axis. Now use the slope to count up or down and over left or right to the next point. This slope is [latex]\frac{1}{2}[/latex], so you can count up one and right two—both positive because both parts of the slope are positive. Connect the dots. A line crosses through negative 4 on the y-axis and has a slope of 1/2.

(NOTE: it is important for the equation to first be in slope intercept form. If it is not, we will have to solve it for [latex]y[/latex] so we can identify the slope and the [latex]y[/latex]-intercept.)

Try It

[ohm_question]79774[/ohm_question]
You can watch the video below for another example of how to write the equation of the line, when given a graph, by identifying the slope and y-intercept. https://youtu.be/GIn7vbB5AYo  

Contribute!

Did you have an idea for improving this content? We’d love your input.

Licenses & Attributions

CC licensed content, Shared previously

CC licensed content, Specific attribution

  • Beginning and Intermediate Algebra. Authored by: Tyler Wallace. License: CC BY: Attribution.