Classify Solutions for Systems
Learning Outcomes
- Classify solutions for systems
Use a graph to classify solutions to systems
Recall that a linear equation graphs as a line, which indicates that all of the points on the line are solutions to that linear equation. There are an infinite number of solutions. As we saw in the last section, if you have a system of linear equations that intersect at one point, this point is a solution to the system. What happens if the lines never cross, as in the case of parallel lines? How would you describe the solutions to that kind of system? In this section, we will explore the three possible outcomes for solutions to a system of linear equations.Three possible outcomes for solutions to systems of equations
Recall that the solution for a system of equations is the value or values that are true for all equations in the system. There are three possible outcomes for solutions to systems of linear equations. The graphs of equations within a system can tell you how many solutions exist for that system. Look at the images below. Each shows two lines that make up a system of equations.| One Solution | No Solutions | Infinite Solutions |
|---|---|---|
 |
 |
 |
| If the graphs of the equations intersect, then there is one solution that is true for both equations. | If the graphs of the equations do not intersect (for example, if they are parallel), then there are no solutions that are true for both equations. | If the graphs of the equations are the same, then there are an infinite number of solutions that are true for both equations. |
- One Solution: When a system of equations intersects at an ordered pair, the system has one solution.
- Infinite Solutions: Sometimes the two equations will graph as the same line, in which case we have an infinite number of solutions.
- No Solution: When the lines that make up a system are parallel, there are no solutions because the two lines share no points in common.
Example
Using the graph of , shown below, determine how many solutions the system has.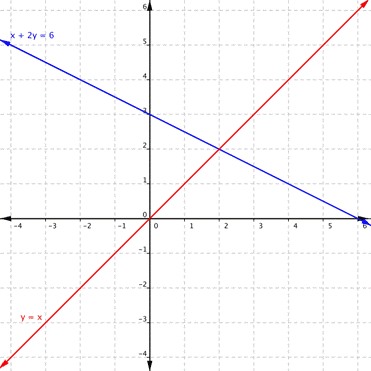
Answer: The lines intersect at one point. So the two lines have only one point in common, there is only one solution to the system.
Answer
There is one solution to this system.Example (Advanced)
Using the graph of , shown below, determine how many solutions the system has.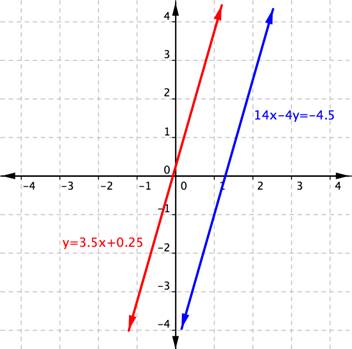
Answer: The lines are parallel, meaning they do not intersect. There are no solutions to the system.
Answer
There are no solutions to the system.Example
How many solutions does the system have?Answer:
First, graph both equations on the same axes.
 The two equations graph as the same line. So every point on that line is a solution for the system of equations.
The two equations graph as the same line. So every point on that line is a solution for the system of equations.
Answer
The system has an infinite number of solutions.A General Note: Types of Linear Systems
There are three types of systems of linear equations in two variables and three types of solutions.- An independent system has exactly one solution pair . The point where the two lines intersect is the only solution.
- An inconsistent system has no solution.The two lines are parallel and will never intersect.
- A dependent system has infinitely many solutions. The lines are coincident. They are the same line, so every coordinate pair on the line is a solution to both equations.
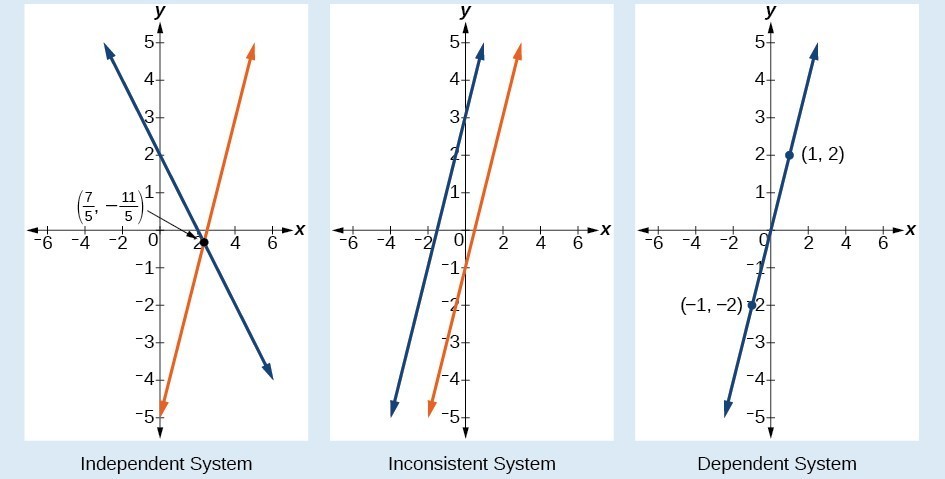 The independent and dependent systems are also consistent because they both have at least one solution.
The independent and dependent systems are also consistent because they both have at least one solution.
Contribute!
Did you have an idea for improving this content? We’d love your input.
