Systems of Three Equations in Three Variables
Learning Outcomes
- Solve systems of three equations in three variables
Solution Set, One Solution
The figure below illustrates how a system with three variables can have one solution. Systems that have a single solution are those which result in a solution set consisting of an ordered triple . Graphically, the ordered triple defines a point that is the intersection of three planes in space.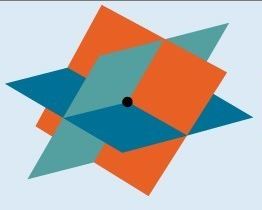
Example
Determine whether the ordered triple is a solution to the system.Answer: We will check each equation by substituting in the values of the ordered triple for , and . The ordered triple is indeed a solution to the system.
How To: Given a linear system of three equations, solve for three unknowns
- Pick any pair of equations and solve for one variable.
- Pick another pair of equations and solve for the same variable.
- You have created a system of two equations in two unknowns. Solve the resulting two-by-two system.
- Back-substitute known variables into any one of the original equations and solve for the missing variable.
Example
Solve the given system.Answer: The third equation states that , so we substitute this into the second equation to obtain a solution for . Now we have two of our solutions, and we can substitute them both into the first equation to solve for . Now we have our ordered triple; remember that where you place the solutions matters!
Analysis of the Solution:
Each of the lines in the system above represents a plane (think about a sheet of paper). If you imagine three sheets of notebook paper each representing a portion of these planes, you will start to see the complexities involved in how three such planes can intersect. Below is a sketch of the three planes. It turns out that any two of these planes intersect in a line, so our intersection point is where all three of these lines meet.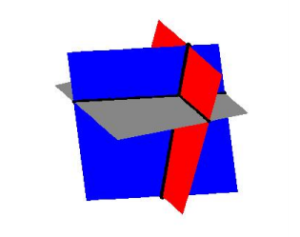 Three planes intersecting.
Three planes intersecting.Example
Find a solution to the following system:Answer: We labeled the equations this time to be able to keep track of things a little more easily. The most obvious first step here is to eliminate by adding equations (2) and (3).
Now we can substitute the value for that we obtained into equation .
Be careful here not to get confused with a solution of and an inconsistent solution. It is ok for variables to equal . Now we can substitute and back into the first equation.
Try It
[ohm_question]38331[/ohm_question]Summary
- The solution to a system of linear equations in three variables is an ordered triple of the form .
- Solutions can be verified using substitution and the order of operations.
- Systems of three variables can be solved using the same techniques as we used to solve systems with two variables, including elimination and substitution.
Contribute!
Did you have an idea for improving this content? We’d love your input.
