Absolute Value Functions
Learning Objectives
By the end of this lesson, you will be able to:- Graph an absolute value function.
- Find the intercepts of an absolute value function
 Distances in deep space can be measured in all directions. As such, it is useful to consider distance in terms of absolute values. (credit: "s58y"/Flickr)
Distances in deep space can be measured in all directions. As such, it is useful to consider distance in terms of absolute values. (credit: "s58y"/Flickr)Understanding Absolute Value
Recall that in its basic form [latex]\displaystyle{f}\left({x}\right)={|x|}[/latex], the absolute value function, is one of our toolkit functions. The absolute value function is commonly thought of as providing the distance the number is from zero on a number line. Algebraically, for whatever the input value is, the output is the value without regard to sign.A General Note: Absolute Value Function
The absolute value function can be defined as a piecewise function$latex f(x) = \begin{array}{l} x ,\ x \geq 0 \\ -x , x < 0\\ \end{array} $
Example: Determine a Number within a Prescribed Distance
Describe all values [latex]x[/latex] within or including a distance of 4 from the number 5.Answer:
 We want the distance between [latex]x[/latex] and 5 to be less than or equal to 4. We can draw a number line to represent the condition to be satisfied.
The distance from [latex]x[/latex] to 5 can be represented using the absolute value as [latex]|x - 5|[/latex]. We want the values of [latex]x[/latex] that satisfy the condition [latex]|x - 5|\le 4[/latex].
We want the distance between [latex]x[/latex] and 5 to be less than or equal to 4. We can draw a number line to represent the condition to be satisfied.
The distance from [latex]x[/latex] to 5 can be represented using the absolute value as [latex]|x - 5|[/latex]. We want the values of [latex]x[/latex] that satisfy the condition [latex]|x - 5|\le 4[/latex].
Analysis of the Solution
Note that [latex-display]\displaystyle{-4}\le{x - 5}[/latex-display] [latex-display]\displaystyle{1}\le{x}[/latex-display] And: [latex-display]\displaystyle{x-5}\le{4}[/latex-display] [latex-display]\displaystyle{x}\le{9}[/latex-display] So [latex]|x - 5|\le 4[/latex] is equivalent to [latex]1\le x\le 9[/latex]. However, mathematicians generally prefer absolute value notation.Try It
Describe all values [latex]x[/latex] within a distance of 3 from the number 2.Answer: [latex-display]|x - 2|\le 3[/latex-display]
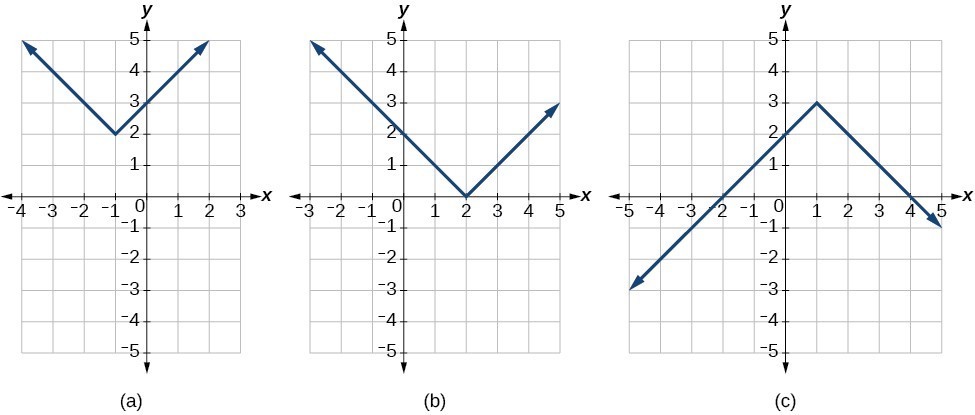 (a) The absolute value function does not intersect the horizontal axis. (b) The absolute value function intersects the horizontal axis at one point. (c) The absolute value function intersects the horizontal axis at two points.
(a) The absolute value function does not intersect the horizontal axis. (b) The absolute value function intersects the horizontal axis at one point. (c) The absolute value function intersects the horizontal axis at two points.Find the Intercepts of an Absolute Value Function
Knowing how to solve problems involving absolute value functions is useful. For example, we may need to identify numbers or points on a line that are at a specified distance from a given reference point.How To: Given the formula for an absolute value function, find the horizontal intercepts of its graph.
- Isolate the absolute value term.
- Use [latex]|A|=B[/latex] to write [latex]A=B[/latex] or [latex]\mathrm{-A}=B[/latex], assuming [latex]B>0[/latex].
- Solve for [latex]x[/latex].
Example: Finding the Zeros of an Absolute Value Function
For the function [latex]f\left(x\right)=|4x+1|-7[/latex] , find the values of [latex]x[/latex] such that [latex]\text{ }f\left(x\right)=0[/latex] .Answer:
[latex]\begin{array}{l}0=|4x+1|-7\hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \text{Substitute 0 for }f\left(x\right).\hfill \\ 7=|4x+1|\hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \text{Isolate the absolute value on one side of the equation}.\hfill \\ \hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \hfill \\ \hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \hfill \\ \hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \hfill \\ 7=4x+1\hfill & \text{or}\hfill & \hfill & \hfill & \hfill & -7=4x+1\hfill & \text{Break into two separate equations and solve}.\hfill \\ 6=4x\hfill & \hfill & \hfill & \hfill & \hfill & -8=4x\hfill & \hfill \\ \hfill & \hfill & \hfill & \hfill & \hfill & \hfill & \hfill \\ x=\frac{6}{4}=1.5\hfill & \hfill & \hfill & \hfill & \hfill & \text{ }x=\frac{-8}{4}=-2\hfill & \hfill \end{array}[/latex]
 The function outputs 0 when [latex]x=1.5[/latex] or [latex]x=-2[/latex].
The function outputs 0 when [latex]x=1.5[/latex] or [latex]x=-2[/latex].
Try It
For the function [latex]f\left(x\right)=|2x - 1|-3[/latex], find the values of [latex]x[/latex] such that [latex]f\left(x\right)=0[/latex].Answer: [latex]x=-1[/latex] or [latex]x=2[/latex]
Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- Precalculus. Provided by: OpenStax Authored by: Jay Abramson, et al.. Located at: https://openstax.org/books/precalculus/pages/1-introduction-to-functions. License: CC BY: Attribution. License terms: Download For Free at : http://cnx.org/contents/[email protected]..
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
- Question ID 469. Authored by: WebWork-Rochester, mb Lippman,David. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 60791. Authored by: Day, Alyson. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 40657. Authored by: Jenck,Michael. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
