We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > College Algebra

Horizontal and Vertical Translations of Exponential Functions

Learning Objectives

  • Graph exponential functions shifted horizontally or vertically and write the associated equation
Transformations of exponential graphs behave similarly to those of other functions. Just as with other parent functions, we can apply the four types of transformations—shifts, reflections, stretches, and compressions—to the parent function [latex]f\left(x\right)={b}^{x}[/latex] without loss of shape. For instance, just as the quadratic function maintains its parabolic shape when shifted, reflected, stretched, or compressed, the exponential function also maintains its general shape regardless of the transformations applied.

Graphing a Vertical Shift

The first transformation occurs when we add a constant d to the parent function [latex]f\left(x\right)={b}^{x}[/latex], giving us a vertical shift d units in the same direction as the sign. For example, if we begin by graphing a parent function, [latex]f\left(x\right)={2}^{x}[/latex], we can then graph two vertical shifts alongside it, using [latex]d=3[/latex]: the upward shift, [latex]g\left(x\right)={2}^{x}+3[/latex] and the downward shift, [latex]h\left(x\right)={2}^{x}-3[/latex]. Both vertical shifts are shown in the figure below.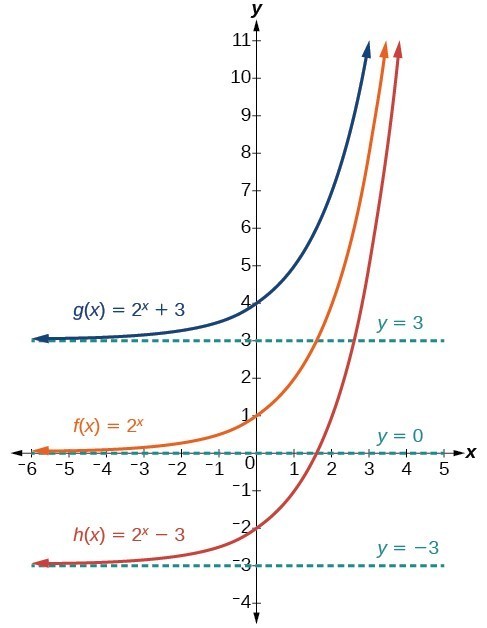 Graph of three functions, g(x) = 2^x+3 in blue with an asymptote at y=3, f(x) = 2^x in orange with an asymptote at y=0, and h(x)=2^x-3 with an asymptote at y=-3. Note that each functions’ transformations are described in the text. Observe the results of shifting [latex]f\left(x\right)={2}^{x}[/latex] vertically:
  • The domain, [latex]\left(-\infty ,\infty \right)[/latex] remains unchanged.
  • When the function is shifted up 3 units to [latex]g\left(x\right)={2}^{x}+3[/latex]:
    • The y-intercept shifts up 3 units to [latex]\left(0,4\right)[/latex].
    • The asymptote shifts up 3 units to [latex]y=3[/latex].
    • The range becomes [latex]\left(3,\infty \right)[/latex].
  • When the function is shifted down 3 units to [latex]h\left(x\right)={2}^{x}-3[/latex]:
    • The y-intercept shifts down 3 units to [latex]\left(0,-2\right)[/latex].
    • The asymptote also shifts down 3 units to [latex]y=-3[/latex].
    • The range becomes [latex]\left(-3,\infty \right)[/latex].

Try it

  1. Use the slider in the graph below to create a graph of [latex]f(x) = 2^x[/latex] that has been shifted 4 units up. Add a line that represents the horizontal asymptote for this function. What is the equation for this function? What is the new y-intercept? What is it's domain and range?
  2. Now create a graph of the function [latex]f(x) = 2^x[/latex] that has been shifted down 2 units. Add a line that represents the horizontal asymptote.What is the equation for this function? What is the new y-intercept? What is it's domain and range?
https://www.desmos.com/calculator/5mrjqegkxk

Answer:

  1. Equation: [latex]f(x) = 2^x+4[/latex], Horizontal Asymptote: [latex]y = 4[/latex], y-intercept: [latex](0,5)[/latex]Domain: [latex](-\infty,\infty)[/latex], Range: [latex](4,\infty)[/latex]
  2. Equation: [latex]f(x) = 2^x-2[/latex], Horizontal Asymptote: [latex]y = -2[/latex], y-intercept: [latex](0,-1)[/latex]Domain: [latex](-\infty,\infty)[/latex], Range: [latex](-2,\infty)[/latex]

 

Graphing a Horizontal Shift

The next transformation occurs when we add a constant c to the input of the parent function [latex]f\left(x\right)={b}^{x}[/latex], giving us a horizontal shift c units in the opposite direction of the sign. For example, if we begin by graphing the parent function [latex]f\left(x\right)={2}^{x}[/latex], we can then graph two horizontal shifts alongside it, using [latex]c=3[/latex]: the shift left, [latex]g\left(x\right)={2}^{x+3}[/latex], and the shift right, [latex]h\left(x\right)={2}^{x - 3}[/latex]. Both horizontal shifts are shown in the figure below.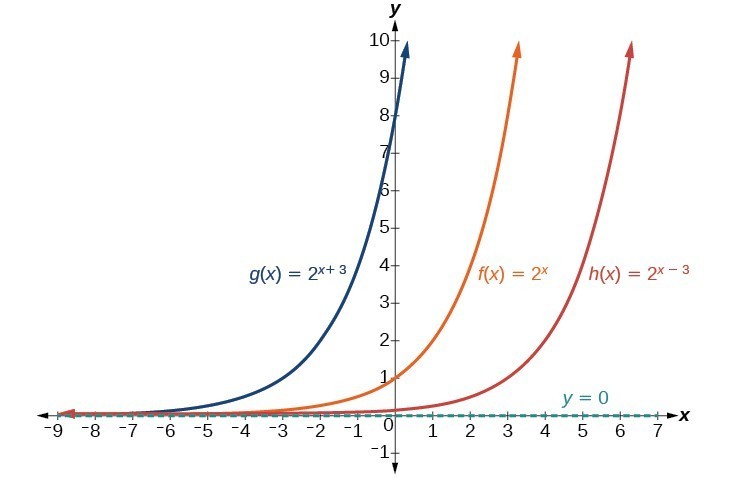 Graph of three functions, g(x) = 2^(x+3) in blue, f(x) = 2^x in orange, and h(x)=2^(x-3). Each functions’ asymptotes are at y=0Note that each functions’ transformations are described in the text. Observe the results of shifting [latex]f\left(x\right)={2}^{x}[/latex] horizontally:
  • The domain, [latex]\left(-\infty ,\infty \right)[/latex], remains unchanged.
  • The asymptote, [latex]y=0[/latex], remains unchanged.
  • The y-intercept shifts such that:
    • When the function is shifted left 3 units to [latex]g\left(x\right)={2}^{x+3}[/latex], the y-intercept becomes [latex]\left(0,8\right)[/latex]. This is because [latex]{2}^{x+3}=\left(8\right){2}^{x}[/latex], so the initial value of the function is 8.
    • When the function is shifted right 3 units to [latex]h\left(x\right)={2}^{x - 3}[/latex], the y-intercept becomes [latex]\left(0,\frac{1}{8}\right)[/latex]. Again, see that [latex]{2}^{x - 3}=\left(\frac{1}{8}\right){2}^{x}[/latex], so the initial value of the function is [latex]\frac{1}{8}[/latex].

try it

  1. Use the slider in the graph below to create a graph of [latex]f(x) = 2^x[/latex] that has been shifted 4 units to the right. What is the equation for this function? What is the new y-intercept? What are it's domain and range?
  2. Use the slider in the graph below to create a graph of [latex]f(x) = 2^x[/latex] that has been shifted 3 units to the left. What is the equation for this function? What is the new y-intercept? What are it's domain and range?
https://www.desmos.com/calculator/rpv1kea0pz

Answer:

  1. Equation: [latex]f(x) = 2^{x-4}[/latex], y-intercept: [latex](0,\frac{1}{32})[/latex]Domain: [latex](-\infty,\infty)[/latex], Range: [latex](0,\infty)[/latex]
  2. Equation: [latex]f(x) = 2^{x+3}[/latex], y-intercept: [latex](0,8)[/latex]Domain: [latex](-\infty,\infty)[/latex], Range: [latex](0,\infty)[/latex]

 

A General Note: Shifts of the Parent Function [latex]f\left(x\right)={b}^{x}[/latex]

For any constants c and d, the function [latex]f\left(x\right)={b}^{x+c}+d[/latex] shifts the parent function [latex]f\left(x\right)={b}^{x}[/latex]
  • vertically d units, in the same direction of the sign of d.
  • horizontally c units, in the opposite direction of the sign of c.
  • The y-intercept becomes [latex]\left(0,{b}^{c}+d\right)[/latex].
  • The horizontal asymptote becomes y = d.
  • The range becomes [latex]\left(d,\infty \right)[/latex].
  • The domain, [latex]\left(-\infty ,\infty \right)[/latex], remains unchanged.

How To: Given an exponential function with the form [latex]f\left(x\right)={b}^{x+c}+d[/latex], graph the translation.

  1. Draw the horizontal asymptote y = d.
  2. Identify the shift as [latex]\left(-c,d\right)[/latex]. Shift the graph of [latex]f\left(x\right)={b}^{x}[/latex] left c units if c is positive, and right [latex]c[/latex] units if c is negative.
  3. Shift the graph of [latex]f\left(x\right)={b}^{x}[/latex] up d units if d is positive, and down d units if d is negative.
  4. State the domain, [latex]\left(-\infty ,\infty \right)[/latex], the range, [latex]\left(d,\infty \right)[/latex], and the horizontal asymptote [latex]y=d[/latex].

Example: Graphing a Shift of an Exponential Function

Graph [latex]f\left(x\right)={2}^{x+1}-3[/latex]. State the domain, range, and asymptote.

Answer: We have an exponential equation of the form [latex]f\left(x\right)={b}^{x+c}+d[/latex], with [latex]b=2[/latex], [latex]c=1[/latex], and [latex]d=-3[/latex]. Draw the horizontal asymptote [latex]y=d[/latex], so draw [latex]y=-3[/latex]. Identify the shift as [latex]\left(-c,d\right)[/latex], so the shift is [latex]\left(-1,-3\right)[/latex].

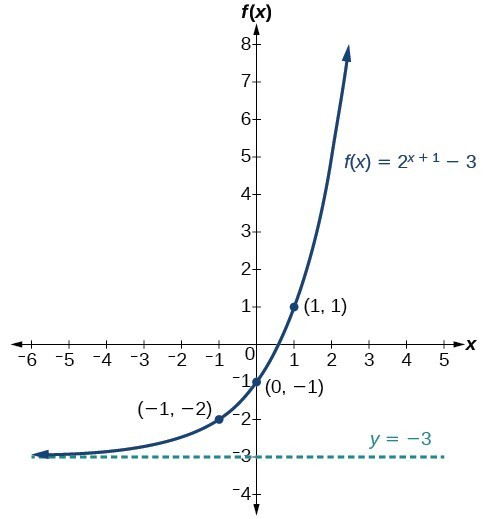Graph of the function, f(x) = 2^(x+1)-3, with an asymptote at y=-3. Labeled points in the graph are (-1, -2), (0, -1), and (1, 1). The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(-3,\infty \right)[/latex]; the horizontal asymptote is [latex]y=-3[/latex].
Shift the graph of [latex]f\left(x\right)={b}^{x}[/latex] left 1 units and down 3 units. The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(-3,\infty \right)[/latex]; the horizontal asymptote is [latex]y=-3[/latex].

Try It

Use the sliders in the graph below to create a graph of the function [latex]f\left(x\right)={2}^{x - 1}+3[/latex]. State domain, range, and asymptote. https://www.desmos.com/calculator/e5l4eca3ob

Answer: The domain is [latex]\left(-\infty ,\infty \right)[/latex]; the range is [latex]\left(3,\infty \right)[/latex]; the horizontal asymptote is y = 3.

In the following video, we show more examples of the difference between horizontal and vertical translations of exponential functions and the resultant graphs and equations. https://youtu.be/phYxEeJ7ZW4

Use a Graph to Approximate a Solution to an Exponential Equation

Graphing can help you confirm or find the solution to an exponential equation. An exponential equation is different from a function because a function is a large collection of points made of inputs and corresponding outputs, whereas equations that you have seen typically have one, two, or no solutions.  For example, [latex]f(x)=2^{x}[/latex] is a function and is comprised of many points [latex](x,f(x))[/latex], and [latex]4=2^{x}[/latex] can be solved to find the specific value for x that makes it a true statement. The graph below shows the intersection of the line [latex]f(x)=4[/latex], and [latex]f(x)=2^{x}[/latex], you can see they cross at [latex]y=4[/latex]. https://www.desmos.com/calculator/lhmpdkbjt0   In the next example, you can try this for yourself.

Example : Approximating the Solution of an Exponential Equation

Use Desmos to solve [latex]42=1.2{\left(5\right)}^{x}+2.8[/latex] graphically.

Answer: First, graph the function [latex]f(x)=1.2{\left(5\right)}^{x}+2.8[/latex] , then add another function [latex]f(x) = 42[/latex]. Desmos automatically calculates points of interest  including intersections.  Essentially, you are looking for the intersection of two functions. Click on the point of intersection, and you will see the the x and y values for the point. Your graph will look like this: https://www.desmos.com/calculator/ozaejvejqn

Try It

Solve [latex]4=7.85{\left(1.15\right)}^{x}-2.27[/latex] graphically. Round to the nearest thousandth.

Answer: [latex]x\approx -1.608[/latex]

 

Licenses & Attributions

CC licensed content, Original

  • Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
  • Horizontal and Vertical Translations of Exponential Functions Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/5mrjqegkxk. License: Public Domain: No Known Copyright.
  • Horizontal and Vertical Translations of Exponential Functions 2 Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/rpv1kea0pz. License: Public Domain: No Known Copyright.
  • Horizontal and Vertical Translations of Exponential Functions 3 Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/e5l4eca3ob. License: Public Domain: No Known Copyright.
  • Solve Exponential Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/lhmpdkbjt0. License: Public Domain: No Known Copyright.
  • Horizontal and Vertical Translations of Exponential Functions 4 Interactive. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/ozaejvejqn. License: Public Domain: No Known Copyright.

CC licensed content, Shared previously

  • College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://openstax.org/books/college-algebra/pages/1-introduction-to-prerequisites. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/[email protected].
  • Question ID 63064. Authored by: Brin,Leon. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
  • Ex: Match the Graphs of Translated Exponential Function to Equations. Authored by: James Sousa (Mathispower4u.com). License: CC BY: Attribution.