Intercepts of Quadratic Functions
Learning Objectives
- Find the y-intercept of a quadratic function
- Find the real-number x-intercepts, or roots of a quadratic function using factoring and the quadratic formula
 Number of x-intercepts of a parabola
Number of x-intercepts of a parabolaHow To: Given a quadratic function , find the y- and x-intercepts.
- Evaluate to find the y-intercept.
- Solve the quadratic equation to find the x-intercepts.
Example: Finding the y- and x-Intercepts of a Parabola
Find the y- and x-intercepts of the quadratic .Answer: We find the y-intercept by evaluating .
So the y-intercept is at . For the x-intercepts, or roots, we find all solutions of .
In this case, the quadratic can be factored easily, providing the simplest method for solution.
So the roots are at and .
Analysis of the Solution
By graphing the function, we can confirm that the graph crosses the y-axis at . We can also confirm that the graph crosses the x-axis at and .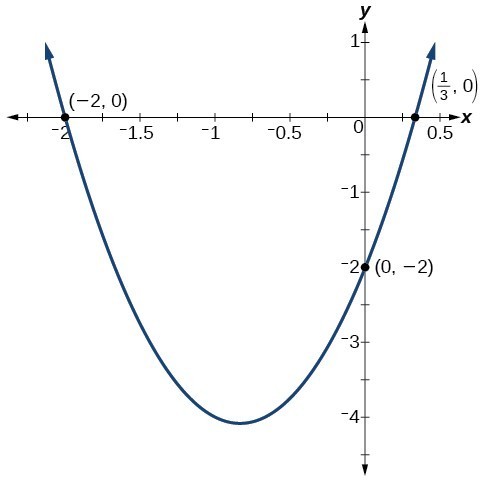
How To: Given a quadratic function, find the x-intercepts by rewriting in standard form.
- Substitute a and b into .
- Substitute x = h into the general form of the quadratic function to find k.
- Rewrite the quadratic in standard form using h and k.
- Solve for when the output of the function will be zero to find the x-intercepts.
Example: Finding the Roots of a Parabola
Find the x-intercepts of the quadratic function .Answer: We begin by solving for when the output will be zero.
Because the quadratic is not easily factorable in this case, we solve for the intercepts by first rewriting the quadratic in standard form.
We know that a = 2. Then we solve for h and k.
So now we can rewrite in standard form.
We can now solve for when the output will be zero.
The graph has x-intercepts at and .
Analysis of the Solution
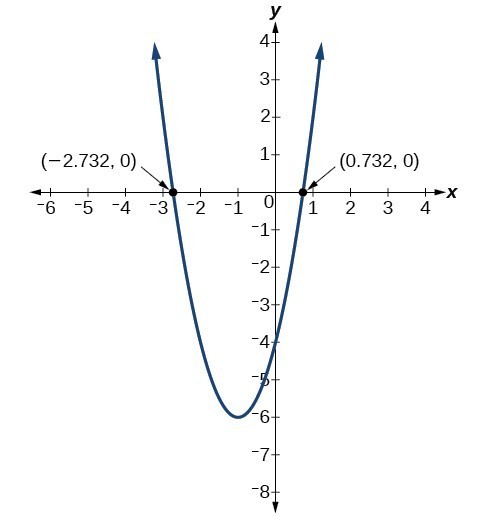 We can check our work by graphing the given function on a graphing utility and observing the roots.
We can check our work by graphing the given function on a graphing utility and observing the roots.
Try It
The function is graphed below. You can use Desmos to find the x-and y-intercepts by clicking on the graph. Four points will appear. List each point, and what kind of point it is, we got you started with the vertex:- Vertex =
Answer: y-intercept at (0, 13)
