Write and Manipulate Inequalities
Learning Objectives
- Use interval notation to express inequalities
- Use properties of inequalities
Indicating the solution to an inequality such as x≥4 can be achieved in several ways.
We can use a number line as shown below. The blue ray begins at x=4 and, as indicated by the arrowhead, continues to infinity, which illustrates that the solution set includes all real numbers greater than or equal to 4.
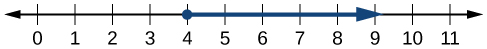 We can use set-builder notation: {x∣x≥4}, which translates to "all real numbers x such that x is greater than or equal to 4." Notice that braces are used to indicate a set.
The third method is interval notation, in which solution sets are indicated with parentheses or brackets. The solutions to x≥4 are represented as [4,∞). This is perhaps the most useful method, as it applies to concepts studied later in this course and to other higher-level math courses.
The main concept to remember is that parentheses represent solutions greater or less than the number, and brackets represent solutions that are greater than or equal to or less than or equal to the number. Use parentheses to represent infinity or negative infinity, since positive and negative infinity are not numbers in the usual sense of the word and, therefore, cannot be "equaled." A few examples of an interval, or a set of numbers in which a solution falls, are [−2,6), or all numbers between −2 and 6, including −2, but not including 6; (−1,0), all real numbers between, but not including −1 and 0; and (−∞,1], all real numbers less than and including 1. The table below outlines the possibilities.
We can use set-builder notation: {x∣x≥4}, which translates to "all real numbers x such that x is greater than or equal to 4." Notice that braces are used to indicate a set.
The third method is interval notation, in which solution sets are indicated with parentheses or brackets. The solutions to x≥4 are represented as [4,∞). This is perhaps the most useful method, as it applies to concepts studied later in this course and to other higher-level math courses.
The main concept to remember is that parentheses represent solutions greater or less than the number, and brackets represent solutions that are greater than or equal to or less than or equal to the number. Use parentheses to represent infinity or negative infinity, since positive and negative infinity are not numbers in the usual sense of the word and, therefore, cannot be "equaled." A few examples of an interval, or a set of numbers in which a solution falls, are [−2,6), or all numbers between −2 and 6, including −2, but not including 6; (−1,0), all real numbers between, but not including −1 and 0; and (−∞,1], all real numbers less than and including 1. The table below outlines the possibilities.
| Set Indicated |
Set-Builder Notation |
Interval Notation |
| All real numbers between a and b, but not including a or b |
{x∣a<x<b} |
(a,b) |
| All real numbers greater than a, but not including a |
{x∣x>a} |
(a,∞) |
| All real numbers less than b, but not including b |
{x∣x<b} |
(−∞,b) |
| All real numbers greater than a, including a |
{x∣x≥a} |
[a,∞) |
| All real numbers less than b, including b |
{x∣x≤b} |
(−∞,b] |
| All real numbers between a and b, including a |
{x∣a≤x<b} |
[a,b) |
| All real numbers between a and b, including b |
{x∣a<x≤b} |
(a,b] |
| All real numbers between a and b, including a and b |
{x∣a≤x≤b} |
[a,b] |
| All real numbers less than a or greater than b |
{x∣x<a and x>b} |
(−∞,a)∪(b,∞) |
| All real numbers |
{x∣x is all real numbers} |
(−∞,∞) |
Example: Using Interval Notation to Express All Real Numbers Greater Than or Equal to a
Use interval notation to indicate all real numbers greater than or equal to
−2.
Answer:
Use a bracket on the left of −2 and parentheses after infinity: [−2,∞). The bracket indicates that −2 is included in the set with all real numbers greater than −2 to infinity.
Try It
Use interval notation to indicate all real numbers between and including
−3 and
5.
Answer:
[−3,5]
Example: Using Interval Notation to Express All Real Numbers Less Than or Equal to a or Greater Than or Equal to b
Write the interval expressing all real numbers less than or equal to
−1 or greater than or equal to
1.
Answer:
We have to write two intervals for this example. The first interval must indicate all real numbers less than or equal to 1. So, this interval begins at −∞ and ends at −1, which is written as (−∞,−1].
The second interval must show all real numbers greater than or equal to 1, which is written as [1,∞). However, we want to combine these two sets. We accomplish this by inserting the union symbol, ∪, between the two intervals.
(−∞,−1]∪[1,∞)
Try It
Express all real numbers less than
−2 or greater than or equal to 3 in interval notation.
Answer:
(−∞,−2)∪[3,∞)
try it now
Use the sliders in the graph below to adjust the length of the line.
- Adjust the left endpoint to (-15,0), and the right endpoint to (5,0)
- Write an inequality that represents the line you created.
[practice-area rows="1"][/practice-area]
3. Now slide the point labeled b over to 15. What do you think happened to the line?
[practice-area rows="1"][/practice-area]
4. Now adjust the end points and make your own inequality.
https://www.desmos.com/calculator/4529rytfef
Answer:
With endpoints (-15,0) and (5,0), the values for x on the line are between -15 and 5, so we can write −15<x<5. We made it a strict inequality because the dots on the endpoints of the lines are open.
The line disappeared because the inequality being represented is of the form b<x<a and when b>a the inequality is no longer valid.
Using the Properties of Inequalities
When we work with inequalities, we can usually treat them similarly to but not exactly as we treat equalities. We can use the addition property and the multiplication property to help us solve them. The one exception is when we multiply or divide by a negative number; doing so reverses the inequality symbol.
A General Note: Properties of Inequalities
Addition PropertyMultiplication PropertyIf a<b, then a+c<b+c.If a<b and c>0, then ac<bc.If a<b and c<0, then ac>bc.
These properties also apply to
a≤b,
a>b, and
a≥b.
Example: Demonstrating the Addition Property
Illustrate the addition property for inequalities by solving each of the following:
- x−15<4
- 6≥x−1
- x+7>9
Answer:
The addition property for inequalities states that if an inequality exists, adding or subtracting the same number on both sides does not change the inequality.
- x−15<4x−15+15<4+15x<19Add 15 to both sides.
- 6≥x−16+1≥x−1+17≥xAdd 1 to both sides.
- x+7>9x+7−7>9−7x>2Subtract 7 from both sides.
Try It
Solve
3x−2<1.
Answer:
x<1
Solving Inequalities in One Variable Algebraically
As the examples have shown, we can perform the same operations on both sides of an inequality, just as we do with equations; we combine like terms and perform operations. To solve, we isolate the variable.
Example: Solving an Inequality Algebraically
Solve the inequality:
13−7x≥10x−4.
Answer:
Solving this inequality is similar to solving an equation up until the last step.
13−7x≥10x−413−17x≥−4−17x≥−17x≤1Move variable terms to one side of the inequality.Isolate the variable term.Dividing both sides by −17 reverses the inequality.
The solution set is given by the interval
(−∞,1], or all real numbers less than and including 1.
Try It
Solve the inequality and write the answer using interval notation:
−x+4<21x+1.
Answer: (2,∞)
Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
- Graphing Inequalities. Authored by: Lumen Learning. Located at: https://www.desmos.com/calculator/4529rytfef. License: CC BY: Attribution.
CC licensed content, Shared previously
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. License: CC BY: Attribution. License terms: Download for free at http://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@5.2.
- Question ID 92604, 92605, 92606, 92607. Authored by: Michael Jenck. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 72891. Authored by: Alyson Day. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
- Question ID 58. Authored by: Lippman, David. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
CC licensed content, Specific attribution
- College Algebra. Provided by: OpenStax Authored by: OpenStax College Algebra. Located at: https://cnx.org/contents/9b08c294-057f-4201-9f48-5d6ad992740d@3.278:1/Preface. License: CC BY: Attribution.
