Early Counting Systems
As we begin our journey through the history of mathematics, one question to be asked is “Where do we start?” Depending on how you view mathematics or numbers, you could choose any of a number of launching points from which to begin. Howard Eves suggests the following list of possibilities.[footnote]Eves, Howard; An Introduction to the History of Mathematics, p. 9.[/footnote] Where to start the study of the history of mathematics…- At the first logical geometric “proofs” traditionally credited to Thales of Miletus (600 BCE).
- With the formulation of methods of measurement made by the Egyptians and Mesopotamians/Babylonians.
- Where prehistoric peoples made efforts to organize the concepts of size, shape, and number.
- In pre-human times in the very simple number sense and pattern recognition that can be displayed by certain animals, birds, etc.
- Even before that in the amazing relationships of numbers and shapes found in plants.
- With the spiral nebulae, the natural course of planets, and other universe phenomena.
Recognition of More vs. Less
The idea of number and the process of counting goes back far beyond history began to be recorded. There is some archeological evidence that suggests that humans were counting as far back as 50,000 years ago.[footnote]Eves, p. 9.[/footnote] However, we do not really know how this process started or developed over time. The best we can do is to make a good guess as to how things progressed. It is probably not hard to believe that even the earliest humans had some sense of more and less. Even some small animals have been shown to have such a sense. For example, one naturalist tells of how he would secretly remove one egg each day from a plover’s nest. The mother was diligent in laying an extra egg every day to make up for the missing egg. Some research has shown that hens can be trained to distinguish between even and odd numbers of pieces of food.[footnote]McLeish, John; The Story of Numbers—How Mathematics Has Shaped Civilization, p. 7.[/footnote] With these sorts of findings in mind, it is not hard to conceive that early humans had (at least) a similar sense of more and less. However, our conjectures about how and when these ideas emerged among humans are simply that; educated guesses based on our own assumptions of what might or could have been.Learning Objectives
In this lesson you will:- Determine the number of objects being represented by pebbles placed on an Inca counting board.
- Determine the number represented by a quipu cord
- Identify uses other than counting for a quipu cord
- Become familiar with the evolution of the counting system we use every day
- Write numbers using Roman Numerals
- Convert between Hindu-Arabic and Roman Numerals
The Evolution of Counting and The Inca Counting System
The Need for Simple Counting
As societies and humankind evolved, simply having a sense of more or less, even or odd, etc., would prove to be insufficient to meet the needs of everyday living. As tribes and groups formed, it became important to be able to know how many members were in the group, and perhaps how many were in the enemy’s camp. Certainly it was important for them to know if the flock of sheep or other possessed animals were increasing or decreasing in size. “Just how many of them do we have, anyway?” is a question that we do not have a hard time imagining them asking themselves (or each other). In order to count items such as animals, it is often conjectured that one of the earliest methods of doing so would be with “tally sticks.” These are objects used to track the numbers of items to be counted. With this method, each “stick” (or pebble, or whatever counting device being used) represents one animal or object. This method uses the idea of one to one correspondence. In a one to one correspondence, items that are being counted are uniquely linked with some counting tool. Figure 1.
Figure 1. Figure 2.
Figure 2.Spoken Words
As methods for counting developed, and as language progressed as well, it is natural to expect that spoken words for numbers would appear. Unfortunately, the developments of these words, especially those corresponding to the numbers from one through ten, are not easy to trace. Past ten, however, we do see some patterns:- Eleven comes from “ein lifon,” meaning “one left over.”
- Twelve comes from “twe lif,” meaning “two left over.”
- Thirteen comes from “Three and ten” as do fourteen through nineteen.
- Twenty appears to come from “twe-tig” which means “two tens.”
- Hundred probably comes from a term meaning “ten times.”
Written Numbers
When we speak of “written” numbers, we have to be careful because this could mean a variety of things. It is important to keep in mind that modern paper is only a little more than 100 years old, so “writing” in times past often took on forms that might look quite unfamiliar to us today. As we saw earlier, some might consider wooden sticks with notches carved in them as writing as these are means of recording information on a medium that can be “read” by others. Of course, the symbols used (simple notches) certainly did not leave a lot of flexibility for communicating a wide variety of ideas or information. Other mediums on which “writing” may have taken place include carvings in stone or clay tablets, rag paper made by hand (twelfth century in Europe, but earlier in China), papyrus (invented by the Egyptians and used up until the Greeks), and parchments from animal skins. And these are just a few of the many possibilities. These are just a few examples of early methods of counting and simple symbols for representing numbers. Extensive books, articles and research have been done on this topic and could provide enough information to fill this entire course if we allowed it to. The range and diversity of creative thought that has been used in the past to describe numbers and to count objects and people is staggering. Unfortunately, we don’t have time to examine them all, but it is fun and interesting to look at one system in more detail to see just how ingenious people have been.The Number and Counting System of the Inca Civilization
Background
There is generally a lack of books and research material concerning the historical foundations of the Americas. Most of the “important” information available concentrates on the eastern hemisphere, with Europe as the central focus. The reasons for this may be twofold: first, it is thought that there was a lack of specialized mathematics in the American regions; second, many of the secrets of ancient mathematics in the Americas have been closely guarded.[footnote]Diana, Lind Mae; The Peruvian Quipu in Mathematics Teacher, Issue 60 (Oct., 1967), p. 623–28.[/footnote] The Peruvian system does not seem to be an exception here. Two researchers, Leland Locke and Erland Nordenskiold, have carried out research that has attempted to discover what mathematical knowledge was known by the Incas and how they used the Peruvian quipu, a counting system using cords and knots, in their mathematics. These researchers have come to certain beliefs about the quipu that we will summarize here.Counting Boards
It should be noted that the Incas did not have a complicated system of computation. Where other peoples in the regions, such as the Mayans, were doing computations related to their rituals and calendars, the Incas seem to have been more concerned with the simpler task of record-keeping. To do this, they used what are called the “quipu” to record quantities of items. (We will describe them in more detail in a moment.) However, they first often needed to do computations whose results would be recorded on quipu. To do these computations, they would sometimes use a counting board constructed with a slab of stone. In the slab were cut rectangular and square compartments so that an octagonal (eight-sided) region was left in the middle. Two opposite corner rectangles were raised. Another two sections were mounted on the original surface of the slab so that there were actually three levels available. In the figure shown, the darkest shaded corner regions represent the highest, third level. The lighter shaded regions surrounding the corners are the second highest levels, while the clear white rectangles are the compartments cut into the stone slab.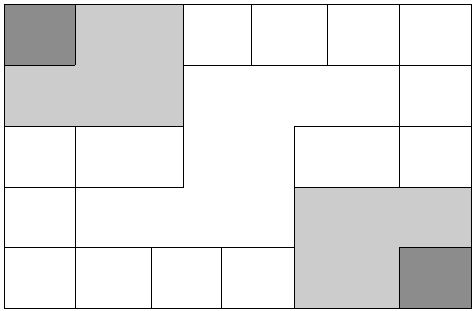 Figure 3.
Figure 3.Example
Suppose you have the following counting board with two different kind of pebbles places as illustrated. Let the solid black pebble represent a dog and the striped pebble represent a cat. How many dogs are being represented?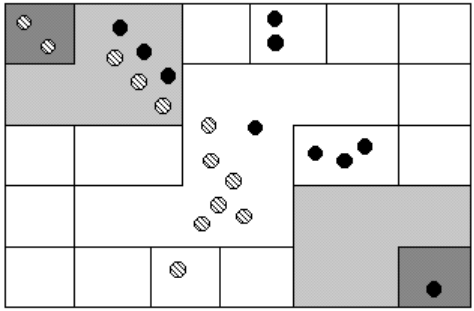
Answer: There are two black pebbles in the outer square regions…these represent 2 dogs. There are three black pebbles in the larger (white) rectangular compartments. These represent 6 dogs. There is one black pebble in the middle region…this represents 3 dogs. There are three black pebbles on the second level…these represent 18 dogs. Finally, there is one black pebble on the highest corner level…this represents 12 dogs. We then have a total of 2+6+3+18+12 = 41 dogs.
Try it now
How many cats are represented on this board?
Answer: 1+6´3+3´6+2´12 = 61 cats
The Quipu
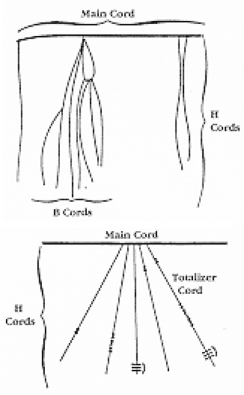 Figure 5.
Figure 5.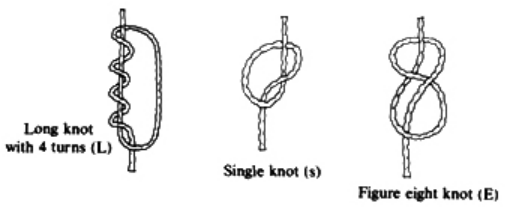 Figure 6
Figure 6Example
What number is represented on the cord shown in figure 7?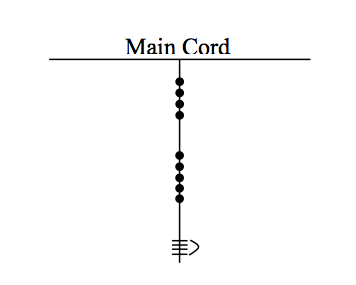
Answer: On the cord, we see a long knot with four turns in it…this represents four in the ones place. Then 5 single knots appear in the tens position immediately above that, which represents 5 tens, or 50. Finally, 4 single knots are tied in the hundreds, representing four 4 hundreds, or 400. Thus, the total shown on this cord is 454.
try it now
What numbers are represented on each of the four cords hanging from the main cord?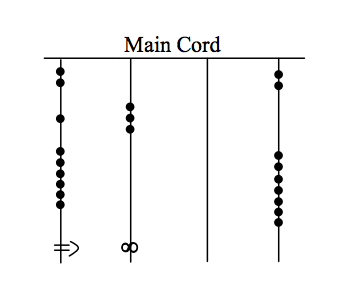
Answer:
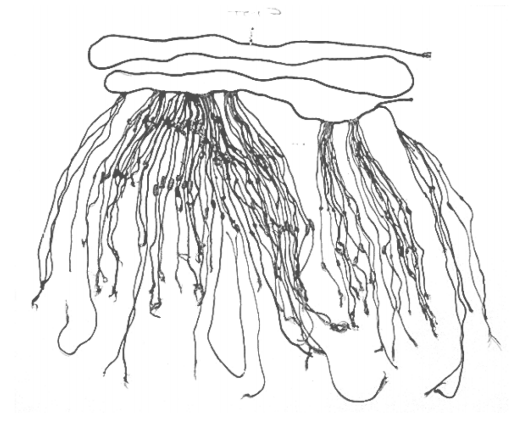 Figure 9.
Figure 9.The Hindu—Arabic Number System and Roman Numerals
The Evolution of a System
Our own number system, composed of the ten symbols {0,1,2,3,4,5,6,7,8,9} is called the Hindu-Arabic system. This is a base-ten (decimal) system since place values increase by powers of ten. Furthermore, this system is positional, which means that the position of a symbol has bearing on the value of that symbol within the number. For example, the position of the symbol 3 in the number 435,681 gives it a value much greater than the value of the symbol 8 in that same number. We’ll explore base systems more thoroughly later. The development of these ten symbols and their use in a positional system comes to us primarily from India.[footnote]http://www-groups.dcs.st-and.ac.uk/~history/HistTopics/Indian_numerals.html[/footnote] Figure 10. Al-Biruni
Figure 10. Al-Biruni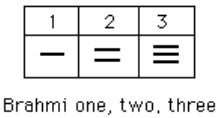 These numerals were used all the way up to the fourth century CE, with variations through time and geographic location. For example, in the first century CE, one particular set of Brahmi numerals took on the following form:[footnote]http://www-groups.dcs.st-and.ac.uk/~history/HistTopics/Indian_numerals.html[/footnote]
These numerals were used all the way up to the fourth century CE, with variations through time and geographic location. For example, in the first century CE, one particular set of Brahmi numerals took on the following form:[footnote]http://www-groups.dcs.st-and.ac.uk/~history/HistTopics/Indian_numerals.html[/footnote]
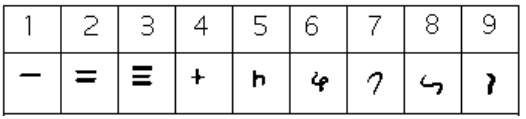 From the fourth century on, you can actually trace several different paths that the Brahmi numerals took to get to different points and incarnations. One of those paths led to our current numeral system, and went through what are called the Gupta numerals. The Gupta numerals were prominent during a time ruled by the Gupta dynasty and were spread throughout that empire as they conquered lands during the fourth through sixth centuries. They have the following form:[footnote]Ibid.[/footnote]
From the fourth century on, you can actually trace several different paths that the Brahmi numerals took to get to different points and incarnations. One of those paths led to our current numeral system, and went through what are called the Gupta numerals. The Gupta numerals were prominent during a time ruled by the Gupta dynasty and were spread throughout that empire as they conquered lands during the fourth through sixth centuries. They have the following form:[footnote]Ibid.[/footnote]
 How the numbers got to their Gupta form is open to considerable debate. Many possible hypotheses have been offered, most of which boil down to two basic types.[footnote]Ibid.[/footnote] The first type of hypothesis states that the numerals came from the initial letters of the names of the numbers. This is not uncommon . . . the Greek numerals developed in this manner. The second type of hypothesis states that they were derived from some earlier number system. However, there are other hypotheses that are offered, one of which is by the researcher Ifrah. His theory is that there were originally nine numerals, each represented by a corresponding number of vertical lines. One possibility is this:[footnote]Ibid.[/footnote]
How the numbers got to their Gupta form is open to considerable debate. Many possible hypotheses have been offered, most of which boil down to two basic types.[footnote]Ibid.[/footnote] The first type of hypothesis states that the numerals came from the initial letters of the names of the numbers. This is not uncommon . . . the Greek numerals developed in this manner. The second type of hypothesis states that they were derived from some earlier number system. However, there are other hypotheses that are offered, one of which is by the researcher Ifrah. His theory is that there were originally nine numerals, each represented by a corresponding number of vertical lines. One possibility is this:[footnote]Ibid.[/footnote]
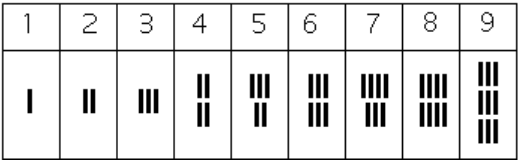 Because these symbols would have taken a lot of time to write, they eventually evolved into cursive symbols that could be written more quickly. If we compare these to the Gupta numerals above, we can try to see how that evolutionary process might have taken place, but our imagination would be just about all we would have to depend upon since we do not know exactly how the process unfolded.
The Gupta numerals eventually evolved into another form of numerals called the Nagari numerals, and these continued to evolve until the eleventh century, at which time they looked like this:[footnote]Ibid.[/footnote]
Because these symbols would have taken a lot of time to write, they eventually evolved into cursive symbols that could be written more quickly. If we compare these to the Gupta numerals above, we can try to see how that evolutionary process might have taken place, but our imagination would be just about all we would have to depend upon since we do not know exactly how the process unfolded.
The Gupta numerals eventually evolved into another form of numerals called the Nagari numerals, and these continued to evolve until the eleventh century, at which time they looked like this:[footnote]Ibid.[/footnote]
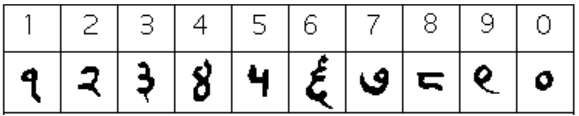 Note that by this time, the symbol for 0 has appeared! The Mayans in the Americas had a symbol for zero long before this, however, as we shall see later in the chapter.
These numerals were adopted by the Arabs, most likely in the eighth century during Islamic incursions into the northern part of India.[footnote]Katz, page 230[/footnote] It is believed that the Arabs were instrumental in spreading them to other parts of the world, including Spain (see below).
Other examples of variations up to the eleventh century include:[footnote]Burton, David M., History of Mathematics, An Introduction, p. 254–255[/footnote]
Note that by this time, the symbol for 0 has appeared! The Mayans in the Americas had a symbol for zero long before this, however, as we shall see later in the chapter.
These numerals were adopted by the Arabs, most likely in the eighth century during Islamic incursions into the northern part of India.[footnote]Katz, page 230[/footnote] It is believed that the Arabs were instrumental in spreading them to other parts of the world, including Spain (see below).
Other examples of variations up to the eleventh century include:[footnote]Burton, David M., History of Mathematics, An Introduction, p. 254–255[/footnote]
 Figure 11. Devangari, eighth century
Figure 11. Devangari, eighth century Figure 12. West Arab Gobar, tenth century
Figure 12. West Arab Gobar, tenth century Figure 13. Spain, 976 BCE
Figure 13. Spain, 976 BCE Figure 14.
Figure 14.Roman Numerals
The numeric system represented by Roman numerals originated in ancient Rome (753 BC–476 AD) and remained the usual way of writing numbers throughout Europe well into the Late Middle Ages (generally comprising the 14th and 15th centuries (c. 1301–1500)). Numbers in this system are represented by combinations of letters from the Latin alphabet. Roman numerals, as used today, are based on seven symbols:| Symbol | I | V | X | L | C | D | M |
| Value | 1 | 5 | 10 | 50 | 100 | 500 | 1,000 |
- I, II, III, IV, V, VI, VII, VIII, IX, X.
| Number | 4 | 9 | 40 | 90 | 400 | 900 |
| Roman Numeral | IV | IX | XL | XC | CD | CM |
- I placed before V or X indicates one less, so four is IV (one less than five) and nine is IX (one less than ten)
- X placed before L or C indicates ten less, so forty is XL (ten less than fifty) and ninety is XC (ten less than a hundred)
- C placed before D or M indicates a hundred less, so four hundred is CD (a hundred less than five hundred) and nine hundred is CM (a hundred less than a thousand)
Example
Write the Hindu-Arabic numeral for MCMIV.Answer: One thousand nine hundred and four, 1904 (M is a thousand, CM is nine hundred and IV is four)
try it now
Modern use
By the 11th century, Hindu–Arabic numerals had been introduced into Europe from al-Andalus, by way of Arab traders and arithmetic treatises. Roman numerals, however, proved very persistent, remaining in common use in the West well into the 14th and 15th centuries, even in accounting and other business records (where the actual calculations would have been made using an abacus). Replacement by their more convenient "Arabic" equivalents was quite gradual, and Roman numerals are still used today in certain contexts. A few examples of their current use are:- Names of monarchs and popes, e.g. Elizabeth II of the United Kingdom, Pope Benedict XVI. These are referred to as regnal numbers; e.g. II is pronounced "the second". This tradition began in Europe sporadically in the Middle Ages, gaining widespread use in England only during the reign of Henry VIII. Previously, the monarch was not known by numeral but by an epithet such as Edward the Confessor. Some monarchs (e.g. Charles IV of Spain and Louis XIV of France) seem to have preferred the use of IIII instead of IV on their coinage (see illustration).
- Generational suffixes, particularly in the US, for people sharing the same name across generations, for example William Howard Taft IV.
- In the French Republican Calendar, initiated during the French Revolution, years were numbered by Roman numerals - from the year I (1792) when this calendar was introduced to the year XIV (1805) when it was abandoned.
- The year of production of films, television shows and other works of art within the work itself. It has been suggested – by BBC News, perhaps facetiously – that this was originally done "in an attempt to disguise the age of films or television programmes."[23] Outside reference to the work will use regular Hindu–Arabic numerals.
- Hour marks on timepieces. In this context, 4 is usually written IIII.
- The year of construction on building faces and cornerstones.
- Page numbering of prefaces and introductions of books, and sometimes of annexes, too.
- Book volume and chapter numbers, as well as the several acts within a play (e.g. Act iii, Scene 2).
- Sequels of some movies, video games, and other works (as in Rocky II).
- Outlines that use numbers to show hierarchical relationships.
- Occurrences of a recurring grand event, for instance:
- The Summer and Winter Olympic Games (e.g. the XXI Olympic Winter Games; the Games of the XXX Olympiad)
- The Super Bowl, the annual championship game of the National Football League (e.g. Super Bowl XXXVII; Super Bowl 50 is a one-time exception[24])
- WrestleMania, the annual professional wrestling event for the WWE (e.g. WrestleMania XXX). This usage has also been inconsistent.

