Solve Systems in Two Variables
Learning Objectives
- Define and classify solutions to systems of linear equations
- Recognize consistent and inconsistent, dependent and independent systems of linear equations
- Determine whether an ordered pair is a solution to a system of linear equations
- Solve a system of linear equations by graphing
- Methods for solving systems
- Use substitution to solve a system algebraically
- Recognize when a system is inconsistent from algebraic results
- Find the break even point for a cost and revenue system
- Write a system of equations based on attendance and cost data
- Solve a system using the elimination method
- Solve a system using the elimination method when multiplication is required
- Recognize when a system is dependent using algebraic results
A system of linear equations consists of two or more linear equations made up of two or more variables such that all equations in the system are considered simultaneously. To find the unique solution to a system of linear equations, we must find a numerical value for each variable in the system that will satisfy all equations in the system at the same time. Some linear systems may not have a solution and others may have an infinite number of solutions. In order for a linear system to have a unique solution, there must be at least as many equations as there are variables. Even so, this does not guarantee a unique solution.
In this section, we will look at systems of linear equations in two variables, which consist of two equations that contain two different variables. For example, consider the following system of linear equations in two variables.
2x+y= 153x−y= 5
The solution to a system of linear equations in two variables is any ordered pair that satisfies each equation independently. In this example, the ordered pair (4, 7) is the solution to the system of linear equations. We can verify the solution by substituting the values into each equation to see if the ordered pair satisfies both equations. Shortly we will investigate methods of finding such a solution if it exists.
2(4)+(7)=15 True3(4)−(7)=5 True
In addition to considering the number of equations and variables, we can categorize systems of linear equations by the number of solutions. A consistent system of equations has at least one solution. A consistent system is considered to be an independent system if it has a single solution, such as the example we just explored. The two lines have different slopes and intersect at one point in the plane. A consistent system is considered to be a dependent system if the equations have the same slope and the same y-intercepts. In other words, the lines coincide so the equations represent the same line. Every point on the line represents a coordinate pair that satisfies the system. Thus, there are an infinite number of solutions.
Another type of system of linear equations is an inconsistent system, which is one in which the equations represent two parallel lines. The lines have the same slope and different y-intercepts. There are no points common to both lines; hence, there is no solution to the system.
A General Note: Types of Linear Systems
There are three types of systems of linear equations in two variables, and three types of solutions.
- An independent system has exactly one solution pair (x,y). The point where the two lines intersect is the only solution.
- An inconsistent system has no solution. Notice that the two lines are parallel and will never intersect.
- A dependent system has infinitely many solutions. The lines are coincident. They are the same line, so every coordinate pair on the line is a solution to both equations.
Below are graphical representations of each type of system.
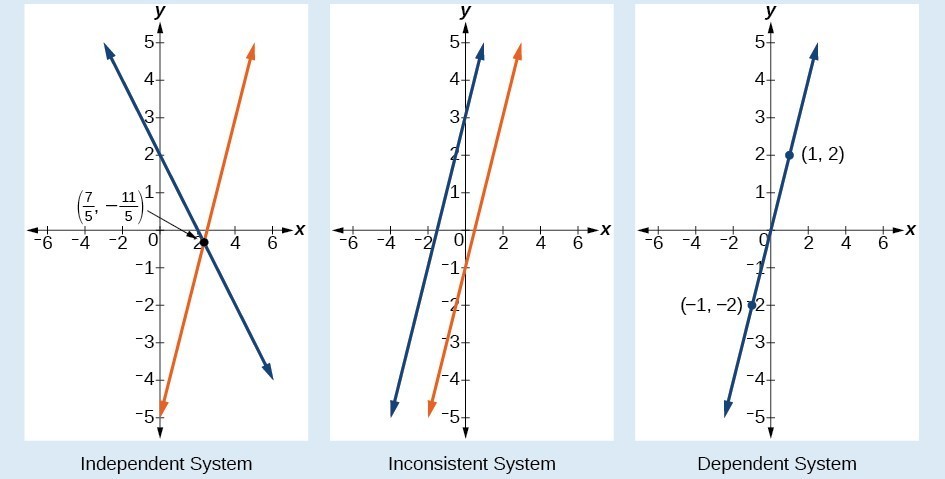
The independent and dependent systems are also consistent because they both have at least one solution.
How To: Given a system of linear equations and an ordered pair, determine whether the ordered pair is a solution.
- Substitute the ordered pair into each equation in the system.
- Determine whether true statements result from the substitution in both equations; if so, the ordered pair is a solution.
Example
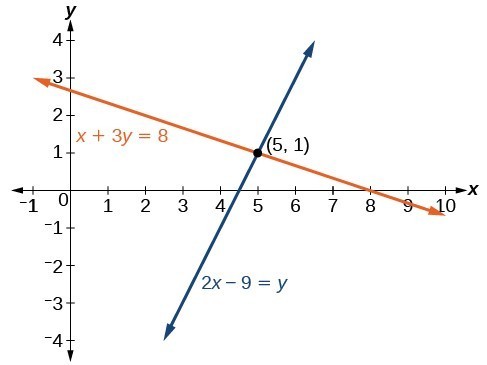
Determine whether the ordered pair
(5,1) is a solution to the given system of equations.
x+3y=82x−9=y
Answer:
Substitute the ordered pair (5,1) into both equations.
(5)+3(1)=8 8=82(5)−9=(1) 1=1TrueTrue
The ordered pair
(5,1) satisfies both equations, so it is the solution to the system.
We can see the solution clearly by plotting the graph of each equation. Since the solution is an ordered pair that satisfies both equations, it is a point on both of the lines and thus the point of intersection of the two lines.
In the following video we will show another example of how to verify whether an ordered pair is a solution to a system of equations.
https://youtu.be/2IxgKgjX00k
Solving Systems of Equations by Graphing
There are multiple methods of solving systems of linear equations. For a system of linear equations in two variables, we can determine both the type of system and the solution by graphing the system of equations on the same set of axes.
Example
Solve the following system of equations by graphing. Identify the type of system.
2x+y=−8x−y=−1
Answer:
Solve the first equation for y.
2x+y=−8y=−2x−8
Solve the second equation for
y.
x−y=−1y=x+1
Graph both equations on the same set of axes as in the figure below.
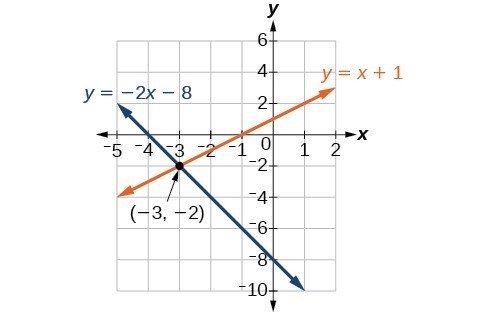
The lines appear to intersect at the point
(−3,−2). We can check to make sure that this is the solution to the system by substituting the ordered pair into both equations
2(−3)+(−2)=−8 −8=−8 (−3)−(−2)=−1 −1=−1TrueTrue
The solution to the system is the ordered pair
(−3,−2), so the system is independent.
Graphing can be used if the system is inconsistent or dependent. In both cases we can still graph the system to determine the type of system and solution. If the two lines are parallel, the system has no solution and is inconsistent. If the two lines are identical, the system has infinite solutions and is a dependent system.
In the following video we show another example of how to identify whether a graphed system has a solution, and identify what type of solution is represented.
https://youtu.be/ZolxtOjcEQY
In our last video we show how to solve a system of equations by first graphing the lines, then identifying the type of solution the system has.
https://youtu.be/Lv832rXAQ5k
Substitution
Solving a linear system in two variables by graphing works well when the solution consists of integer values, but if our solution contains decimals or fractions, it is not the most precise method. We will consider two more methods of solving a system of linear equations that are more precise than graphing. One such method is solving a system of equations by the substitution method, in which we solve one of the equations for one variable and then substitute the result into the second equation to solve for the second variable. Recall that we can solve for only one variable at a time, which is the reason the substitution method is both valuable and practical.
How To: Given a system of two equations in two variables, solve using the substitution method.
- Solve one of the two equations for one of the variables in terms of the other.
- Substitute the expression for this variable into the second equation, then solve for the remaining variable.
- Substitute that solution into either of the original equations to find the value of the first variable. If possible, write the solution as an ordered pair.
- Check the solution in both equations.
Example
Solve the following system of equations by substitution.
−x+y=−5 2x−5y=1
Answer:
First, we will solve the first equation for y.
−x+y=−5 y=x−5
Now we can substitute the expression
x−5 for
y in the second equation.
2x−5y=12x−5(x−5)=1 2x−5x+25=1 −3x=−24 x=8
Now, we substitute
x=8 into the first equation and solve for
y.
−(8)+y=−5 y=3
Our solution is
(8,3).
Check the solution by substituting
(8,3) into both equations.
−x+y=−5−(8)+(3)=−52x−5y=12(8)−5(3)=1TrueTrue
The substitution method can be used to solve any linear system in two variables, but the method works best if one of the equations contains a coefficient of 1 or
–1 so that we do not have to deal with fractions.
In the following video, you will be given an example of solving a systems of two equations using the substitution method.
https://youtu.be/MIXL35YRzRw
If you had chosen the other equation to start with in the previous example, you would still be able to find the same solution. It is really a matter of preference because sometimes solving for a variable will result in having to work with fractions. As you become more experienced with algebra, you will be able to anticipate what choices will lead to more desirable outcomes.
Recall that an inconsistent system consists of parallel lines that have the same slope but different y-intercepts. They will never intersect. When searching for a solution to an inconsistent system, we will come up with a false statement, such as 12=0.
Example
Solve the following system of equations.
x=9−2yx+2y=13
Answer:
We can approach this problem in two ways. Because one equation is already solved for x, the most obvious step is to use substitution.
x+2y=13(9−2y)+2y=139+0y=139=13
Clearly, this statement is a contradiction because
9=13. Therefore, the system has no solution.
The second approach would be to first manipulate the equations so that they are both in slope-intercept form. We manipulate the first equation as follows.
x=9−2y2y=−x+9 y=−21x+29
We then convert the second equation expressed to slope-intercept form.
x+2y=13 2y=−x+13 y=−21x+213
Comparing the equations, we see that they have the same slope but different
y-intercepts. Therefore, the lines are parallel and do not intersect.
y=−21x+29y=−21x+213
Writing the equations in slope-intercept form confirms that the system is inconsistent because all lines will intersect eventually unless they are parallel. Parallel lines will never intersect; thus, the two lines have no points in common. The graphs of the equations in this example are shown below.
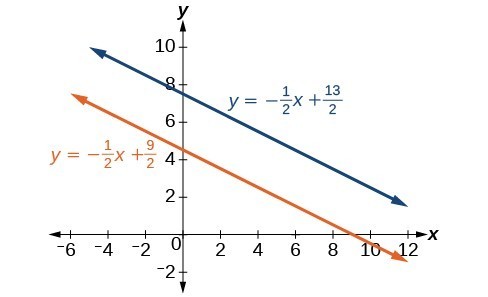
Answer
There is no solution to this system of linear equations.
In the next video we show another example of using substitution to solve a system that has no solution.
https://youtu.be/kTtKfh5gFUc
In our next video we show that a system can have an infinite number of solutions.
https://youtu.be/Pcqb109yK5Q
Consider a skateboard manufacturer’s revenue function, this is the function used to calculate the amount of money that comes into the business. It can be represented by the equation R=xp, where x= quantity and p= price. The revenue function is shown in orange in the graph below.
The cost function is the function used to calculate the costs of doing business. It includes fixed costs, such as rent and salaries, and variable costs, such as utilities. The cost function is shown in blue in the graph below. The x-axis represents the quantity of skateboards produced and sold in hundreds of units. The y-axis represents either cost or revenue in hundreds of dollars.
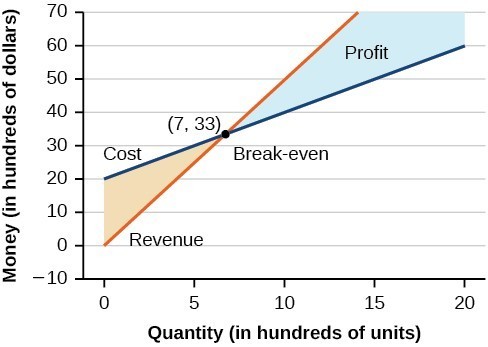 The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
Example
Given the cost function
C(x)=0.85x+35,000 and the revenue function
R(x)=1.55x, find the break-even point.
Answer:
Write the system of equations using y to replace function notation.
y=0.85x+35,000y=1.55x
Substitute the expression
0.85x+35,000 from the first equation into the second equation and solve for
x.
0.85x+35,000=1.55x35,000=0.7x50,000=x
Then, we substitute
x=50,000 into either the cost function or the revenue function.
1.55(50,000)=77,500
The break-even point is
(50,000,77,500).
The cost to produce 50,000 units is $77,500, and the revenue from the sales of 50,000 units is also $77,500. To make a profit, the business must produce and sell more than 50,000 units.
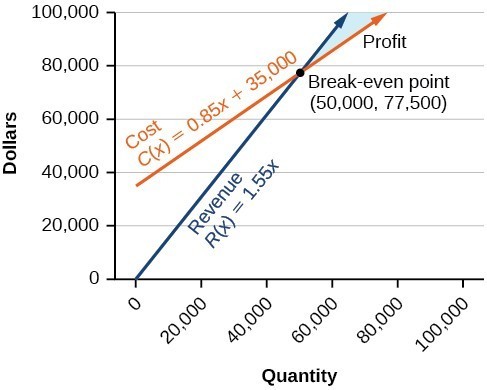
The company will make a profit after 50,000 units are produced.
In the next example, we will show how to write a system of linear equations given attendance and ticket cost data. We will then find the number of tickets purchased based on our system.
Example
The cost of a ticket to the circus is $25.00 for children and $50.00 for adults. On a certain day, attendance at the circus is 2,000 and the total gate revenue is $70,000. How many children and how many adults bought tickets?
Answer:
Let c= the number of children and a= the number of adults in attendance.
The total number of people is 2,000. We can use this to write an equation for the number of people at the circus that day
c+a=2,000
The revenue from all children can be found by multiplying $25.00 by the number of children,
25c. The revenue from all adults can be found by multiplying $50.00 by the number of adults,
50a. The total revenue is $70,000. We can use this to write an equation for the revenue.
25c+50a=70,000
We now have a system of linear equations in two variables.
c+a=2,00025c+50a=70,000
In the first equation, the coefficient of both variables is 1. We can quickly solve the first equation for either
c or
a. We will solve for
a.
c+a=2,000a=2,000−c
Substitute the expression
2,000−c in the second equation for
a and solve for
c.
25c+50(2,000−c)=70,00025c+100,000−50c=70,000 −25c=−30,000 c=1,200
Substitute
c=1,200 into the first equation to solve for
a.
1,200+a=2,000 a=800
We find that
1,200 children and
800 adults bought tickets to the circus that day.
In our last video example we show how to set up a system of linear equations that represents the total cost for admission to a museum.
https://youtu.be/euh9ksWrq0A
Elimination
A third method of solving systems of linear equations is the elimination method. In this method, we add two terms with the same variable, but opposite coefficients, so that the sum is zero. Of course, not all systems are set up with the two terms of one variable having opposite coefficients. Often we must adjust one or both of the equations by multiplication so that one variable will be eliminated by elimination.
Example
Solve the given system of equations by elimination.
x+2y=−1−x+y=3
Answer:
Both equations are already set equal to a constant. Notice that the coefficient of x in the second equation, –1, is the opposite of the coefficient of x in the first equation, 1. We can add the two equations to eliminate x without needing to multiply by a constant.
3y=2x+2y=−1−x+y=3
Now that we have eliminated
x, we can solve the resulting equation for
y.
3y=2 y=32
Then, we substitute this value for
y into one of the original equations and solve for
x
−x+y=3 −x+32=3 −x=3−32 −x=37 x=−37
The solution to this system is
(−37,32).
Check the solution in the first equation.
x+2y=−1 (−37)+2(32)= −37+34= −33= −1=−1True
We gain an important perspective on systems of equations by looking at the graphical representation. In the graph below, you will see that the equations intersect at the solution. We do not need to ask whether there may be a second solution because observing the graph confirms that the system has exactly one solution.

In the following video you will see another example of how to use the method of elimination to solve systems of linear equations.
https://youtu.be/M4IEmwcqR3c
Sometimes we have to do a couple of steps of algebra before we can eliminate a variable from a system solve it. In the next example you will see a technique where we multiply one of the equations in the system by a number that will allow us to eliminate one of the variables.
Example
Solve the given system of equations by the
elimination method.
3x+5y=−11x−2y=11
Answer:
Adding these equations as presented will not eliminate a variable. However, we see that the first equation has 3x in it and the second equation has x. So if we multiply the second equation by −3, the x-terms will add to zero.
x−2y=11−3(x−2y)=−3(11) −3x+6y=−33Multiply both sides by −3.Use the distributive property.
Now, let’s add them.
\begin{array}\ \hfill 3x+5y=−11 \\ \hfill −3x+6y=−33 \\ \text{_____________} \\ \hfill 11y=−44 \\ \hfill y=−4 \end{array}
For the last step, we substitute
y=−4 into one of the original equations and solve for
x.
3x+5y=−113x+5(−4)=−113x−20=−113x=9x=3
Our solution is the ordered pair
(3,−4). Check the solution in the original second equation.
x−2y=11(3)−2(−4)=3+8 =11True
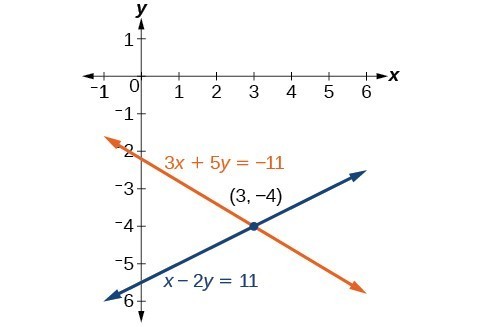
Below is another video example of using the elimination method to solve a system of linear equations.
https://youtu.be/_liDhKops2w
In the next example, we will see that sometimes both equations need to be multiplied by different numbers in order for one variable to be eliminated.
Example
Solve the given system of equations in two variables by elimination.
2x+3y=−165x−10y=30
Answer:
One equation has 2x and the other has 5x. The least common multiple is 10x so we will have to multiply both equations by a constant in order to eliminate one variable. Let’s eliminate x by multiplying the first equation by −5 and the second equation by 2.
−5(2x+3y)=−5(−16) −10x−15y=80 2(5x−10y)=2(30) 10x−20y=60
Then, we add the two equations together.
\begin{array}\ −10x−15y=80 \\ 10x−20y=60 \\ \text{______________} \\ \text{ }−35y=140 \\ y=−4 \end{array}
Substitute
y=−4 into the original first equation.
2x+3(−4)=−162x−12=−162x=−4x=−2
The solution is
(−2,−4). Check it in the other equation.
5x−10y=305(−2)−10(−4)=30 −10+40=30 30=30

Below is a summary of the general steps for using the elimination method to solve a system of equations.
How To: Given a system of equations, solve using the elimination method.
- Write both equations with x- and y-variables on the left side of the equal sign and constants on the right.
- Write one equation above the other, lining up corresponding variables. If one of the variables in the top equation has the opposite coefficient of the same variable in the bottom equation, add the equations together, eliminating one variable. If not, use multiplication by a nonzero number so that one of the variables in the top equation has the opposite coefficient of the same variable in the bottom equation, then add the equations to eliminate the variable.
- Solve the resulting equation for the remaining variable.
- Substitute that value into one of the original equations and solve for the second variable.
- Check the solution by substituting the values into the other equation.
In the next example, we will show how to solve a system with fractions. As with single linear equations, the easiest way to solve is to clear the fractions first with the least common denominator.
Example
Solve the given system of equations in two variables by elimination.
3x+6y=32x−4y= 1
Answer:
First clear each equation of fractions by multiplying both sides of the equation by the least common denominator
6(3x+6y)=6(3) 2x+y=184(2x−4y)=4(1) 2x−y=4
Now multiply the second equation by
−1 so that we can eliminate the
x-variable.
−1(2x−y)=−1(4) −2x+y=−4
Add the two equations to eliminate the
x-variable and solve the resulting equation.
\begin{array}\ \hfill 2x+y=18 \\ \hfill−2x+y=−4 \\ \text{_____________} \\ \hfill 2y=14 \\ \hfill y=7 \end{array}
Substitute
y=7 into the first equation.
2x+(7)=18 2x=11 x=211 =7.5
The solution is
(211,7). Check it in the other equation.
2x−4y=12211−47=1411−47=144=1
In the following video, you will find one more example of using the elimination method to solve a system, this one has coefficients that are fractions.
https://youtu.be/s3S64b1DrtQ
Recall that a dependent system of equations in two variables is a system in which the two equations represent the same line. Dependent systems have an infinite number of solutions because all of the points on one line are also on the other line. After using substitution or elimination, the resulting equation will be an identity, such as 0=0. The last example includes two equations that represent the same line and are therefore dependent.
Example
Find a solution to the system of equations using the
elimination method.
x+3y=23x+9y=6
Answer:
With the elimination method, we want to eliminate one of the variables by adding the equations. In this case, let’s focus on eliminating x. If we multiply both sides of the first equation by −3, then we will be able to eliminate the x -variable.
x+3y=2(−3)(x+3y)=(−3)(2) −3x−9y=−6
Now add the equations.
\begin{array} \hfill−3x−9y=−6 \\ \hfill+3x+9y=6 \\ \hfill \text{_____________} \\ \hfill 0=0 \end{array}
We can see that there will be an infinite number of solutions that satisfy both equations.
If we rewrote both equations in the slope-intercept form, we might know what the solution would look like before adding. Let’s look at what happens when we convert the system to slope-intercept form.
x+3y=2 3y=−x+2 y=−31x+323x+9y=6 9y=−3x+6 y=−93x+96 y=−31x+32
See the graph below. Notice the results are the same. The general solution to the system is
(x,−31x+32).
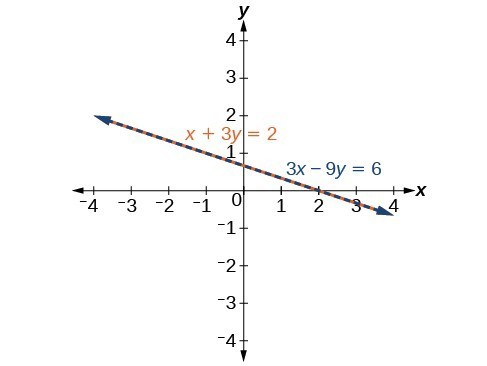
In the following video we show another example of solving a system that is dependent using elimination.
https://youtu.be/NRxh9Q16Ulk
In our last video example we present a system that is inconsistent - it has no solutions which means the lines the equations represent are parallel to each other.
https://youtu.be/z5_ACYtzW98
Licenses & Attributions
CC licensed content, Original
- Determine if an Ordered Pair is a Solution to a System of Linear Equations. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Determine the Number of Solutions to a System of Linear Equations From a Graph. Authored by: James Sousa (Mathispower4u.com) for Lumen Learning. License: CC BY: Attribution.
- Ex 2: Solve a System of Equations Using the Elimination Method. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
CC licensed content, Shared previously
- Ex 2: Solve a System of Equations by Graphing. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex 2: Solve a System of Equations Using Substitution. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Unit 14: Systems of Equations and Inequalities, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology and Education Located at: https://www.nroc.org/. License: CC BY: Attribution.
- Ex: Solve a System of Equations Using Substitution - No Solution. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: Solve a System of Equations Using Substitution - Infinite Solutions. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: Solve an Application Problem Using a System of Linear Equations (09x-43). Authored by: James Sousa (Mathispower4u.com) . License: Public Domain: No Known Copyright.
- Ex 1: Solve a System of Equations Using the Elimination Method. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: Solve a System of Equations Using Eliminations (Fractions). Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: System of Equations Using Elimination (Infinite Solutions). Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- Ex: System of Equations Using Elimination (No Solution). Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
CC licensed content, Specific attribution
- Precalculus. Provided by: OpenStax Authored by: OpenStax College. Located at: https://cnx.org/contents/fd53eae1-fa23-47c7-bb1b-972349835c3c@5.175:1/Preface. License: CC BY: Attribution.
 The independent and dependent systems are also consistent because they both have at least one solution.
The independent and dependent systems are also consistent because they both have at least one solution.

 The lines appear to intersect at the point . We can check to make sure that this is the solution to the system by substituting the ordered pair into both equations
The lines appear to intersect at the point . We can check to make sure that this is the solution to the system by substituting the ordered pair into both equations

 The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
 The company will make a profit after 50,000 units are produced.
The company will make a profit after 50,000 units are produced.




