Divide Polynomials Part I
Learning Outcome
- Multiply and divide polynomials
Divide a Polynomial by a Binomial
Dividing a polynomial by a monomial can be handled by dividing each term in the polynomial separately. This cannot be done when the divisor has more than one term. However, the process of long division can be very helpful with polynomials. Recall how you can use long division to divide two whole numbers, say divided by .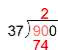 Subtract: is . (If the result is larger than the divisor, , then you need to use a larger number for the quotient.)
Subtract: is . (If the result is larger than the divisor, , then you need to use a larger number for the quotient.)
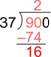 Bring down the next digit and consider how many are in .
Bring down the next digit and consider how many are in .
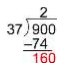 There are four in , so write the next to the two in the quotient. Four is ; write that product below the .
There are four in , so write the next to the two in the quotient. Four is ; write that product below the .
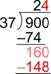 Subtract: is . This is less than so the is correct. Since there are no more digits in the dividend to bring down, you are done.
Subtract: is . This is less than so the is correct. Since there are no more digits in the dividend to bring down, you are done.
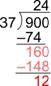 The final answer is R, or . You can check this by multiplying the quotient (without the remainder) by the divisor, and then adding in the remainder. The result should be the dividend:
The final answer is R, or . You can check this by multiplying the quotient (without the remainder) by the divisor, and then adding in the remainder. The result should be the dividend:
To divide polynomials, use the same process. This example shows how to do this when dividing by a binomial.
Example
Divide:Answer:
How many x’s are there in ? That is, what is ?
![]() . Put x in the quotient above the term. These are like terms, which helps to organize the problem.
Write the product of the divisor and the part of the quotient you just found under the dividend. Since , write this underneath, and get ready to subtract.
. Put x in the quotient above the term. These are like terms, which helps to organize the problem.
Write the product of the divisor and the part of the quotient you just found under the dividend. Since , write this underneath, and get ready to subtract.
 Rewrite as its opposite so that you can add the opposite. Adding the opposite is the same as subtracting, and it is easier to do.
Rewrite as its opposite so that you can add the opposite. Adding the opposite is the same as subtracting, and it is easier to do.
![]() Add to and to .
Add to and to .
 Bring down .
Bring down .
 Repeat the process. How many times does x go into ? In other words, what is ?
Repeat the process. How many times does x go into ? In other words, what is ?
 Since , write in the quotient. Multiply and and prepare to subtract the product.
Since , write in the quotient. Multiply and and prepare to subtract the product.
 Rewrite as , so that you can add the opposite.
Rewrite as , so that you can add the opposite.
 Add. In this case, there is no remainder, so you are done.
Add. In this case, there is no remainder, so you are done.
 The answer is .
Check this by multiplying:
The answer is .
Check this by multiplying:
Example
Divide:Answer:
In setting up this problem, notice that there is an term but no term. Add as a “place holder” for this term. Since 0 times anything is 0, you are not changing the value of the dividend.
![]() Focus on the first terms again: how many x’s are there in ? Since , put in the quotient.
Multiply: ; write this underneath the dividend, and prepare to subtract.
Focus on the first terms again: how many x’s are there in ? Since , put in the quotient.
Multiply: ; write this underneath the dividend, and prepare to subtract.
![]() Rewrite the subtraction using the opposite of the expression . Then add.
Rewrite the subtraction using the opposite of the expression . Then add.
 Bring down the rest of the expression in the dividend. It is helpful to bring down all of the remaining terms.
Bring down the rest of the expression in the dividend. It is helpful to bring down all of the remaining terms.
 Now, repeat the process with the remaining expression, , as the dividend.
Now, repeat the process with the remaining expression, , as the dividend.
 Remember to watch the signs!
Remember to watch the signs!
 How many x’s are there in x? Since there are , multiply , write this underneath the dividend, and prepare to subtract.
How many x’s are there in x? Since there are , multiply , write this underneath the dividend, and prepare to subtract.
 Continue until the degree of the remainder is less than the degree of the divisor. In this case the degree of the remainder, , is , which is less than the degree of , which is .
Also notice that you have brought down all the terms in the dividend and that the quotient extends to the right edge of the dividend. These are other ways to check whether you have completed the problem.
Continue until the degree of the remainder is less than the degree of the divisor. In this case the degree of the remainder, , is , which is less than the degree of , which is .
Also notice that you have brought down all the terms in the dividend and that the quotient extends to the right edge of the dividend. These are other ways to check whether you have completed the problem.
 You can write the remainder using the symbol R or as a fraction added to the rest of the quotient with the remainder in the numerator and the divisor in the denominator. In this case, since the remainder is negative, you can also subtract the opposite.
Possible forms of the solution are:
Check the result:
You can write the remainder using the symbol R or as a fraction added to the rest of the quotient with the remainder in the numerator and the divisor in the denominator. In this case, since the remainder is negative, you can also subtract the opposite.
Possible forms of the solution are:
Check the result:
- When subtracting, be sure to subtract the whole expression, not just the first term. This is very easy to forget, so be careful!
- Stop when the degree of the remainder is less than the degree of the dividend or when you have brought down all the terms in the dividend and your quotient extends to the right edge of the dividend.
