Graph Linear Functions Using Slope and y-Intercept
Learning Outcome
- Graph a linear function using the slope and y-intercept
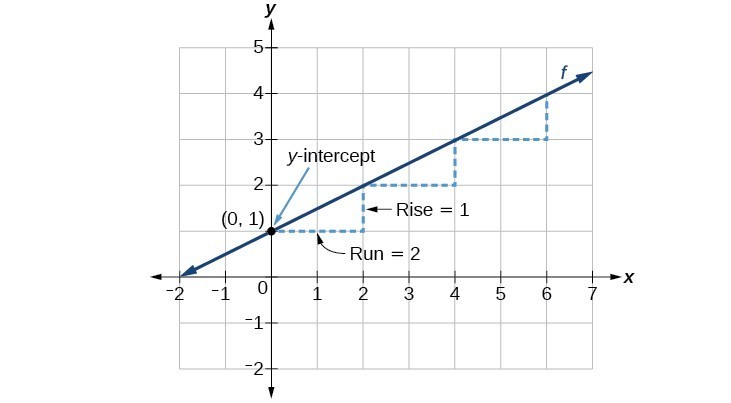
The function is in slope-intercept form, so the slope is . Because the slope is positive, we know the graph will slant upward from left to right. The y-intercept is the point on the graph when . The graph crosses the y-axis at . Now we know the slope and the y-intercept. We can begin graphing by plotting the point . We know that the slope is rise over run, . From our example, we have , which means that the rise is and the run is . So starting from our y-intercept , we can rise and then run , or run and then rise . We repeat until we have a few points and then we draw a line through the points as shown in the graph below.
A General Note: Graphical Interpretation of a Linear Function
In the equation- b is the y-intercept of the graph and indicates the point (0, b) at which the graph crosses the y-axis.
- m is the slope of the line and indicates the vertical displacement (rise) and horizontal displacement (run) between each successive pair of points. Recall the formula for the slope:
How To: Given the equation for a linear function, graph the function using the y-intercept and slope
- Evaluate the function at an input value of zero to find the y-intercept.
- Identify the slope.
- Plot the point represented by the y-intercept.
- Use to determine at least two more points on the line.
- Sketch the line that passes through the points.
Example
Graph using the y-intercept and slope.Answer:
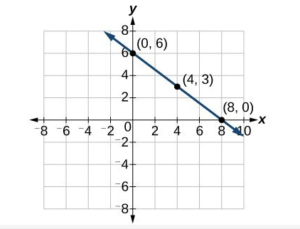
Evaluate the function at to find the y-intercept. The output value when is , so the graph will cross the y-axis at .
According to the equation for the function, the slope of the line is . This tells us that for each vertical decrease in the "rise" of units, the "run" increases by units in the horizontal direction. We can now graph the function by first plotting the y-intercept in the graph below. From the initial value , we move down units and to the right units. We can extend the line to the left and right by using this relationship to plot additional points and then drawing a line through the points.
 The graph slants downward from left to right, which means it has a negative slope as expected.
The graph slants downward from left to right, which means it has a negative slope as expected.
Try It
[ohm_question]79774[/ohm_question]Example
Graph using the slope and y-intercept.Answer:
The slope of this function is and the y-intercept is We can start graphing by plotting the y-intercept and counting down three units and right units. The first stop would be , and the next stop would be .