Radical Expressions and Rational Exponents
Learning Outcomes
- Convert radicals to expressions with rational exponents
- Convert expressions with rational exponents to their radical equivalent
Write an Expression with a Rational Exponent as a Radical
Radicals and fractional exponents are alternate ways of expressing the same thing. In the table below we show equivalent ways to express radicals: with a root, with a rational exponent, and as a principal root.|
Radical Form |
Exponent Form |
Principal Root |
|---|---|---|
|
Radical Form |
Exponent Form |
Principal Root |
|---|---|---|
|
Radical Form |
Exponent Form |
|---|---|
| … | … |
Example
Express in radical form.Answer: Rewrite the expression with the fractional exponent as a radical. The denominator of the fraction determines the root, in this case the cube root.
The parentheses in indicate that the exponent refers to everything within the parentheses.
Example
Express in radical form.Answer: Rewrite the expression with the fractional exponent as a radical. The denominator of the fraction determines the root, in this case the cube root.
The exponent refers only to the part of the expression immediately to the left of the exponent, in this case x, but not the .
Write a Radical Expression as an Expression with a Rational Exponent
 Flexibility
FlexibilityExample
Write as an expression with a rational exponent.Answer: The radical form can be rewritten as the exponent . Remove the radical and place the exponent next to the base.
Example
Express with rational exponents.Answer: Rewrite the radical using a rational exponent. The root determines the fraction. In this case, the index of the radical is , so the rational exponent will be .
Since is outside the radical, it is not included in the grouping symbol and the exponent does not refer to it.
Rational Exponents Whose Numerator is Not Equal to One
All of the numerators for the fractional exponents in the examples above were . You can use fractional exponents that have numerators other than to express roots, as shown below.|
Radical |
Exponent |
|---|---|
| … | … |
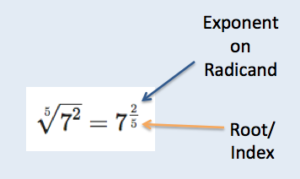 To rewrite a radical using a fractional exponent, the power to which the radicand is raised becomes the numerator and the root/ index becomes the denominator.
To rewrite a radical using a fractional exponent, the power to which the radicand is raised becomes the numerator and the root/ index becomes the denominator.
Writing Rational Exponents
Any radical in the form can be written using a fractional exponent in the form .Example
Rewrite the radicals using a rational exponent, then simplify your result.Answer: 1. can be rewritten as , so in this case , therefore Simplify the exponent. 2. can be rewritten as , so in this case , therefore
Simplify the expression using rules for exponents.
Example
Rewrite the expressions using a radical.Answer:
- , the numerator is and the denominator is , therefore we will have the third root of x squared,
- , the numerator is and the denominator is , so we will have the seventh root of raised to the fourth power.
