The Discriminant
Learning Outcome
- Define the discriminant and use it to classify solutions to quadratic equations
The Discriminant
The quadratic formula not only generates the solutions to a quadratic equation, but also tells us about the nature of the solutions. When we consider the discriminant, or the expression under the radical, [latex]{b}^{2}-4ac[/latex], it tells us whether the solutions are real numbers or complex numbers and how many solutions of each type to expect. Let us explore how the discriminant affects the evaluation of [latex] \sqrt{{{b}^{2}}-4ac}[/latex] in the quadratic formula and how it helps to determine the solution set.- If [latex]b^{2}-4ac>0[/latex], then the number underneath the radical will be a positive value. You can always find the square root of a positive number, so evaluating the quadratic formula will result in two real solutions (one by adding the positive square root and one by subtracting it).
- If [latex]b^{2}-4ac=0[/latex], then you will be taking the square root of [latex]0[/latex], which is [latex]0[/latex]. Since adding and subtracting [latex]0[/latex] both give the same result, the "[latex]\pm[/latex]" portion of the formula does not matter. There will be one real repeated solution.
- If [latex]b^{2}-4ac<0[/latex], then the number underneath the radical will be a negative value. Since you cannot find the square root of a negative number using real numbers, there are no real solutions. However, you can use imaginary numbers. You will then have two complex solutions, one by adding the imaginary square root and one by subtracting it.
| Value of Discriminant | Results |
|---|---|
| [latex]{b}^{2}-4ac=0[/latex] | One repeated rational solution |
| [latex]{b}^{2}-4ac>0[/latex], perfect square | Two rational solutions |
| [latex]{b}^{2}-4ac>0[/latex], not a perfect square | Two irrational solutions |
| [latex]{b}^{2}-4ac<0[/latex] | Two complex solutions |
A General Note: The Discriminant
For [latex]a{x}^{2}+bx+c=0[/latex], where [latex]a[/latex], [latex]b[/latex], and [latex]c[/latex] are real numbers, the discriminant is the expression under the radical in the quadratic formula: [latex]{b}^{2}-4ac[/latex]. It tells us whether the solutions are real numbers or complex numbers and how many solutions of each type to expect.Example
Use the discriminant to find the nature of the solutions to the following quadratic equations:- [latex]{x}^{2}+4x+4=0[/latex]
- [latex]8{x}^{2}+14x+3=0[/latex]
- [latex]3{x}^{2}-5x - 2=0[/latex]
- [latex]3{x}^{2}-10x+15=0[/latex]
Answer: Calculate the discriminant [latex]{b}^{2}-4ac[/latex] for each equation and state the expected type of solutions.
- [latex]{x}^{2}+4x+4=0[/latex] [latex] \\ {b}^{2}-4ac={\left(4\right)}^{2}-4\left(1\right)\left(4\right)=0[/latex] [latex]\text{There will be one repeated rational solution.}[/latex]
- [latex]8{x}^{2}+14x+3=0[/latex][latex] \\ {b}^{2}-4ac={\left(14\right)}^{2}-4\left(8\right)\left(3\right)=100[/latex] [latex]\text{100 is a perfect square, so there will be two rational solutions.}[/latex]
- [latex]3{x}^{2}-5x - 2=0[/latex][latex] \\ {b}^{2}-4ac={\left(-5\right)}^{2}-4\left(3\right)\left(-2\right)=49[/latex] [latex]\text{49 is a perfect square, so there will be two rational solutions.}[/latex]
- [latex]3{x}^{2}-10x+15=0[/latex][latex] \\ {b}^{2}-4ac={\left(-10\right)}^{2}-4\left(3\right)\left(15\right)=-80[/latex] [latex]\text{There will be two complex solutions.}[/latex]
Example
Use the discriminant to determine how many and what kind of solutions the quadratic equation [latex]x^{2}-4x+10=0[/latex] has.Answer: Evaluate [latex]b^{2}-4ac[/latex]. First note that [latex]a=1,b=−4[/latex], and [latex]c=10[/latex]. [latex-display]\begin{array}{l}b^{2}-4ac=\left(-4\right)^{2}-4\left(1\right)\left(10\right)=16-40=-24\end{array}[/latex-display] The result is a negative number. The discriminant is negative, so the quadratic equation has two complex solutions.
Example
Use the following graphs of quadratic functions to determine how many and what type of solutions the corresponding quadratic equation [latex]f(x)=0[/latex] will have. Determine whether the discriminant will be greater than, less than, or equal to zero for each. a. b.
b.
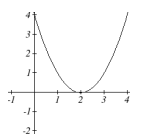 c.
c.
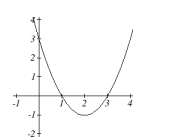
Answer: a. This quadratic function does not touch or cross the x-axis; therefore, the corresponding equation [latex]f(x)=0[/latex] will have complex solutions. This implies that [latex]b^{2}-4ac<0[/latex]. b. This quadratic function touches the x-axis exactly once which implies there is one repeated solution to the equation [latex]f(x)=0[/latex]. We can then say that [latex]b^{2}-4ac=0[/latex]. c. In our final graph, the quadratic function crosses the x-axis twice which tells us that there are two real number solutions to the equation [latex]f(x)=0[/latex], and therefore [latex]b^{2}-4ac>0[/latex].
| Discriminant | Number and Type of Solutions | Graph of Quadratic Function |
| [latex]b^{2}-4ac<0[/latex] | two complex solutions | will not cross the x-axis |
| [latex]b^{2}-4ac=0[/latex] | one real repeated solution | will touch x-axis once |
| [latex]b^{2}-4ac>0[/latex] | two real solutions | will cross x-axis twice |
Summary
The discriminant of the quadratic formula is the quantity under the radical, [latex] {{b}^{2}}-4ac[/latex]. It determines the number and the type of solutions that a quadratic equation has. If the discriminant is positive, there are [latex]2[/latex] real solutions. If it is [latex]0[/latex], there is [latex]1[/latex] real repeated solution. If the discriminant is negative, there are [latex]2[/latex] complex solutions (but no real solutions). The discriminant can also tell us about the behavior of the graph of a quadratic function.Licenses & Attributions
CC licensed content, Original
- Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.
CC licensed content, Shared previously
- Ex: The Discriminant. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.
- College Algebra. Provided by: OpenStax Authored by: Abramson, Jay et al.. Located at: https://cnx.org/contents/[email protected]:1/Preface. License: CC BY: Attribution.
