Finding the Prime Factorization of a Composite Number
Learning Outcomes
- Find the prime factorization of a number using the factor tree method
- Find the prime factorization of a number using the ladder method
Prime Factorization
The prime factorization of a number is the product of prime numbers that equals the number. Doing the Manipulative Mathematics activity "Prime Numbers" will help you develop a better sense of prime numbers. You may want to refer to the following list of prime numbers less than as you work through this section.Prime Factorization Using the Factor Tree Method
One way to find the prime factorization of a number is to make a factor tree. We start by writing the number, and then writing it as the product of two factors. We write the factors below the number and connect them to the number with a small line segment—a "branch" of the factor tree. If a factor is prime, we circle it (like a bud on a tree), and do not factor that "branch" any further. If a factor is not prime, we repeat this process, writing it as the product of two factors and adding new branches to the tree. We continue until all the branches end with a prime. When the factor tree is complete, the circled primes give us the prime factorization. For example, let’s find the prime factorization of . We can start with any factor pair such as and . We write and below with branches connecting them.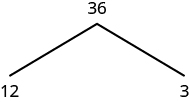 The factor is prime, so we circle it. The factor is composite, so we need to find its factors. Let’s use and . We write these factors on the tree under the .
The factor is prime, so we circle it. The factor is composite, so we need to find its factors. Let’s use and . We write these factors on the tree under the .
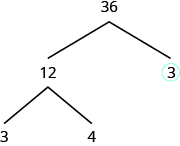 The factor is prime, so we circle it. The factor is composite, and it factors into . We write these factors under the . Since is prime, we circle both .
The factor is prime, so we circle it. The factor is composite, and it factors into . We write these factors under the . Since is prime, we circle both .
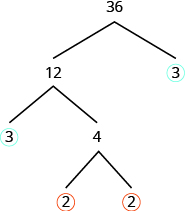 The prime factorization is the product of the circled primes. We generally write the prime factorization in order from least to greatest.
In cases like this, where some of the prime factors are repeated, we can write prime factorization in exponential form.
Note that we could have started our factor tree with any factor pair of . We chose and , but the same result would have been the same if we had started with and and .
The prime factorization is the product of the circled primes. We generally write the prime factorization in order from least to greatest.
In cases like this, where some of the prime factors are repeated, we can write prime factorization in exponential form.
Note that we could have started our factor tree with any factor pair of . We chose and , but the same result would have been the same if we had started with and and .
Find the prime factorization of a composite number using the tree method
- Find any factor pair of the given number, and use these numbers to create two branches.
- If a factor is prime, that branch is complete. Circle the prime.
- If a factor is not prime, write it as the product of a factor pair and continue the process.
- Write the composite number as the product of all the circled primes.
example
Find the prime factorization of using the factor tree method. Solution:| We can start our tree using any factor pair of . Let's use . We circle the because it is prime and so that branch is complete. |  |
| Now we will factor . Let's use . |  |
| Neither factor is prime, so we do not circle either.We factor the , using . We factor . We circle the since they are prime. Now all of the branches end in a prime. |  |
| Write the product of the circled numbers. | |
| Write in exponential form. |
try it
[ohm_question]146554[/ohm_question]example
Find the prime factorization of using the factor tree method.Answer: Solution:
| We start with the factor pair . Neither factor is prime so we factor them further. |  |
| Now the factors are all prime, so we circle them. |  |
| Then we write as the product of all circled primes. |
try it
#145452example
Find the prime factorization of using the ladder method.Answer: Solution:
| Divide the number by the smallest prime, . |  |
| Continue dividing by until it no longer divides evenly. |  |
| The quotient, , is prime, so the ladder is complete. Write the prime factorization of . |
