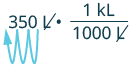example
Nick ran a
10-kilometer race. How many meters did he run?
(credit: William Warby, Flickr)

Solution
We will convert kilometers to meters using the Identity Property of Multiplication and the equivalencies in the reference table from earlier.
|
10 kilometers |
| Multiply the measurement to be converted by 1. |
10km⋅1 |
| Write 1 as a fraction relating kilometers and meters. |
10km⋅1km1000m |
| Simplify. |
1km10km⋅1000m |
| Multiply. |
10,000 m |
|
Nick ran 10,000 meters. |
example
Eleanor’s newborn baby weighed
3200 grams. How many kilograms did the baby weigh?
Answer:
Solution
We will convert grams to kilograms.
|
3200grams |
| Multiply the measurement to be converted by 1. |
3200g⋅1 |
| Write 1 as a fraction relating kilograms and grams. |
3200g⋅1000g1kg |
| Simplify. |
3200g⋅1000g1kg |
| Multiply. |
10003200kilograms |
| Divide. |
3.2 kilograms |
|
The baby weighed 3.2 kilograms. |
example
Convert: 1.
350 liters to kiloliters 2.
4.1 liters to milliliters.
Answer:
Solution
1. We will convert liters to kiloliters. In the reference table, we see that 1 kiloliter=1000 liters.
|
350 L |
| Multiply by 1, writing 1 as a fraction relating liters to kiloliters. |
350L⋅1000L1kl |
| Simplify. |
350L⋅1000L1kl |
| Move the decimal 3 units to the left. |
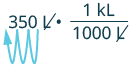 |
|
0.35 kL |
2. We will convert liters to milliliters. In the reference table, we see that
1 liter=1000milliliters.
|
4.1 L |
| Multiply by 1, writing 1 as a fraction relating milliliters to liters. |
4.1L⋅1L1000ml |
| Simplify. |
4.1LL⋅1L1000ml |
| Move the decimal 3 units to the right. |
 |
|
4100 mL |
example
Ryland is
1.6 meters tall. His younger brother is
85 centimeters tall. How much taller is Ryland than his younger brother?
Answer:
Solution
We will subtract the lengths in meters. Convert 85 centimeters to meters by moving the decimal 2 places to the left; 85 cm is the same as 0.85 m.
Now that both measurements are in meters, subtract to find out how much taller Ryland is than his brother.
\begin{array}{}\\ \\ \hfill \text{1.60 m}\\ \hfill \underset{\text{_______}}{\text{-0.85 m}}\\ \hfill \text{0.75 m}\end{array}
Ryland is 0.75 meters taller than his brother.
example
Dena’s recipe for lentil soup calls for
150 milliliters of olive oil. Dena wants to triple the recipe. How many liters of olive oil will she need?
Answer:
Solution
We will find the amount of olive oil in milliliters then convert to liters.
|
Triple 150 mL |
| Translate to algebra. |
3⋅150mL |
| Multiply. |
450mL |
| Convert to liters. |
450mL⋅1mL0.001L |
| Simplify. |
0.45L |
|
Dena needs 0.45 liter of olive oil. |
 Solution
We will convert kilometers to meters using the Identity Property of Multiplication and the equivalencies in the reference table from earlier.
Solution
We will convert kilometers to meters using the Identity Property of Multiplication and the equivalencies in the reference table from earlier.