Notation and Modeling Subtraction of Integers
Learning Outcomes
- Model integer subtraction with color counters
example
Model: . Solution:| Interpret the expression. | means take away . |
| Model the first number. Start with positives. |  |
| Take away the second number. So take away positives. |  |
| Find the counters that are left. |  |
| . The difference between and is . |
try it
Model the expression:Answer:

Answer:

example
Model:Answer: Solution:
| Interpret the expression. | means take away . |
| Model the first number. Start with negatives. |  |
| Take away the second number. So take away negatives. |  |
| Find the number of counters that are left. |  |
| . The difference between and is . |
try it
Model the expression:Answer:

Answer:

- First, we subtracted positives from positives to get positives.
- Then we subtracted negatives from negatives to get negatives.
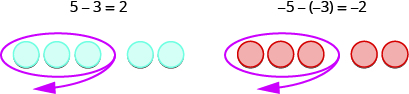 Now let’s see what happens when we subtract one positive and one negative number. We will need to use both positive and negative counters and sometimes some neutral pairs, too. Adding a neutral pair does not change the value.
Now let’s see what happens when we subtract one positive and one negative number. We will need to use both positive and negative counters and sometimes some neutral pairs, too. Adding a neutral pair does not change the value.
example
Model: .Answer: Solution:
| Interpret the expression. | means take away . |
| Model the first number. Start with negatives. |  |
| Take away the second number. So we need to take away positives. | |
| But there are no positives to take away. Add neutral pairs until you have positives. |  |
| Now take away positives. |  |
| Count the number of counters that are left. |  |
| . The difference of and is . |
try it
Model the expression:Answer:

Answer:

example
Model: .Answer: Solution:
| Interpret the expression. | means take away . |
| Model the first number. Start with positives. |  |
| Take away the second number, so take away negatives. | |
| But there are no negatives to take away. Add neutral pairs until you have negatives. |  |
| Then take away negatives. |  |
| Count the number of counters that are left. |  |
| The difference of and is . |
try it
Model the expression:Answer:

Answer:

- subtracting a positive number from a positive number
- subtracting a positive number from a negative number
- subtracting a negative number from a positive number
- subtracting a negative number from a negative number
example
Model each subtraction.Answer:
| 1. | |
| This means take away . | |
| Start with positives. |  |
| Take away positives. |  |
| How many are left? | |
| 2. | |
| This means take away . | |
| Start with negatives. |  |
| You need to take away positives. Add neutral pairs to get positives. | 
 |
| Take away positives. |  |
| How many are left? |  |
| 3. | |
| This means take away . | |
| Start with positives. |  |
| Add neutrals to get negatives to take away. |  |
| Remove negatives. |  |
| How many are left? |  |
| 4. | |
| This means take away . | |
| Start with negatives. |  |
| Take away negatives. |  |
| How many are left? |  |
try it
Model each subtraction. 1. 2. 3. 4.Answer:
1.
 2.
2.
 3.
3.
 4.
4.

Answer:
1.
 2.
2.
 3.
3.
 4.
4.

example
Model each subtraction expression:Answer: Solution
| 1. We start with positives. |  |
| We need to take away positives, but we have only . | |
| Add neutral pairs until there are positives to take away. |  |
| Then take away positives. |  |
| Find the number of counters that are left. There are negatives. |  |
| 2. We start with negatives. |  |
| We need to take away negatives, but we have only . | |
| Add neutral pairs until there are negatives to take away. |  |
| Then take away the negatives. |  |
| Find the number of counters that are left. There are positives. |  |
try it
Model each subtraction expression.Answer:
1.
 2.
2.

Answer:
1.
 2.
2.

