Problem Set 1: Whole Numbers
Practice Makes Perfect
Identify Counting Numbers and Whole Numbers In the following exercises, determine which of the following numbers are ⓐ counting numbers ⓑ whole numbers.- 0, 5, 125
- 0, 5, 125
- 50, 221
- 0, 50, 221
 561
561
 407
407
 Identify the Place Value of a Digit
In the following exercises, find the place value of the given digits.
Identify the Place Value of a Digit
In the following exercises, find the place value of the given digits.
- 9
- 6
- 0
- 7
- 5
- thousands
- hundreds
- tens
- ten thousands
- hundred thousands
- 9
- 3
- 2
- 8
- 7
- 8
- 6
- 4
- 7
- 0
- hundred thousands
- millions
- thousands
- tens
- ten thousands
- 8
- 4
- 2
- 6
- 7
- 390
- 2,930
- 790
- 5,650
- 13,700
- 391,800
- 28,200
- 481,700
- 1,490
- 1,500
- 2,000
- 3,000
- 164,000
- 163,000
Everyday Math
Writing a Check Jorge bought a car for \text{$24,493}. He paid for the car with a check. Write the purchase price in words. Twenty four thousand, four hundred ninety-three dollars Writing a Check Marissa’s kitchen remodeling cost \text{$18,549}. She wrote a check to the contractor. Write the amount paid in words. Buying a Car Jorge bought a car for \text{$24,493}. Round the price to the nearest:- ten dollars
- hundred dollars
- thousand dollars
- ten-thousand dollars
- $24,490
- $24,500
- $24,000
- $20,000
- ten dollars
- hundred dollars
- thousand dollars
- ten-thousand dollars
- billion people
- hundred-million people
- million people
- hundred-million kilometers
- ten-million kilometers
- million kilometers
Writing Exercises
In your own words, explain the difference between the counting numbers and the whole numbers. Answers may vary. The whole numbers are the counting numbers with the inclusion of zero. Give an example from your everyday life where it helps to round numbers.Self Check
1. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. 2. If most of your checks were...
…confidently. Congratulations! You have achieved the
objectives in this section. Reflect on the study skills you
used so that you can continue to use them. What did you do
to become confident of your ability to do these things? Be
specific.
…with some help. This must be addressed quickly because
topics you do not master become potholes in your road to
success. In math, every topic builds upon previous work.
It is important to make sure you have a strong foundation
before you move on. Who can you ask for help? Your
fellow classmates and instructor are good resources. Is
there a place on campus where math tutors are available?
Can your study skills be improved?
…no—I don’t get it! This is a warning sign and you must
not ignore it. You should get help right away or you will
quickly be overwhelmed. See your instructor as soon as you
can to discuss your situation. Together you can come up
with a plan to get you the help you need.
2. If most of your checks were...
…confidently. Congratulations! You have achieved the
objectives in this section. Reflect on the study skills you
used so that you can continue to use them. What did you do
to become confident of your ability to do these things? Be
specific.
…with some help. This must be addressed quickly because
topics you do not master become potholes in your road to
success. In math, every topic builds upon previous work.
It is important to make sure you have a strong foundation
before you move on. Who can you ask for help? Your
fellow classmates and instructor are good resources. Is
there a place on campus where math tutors are available?
Can your study skills be improved?
…no—I don’t get it! This is a warning sign and you must
not ignore it. You should get help right away or you will
quickly be overwhelmed. See your instructor as soon as you
can to discuss your situation. Together you can come up
with a plan to get you the help you need.
Practice Makes Perfect
Use Addition Notation In the following exercises, translate the following from math expressions to words. five plus two; the sum of 5 and 2. thirteen plus eighteen; the sum of 13 and 18. two hundred fourteen plus six hundred forty-two; the sum of 214 and 642 Model Addition of Whole Numbers In the following exercises, model the addition.

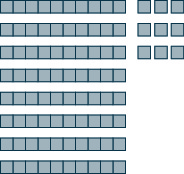
 Add Whole Numbers
In the following exercises, fill in the missing values in each chart.
Add Whole Numbers
In the following exercises, fill in the missing values in each chart.
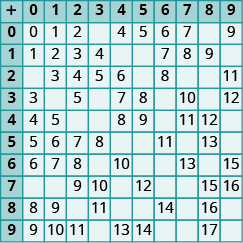
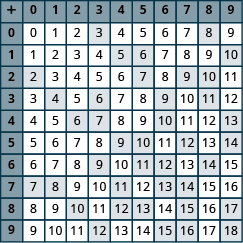
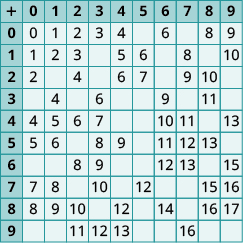
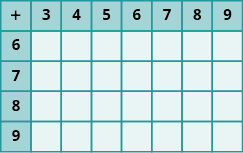
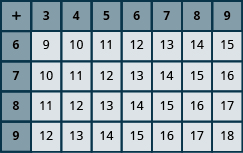
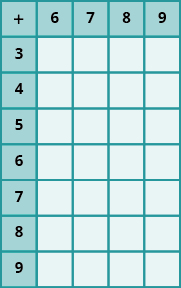
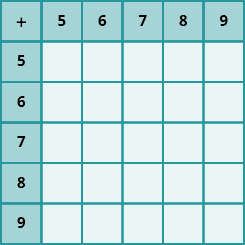
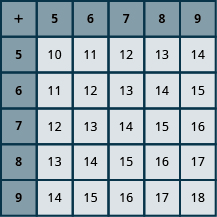
 In the following exercises, add.
In the following exercises, add.
- 13
- 13
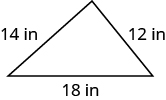 The perimeter of the figure is 44 inches.
The perimeter of the figure is 44 inches.
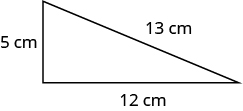
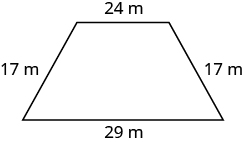
 The perimeter of the figure is 56 meters.
The perimeter of the figure is 56 meters.

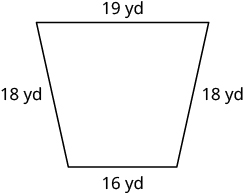 The perimeter of the figure is 71 yards.
The perimeter of the figure is 71 yards.
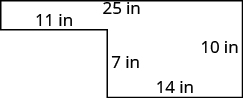
 The perimeter of the figure is 62 feet.
The perimeter of the figure is 62 feet.
Everyday Math
Calories Paulette had a grilled chicken salad, ranch dressing, and a drink for lunch. On the restaurant’s nutrition chart, she saw that each item had the following number of calories: Grilled chicken salad – calories Ranch dressing – calories drink – calories What was the total number of calories of Paulette’s lunch? The total number of calories was 640. Calories Fred had a grilled chicken sandwich, a small order of fries, and a chocolate shake for dinner. The restaurant’s nutrition chart lists the following calories for each item: Grilled chicken sandwich – calories Small fries – calories chocolate shake – calories What was the total number of calories of Fred’s dinner? Test scores A students needs a total of points on five tests to pass a course. The student scored . Did the student pass the course? Yes, he scored 406 points. Elevators The maximum weight capacity of an elevator is pounds. Six men are in the elevator. Their weights are pounds. Is the total weight below the elevators’ maximum capacity?Writing Exercises
How confident do you feel about your knowledge of the addition facts? If you are not fully confident, what will you do to improve your skills? Answers will vary. How have you used models to help you learn the addition facts?Self Check
1. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section.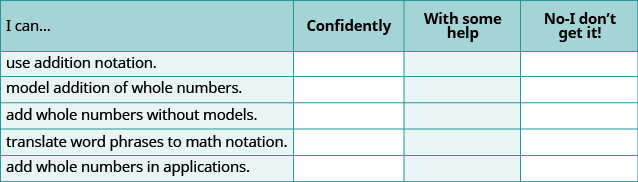 2. After reviewing this checklist, what will you do to become confident for all objectives?
2. After reviewing this checklist, what will you do to become confident for all objectives?
Practice Makes Perfect
Use Subtraction Notation In the following exercises, translate from math notation to words. fifteen minus nine; the difference of fifteen and nine forty-two minus thirty-five; the difference of forty-two and thirty-five hundred seventy-five minus three hundred fifty; the difference of six hundred seventy-five and three hundred fifty Model Subtraction of Whole Numbers In the following exercises, model the subtraction.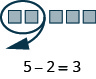
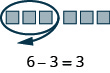
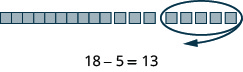
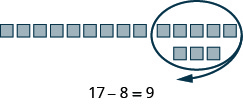

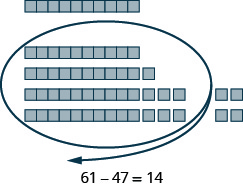 Subtract Whole Numbers
In the following exercises, subtract and then check by adding.
5
8
22
33
123
1,321
28
222
346
3,519
1,186
34,668
Translate Word Phrases to Algebraic Expressions
In the following exercises, translate and simplify.
The difference of and
10 − 3; 7
The difference of and
The difference of and
15 − 4; 11
The difference of and
Subtract from
9 − 6; 3
Subtract from
Subtract from
75 − 28; 47
Subtract from
45 − 20; 25
92 − 67; 25
16 − 12; 4
61 − 38; 23
Mixed Practice
In the following exercises, simplify.
29
72
1,060
22
In the following exercises, translate and simplify.
Seventy more than thirty-five
75 + 35; 110
Sixty more than ninety-three
41 − 13; 28
The difference of and
100 − 76; 24
The difference of and
Subtract Whole Numbers in Applications
In the following exercises, solve.
Temperature The high temperature on June in Las Vegas was degrees and the low temperature was degrees. What was the difference between the high and low temperatures?
The difference between the high and low temperature was 17 degrees
Temperature The high temperature on June in Phoenix was degrees and the low was degrees. What was the difference between the high and low temperatures?
Class size Olivia’s third grade class has children. Last year, her second grade class had children. What is the difference between the number of children in Olivia’s third grade class and her second grade class?
The difference between the third grade and second grade was 13 children.
Class size There are students in the school band and in the school orchestra. What is the difference between the number of students in the band and the orchestra?
Shopping A mountain bike is on sale for \text{$399}. Its regular price is \text{$650}. What is the difference between the regular price and the sale price?
The difference between the regular price and sale price is $251.
Shopping A mattress set is on sale for \text{$755}. Its regular price is \text{$1,600}. What is the difference between the regular price and the sale price?
Savings John wants to buy a laptop that costs \text{$840}. He has \text{$685} in his savings account. How much more does he need to save in order to buy the laptop?
John needs to save $155 more.
Banking Mason had \text{$1,125} in his checking account. He spent \text{$892}. How much money does he have left?
Subtract Whole Numbers
In the following exercises, subtract and then check by adding.
5
8
22
33
123
1,321
28
222
346
3,519
1,186
34,668
Translate Word Phrases to Algebraic Expressions
In the following exercises, translate and simplify.
The difference of and
10 − 3; 7
The difference of and
The difference of and
15 − 4; 11
The difference of and
Subtract from
9 − 6; 3
Subtract from
Subtract from
75 − 28; 47
Subtract from
45 − 20; 25
92 − 67; 25
16 − 12; 4
61 − 38; 23
Mixed Practice
In the following exercises, simplify.
29
72
1,060
22
In the following exercises, translate and simplify.
Seventy more than thirty-five
75 + 35; 110
Sixty more than ninety-three
41 − 13; 28
The difference of and
100 − 76; 24
The difference of and
Subtract Whole Numbers in Applications
In the following exercises, solve.
Temperature The high temperature on June in Las Vegas was degrees and the low temperature was degrees. What was the difference between the high and low temperatures?
The difference between the high and low temperature was 17 degrees
Temperature The high temperature on June in Phoenix was degrees and the low was degrees. What was the difference between the high and low temperatures?
Class size Olivia’s third grade class has children. Last year, her second grade class had children. What is the difference between the number of children in Olivia’s third grade class and her second grade class?
The difference between the third grade and second grade was 13 children.
Class size There are students in the school band and in the school orchestra. What is the difference between the number of students in the band and the orchestra?
Shopping A mountain bike is on sale for \text{$399}. Its regular price is \text{$650}. What is the difference between the regular price and the sale price?
The difference between the regular price and sale price is $251.
Shopping A mattress set is on sale for \text{$755}. Its regular price is \text{$1,600}. What is the difference between the regular price and the sale price?
Savings John wants to buy a laptop that costs \text{$840}. He has \text{$685} in his savings account. How much more does he need to save in order to buy the laptop?
John needs to save $155 more.
Banking Mason had \text{$1,125} in his checking account. He spent \text{$892}. How much money does he have left?
Everyday Math
Road trip Noah was driving from Philadelphia to Cincinnati, a distance of miles. He drove miles, stopped for gas, and then drove another miles before lunch. How many more miles did he have to travel? 157 miles Test Scores Sara needs points to pass her course. She scored on her first four tests. How many more points does Sara need to pass the course?Writing Exercises
Explain how subtraction and addition are related. Answers may vary. How does knowing addition facts help you to subtract numbers?Self Check
1. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. 2. What does this checklist tell you about your mastery of this section? What steps will you take to improve?
2. What does this checklist tell you about your mastery of this section? What steps will you take to improve?
Practice Makes Perfect
Use Multiplication Notation In the following exercises, translate from math notation to words. four times seven; the product of four and seven five times twelve; the product of five and twelve ten times twenty-five; the product of ten and twenty-five forty-two times thirty-three; the product of forty-two and thirty-three Model Multiplication of Whole Numbers In the following exercises, model the multiplication.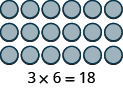
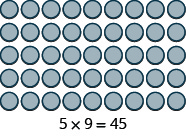 Multiply Whole Numbers
In the following exercises, fill in the missing values in each chart.
Multiply Whole Numbers
In the following exercises, fill in the missing values in each chart.


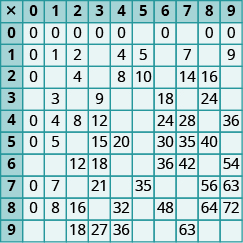
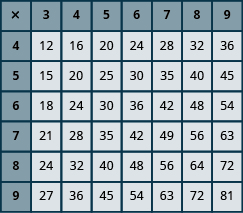


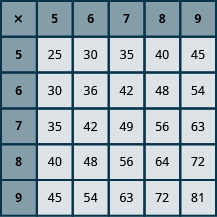
 In the following exercises, multiply.
0
0
43
28
240,055
In the following exercises, multiply.
0
0
43
28
240,055
- 42
- 42
Everyday Math
Stock market Javier owns 300 shares of stock in one company. On Tuesday, the stock price rose \text{$}12 per share. How much money did Javier’s portfolio gain? Javier’s portfolio gained $3,600. Salary Carlton got a \text{$}200 raise in each paycheck. He gets paid 24 times a year. How much higher is his new annual salary?Writing Exercises
How confident do you feel about your knowledge of the multiplication facts? If you are not fully confident, what will you do to improve your skills? Answers will vary. How have you used models to help you learn the multiplication facts?Self Check
1. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. 2. On a scale of 1–10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
2. On a scale of 1–10, how would you rate your mastery of this section in light of your responses on the checklist? How can you improve this?
Practice Makes Perfect
Use Division Notation In the following exercises, translate from math notation to words. fifty-four divided by nine; the quotient of fifty-four and nine thirty-two divided by eight; the quotient of thirty-two and eight forty-eight divided by six; the quotient of forty-eight and six sixty-three divided by seven; the quotient of sixty-three and seven Model Division of Whole Numbers In the following exercises, model the division.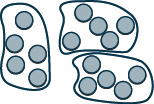
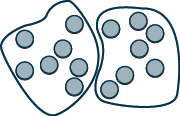
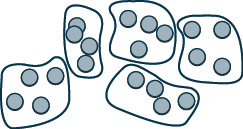
 Divide Whole Numbers
In the following exercises, divide. Then check by multiplying.
9
9
7
9
9
5
1
1
23
19
0
undefined
undefined
0
24
12
93
132
871
7,831
2,403
901
704
10,209
352 R6
6,913 R1
86,234 R4
43,338 R2
382 R5
849
96
1,986 R17
Mixed Practice
In the following exercises, simplify.
3,060
72
1,060
35
Translate Word Phrases to Algebraic Expressions
In the following exercises, translate and simplify.
the quotient of and
45 ÷ 15; 3
the quotient of and
the quotient of and
288 ÷ 24; 12
the quotient of and
Divide Whole Numbers in Applications
In the following exercises, solve.
Trail mix Ric bought ounces of trail mix. He wants to divide it into small bags, with ounces of trail mix in each bag. How many bags can Ric fill?
Ric can fill 32 bags.
Crackers Evie bought a ounce box of crackers. She wants to divide it into bags with ounces of crackers in each bag. How many bags can Evie fill?
Astronomy class There are students in an astronomy class. The professor assigns them into groups of . How many groups of students are there?
There are 25 groups.
Flower shop Melissa’s flower shop got a shipment of roses. She wants to make bouquets of roses each. How many bouquets can Melissa make?
Baking One roll of plastic wrap is feet long. Marta uses feet of plastic wrap to wrap each cake she bakes. How many cakes can she wrap from one roll?
Marta can wrap 16 cakes from 1 roll.
Dental floss One package of dental floss is feet long. Brian uses feet of dental floss every day. How many days will one package of dental floss last Brian?
Mixed Practice
In the following exercises, solve.
Miles per gallon Susana’s hybrid car gets miles per gallon. Her son’s truck gets miles per gallon. What is the difference in miles per gallon between Susana’s car and her son’s truck?
The difference is 28 miles per gallon.
Distance Mayra lives miles from her mother’s house and miles from her mother-in-law’s house. How much farther is Mayra from her mother-in-law’s house than from her mother’s house?
Field trip The students in a Geology class will go on a field trip, using the college’s vans. Each van can hold students. How many vans will they need for the field trip?
They will need 5 vans for the field trip
Potting soil Aki bought a ounce bag of potting soil. How many ounce pots can he fill from the bag?
Hiking Bill hiked miles on the first day of his backpacking trip, miles the second day, miles the third day, and miles the fourth day. What is the total number of miles Bill hiked?
Bill hiked 50 miles
Reading Last night Emily read pages in her Business textbook, pages in her History text, pages in her Psychology text, and pages in her math text. What is the total number of pages Emily read?
Patients LaVonne treats patients each day in her dental office. Last week she worked days. How many patients did she treat last week?
LaVonne treated 48 patients last week.
Scouts There are boys in Dave’s scout troop. At summer camp, each boy earned merit badges. What was the total number of merit badges earned by Dave’s scout troop at summer camp?
Divide Whole Numbers
In the following exercises, divide. Then check by multiplying.
9
9
7
9
9
5
1
1
23
19
0
undefined
undefined
0
24
12
93
132
871
7,831
2,403
901
704
10,209
352 R6
6,913 R1
86,234 R4
43,338 R2
382 R5
849
96
1,986 R17
Mixed Practice
In the following exercises, simplify.
3,060
72
1,060
35
Translate Word Phrases to Algebraic Expressions
In the following exercises, translate and simplify.
the quotient of and
45 ÷ 15; 3
the quotient of and
the quotient of and
288 ÷ 24; 12
the quotient of and
Divide Whole Numbers in Applications
In the following exercises, solve.
Trail mix Ric bought ounces of trail mix. He wants to divide it into small bags, with ounces of trail mix in each bag. How many bags can Ric fill?
Ric can fill 32 bags.
Crackers Evie bought a ounce box of crackers. She wants to divide it into bags with ounces of crackers in each bag. How many bags can Evie fill?
Astronomy class There are students in an astronomy class. The professor assigns them into groups of . How many groups of students are there?
There are 25 groups.
Flower shop Melissa’s flower shop got a shipment of roses. She wants to make bouquets of roses each. How many bouquets can Melissa make?
Baking One roll of plastic wrap is feet long. Marta uses feet of plastic wrap to wrap each cake she bakes. How many cakes can she wrap from one roll?
Marta can wrap 16 cakes from 1 roll.
Dental floss One package of dental floss is feet long. Brian uses feet of dental floss every day. How many days will one package of dental floss last Brian?
Mixed Practice
In the following exercises, solve.
Miles per gallon Susana’s hybrid car gets miles per gallon. Her son’s truck gets miles per gallon. What is the difference in miles per gallon between Susana’s car and her son’s truck?
The difference is 28 miles per gallon.
Distance Mayra lives miles from her mother’s house and miles from her mother-in-law’s house. How much farther is Mayra from her mother-in-law’s house than from her mother’s house?
Field trip The students in a Geology class will go on a field trip, using the college’s vans. Each van can hold students. How many vans will they need for the field trip?
They will need 5 vans for the field trip
Potting soil Aki bought a ounce bag of potting soil. How many ounce pots can he fill from the bag?
Hiking Bill hiked miles on the first day of his backpacking trip, miles the second day, miles the third day, and miles the fourth day. What is the total number of miles Bill hiked?
Bill hiked 50 miles
Reading Last night Emily read pages in her Business textbook, pages in her History text, pages in her Psychology text, and pages in her math text. What is the total number of pages Emily read?
Patients LaVonne treats patients each day in her dental office. Last week she worked days. How many patients did she treat last week?
LaVonne treated 48 patients last week.
Scouts There are boys in Dave’s scout troop. At summer camp, each boy earned merit badges. What was the total number of merit badges earned by Dave’s scout troop at summer camp?
Writing Exercises
Explain how you use the multiplication facts to help with division. Answers may vary. Using multiplication facts can help you check your answers once you’ve finished division. Oswaldo divided by and said his answer was with a remainder of . How can you check to make sure he is correct?Everyday Math
Contact lenses Jenna puts in a new pair of contact lenses every days. How many pairs of contact lenses does she need for days? Jenna uses 26 pairs of contact lenses, but there is 1 day left over, so she needs 27 pairs for 365 days. Cat food One bag of cat food feeds Lara’s cat for days. How many bags of cat food does Lara need for days?Self Check
1. After completing the exercises, use this checklist to evaluate your mastery of the objectives of this section. 2. Overall, after looking at the checklist, do you think you are well-prepared for the next Chapter? Why or why not?
2. Overall, after looking at the checklist, do you think you are well-prepared for the next Chapter? Why or why not?
Chapter Review Exercises
Introduction to Whole Numbers
Identify Counting Numbers and Whole Numbers In the following exercises, determine which of the following are (a) counting numbers (b) whole numbers.- 2, 99
- 0, 2, 99
- 4, 90
- 0, 4, 90
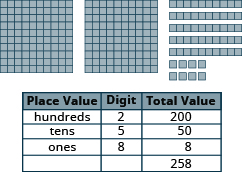 104
Identify the Place Value of a Digit
In the following exercises, find the place value of the given digits.
104
Identify the Place Value of a Digit
In the following exercises, find the place value of the given digits.
- tens
- hundred thousands
- ones
- thousands
- ten thousands
Add Whole Numbers
Use Addition Notation In the following exercises, translate the following from math notation to words. four plus three; the sum of four and three five hundred seventy-one plus six hundred twenty-nine; the sum of five hundred seventy-one and six hundred twenty-nine Model Addition of Whole Numbers In the following exercises, model the addition. Add Whole Numbers
In the following exercises, fill in the missing values in each chart.
Add Whole Numbers
In the following exercises, fill in the missing values in each chart.
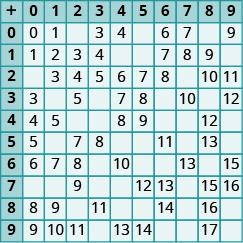
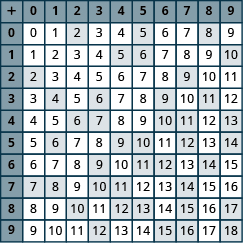
 In the following exercises, add.
In the following exercises, add.
- 2.
- 2.
- 2.
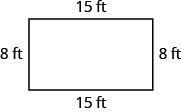 46 feet
46 feet
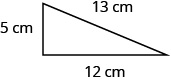
Subtract Whole Numbers
Use Subtraction Notation In the following exercises, translate the following from math notation to words. fourteen minus five; the difference of fourteen and five three hundred fifty-one minus two hundred forty-nine; the difference between three hundred fifty-one and two hundred forty-nine Model Subtraction of Whole Numbers In the following exercises, model the subtraction. Subtract Whole Numbers
In the following exercises, subtract and then check by adding.
3
14
23
322
380
9,985
Translate Word Phrases to Math Notation
In the following exercises, translate and simplify.
the difference of nineteen and thirteen
19 − 13; 6
subtract sixty-five from one hundred
seventy-four decreased by eight
74 − 8; 66
twenty-three less than forty-one
Subtract Whole Numbers in Applications
In the following exercises, solve.
Temperature The high temperature in Peoria one day was degrees Fahrenheit and the low temperature was degrees Fahrenheit. What was the difference between the high and low temperatures?
58 degrees Fahrenheit
Savings Lynn wants to go on a cruise that costs \text{$2,485.} She has \text{$948} in her vacation savings account. How much more does she need to save in order to pay for the cruise?
Subtract Whole Numbers
In the following exercises, subtract and then check by adding.
3
14
23
322
380
9,985
Translate Word Phrases to Math Notation
In the following exercises, translate and simplify.
the difference of nineteen and thirteen
19 − 13; 6
subtract sixty-five from one hundred
seventy-four decreased by eight
74 − 8; 66
twenty-three less than forty-one
Subtract Whole Numbers in Applications
In the following exercises, solve.
Temperature The high temperature in Peoria one day was degrees Fahrenheit and the low temperature was degrees Fahrenheit. What was the difference between the high and low temperatures?
58 degrees Fahrenheit
Savings Lynn wants to go on a cruise that costs \text{$2,485.} She has \text{$948} in her vacation savings account. How much more does she need to save in order to pay for the cruise?
Multiply Whole Numbers
Use Multiplication Notation In the following exercises, translate from math notation to words. eight times five the product of eight and five ten times ninety-five; the product of ten and ninety-five Model Multiplication of Whole Numbers In the following exercises, model the multiplication. Multiply Whole Numbers
In the following exercises, fill in the missing values in each chart.
Multiply Whole Numbers
In the following exercises, fill in the missing values in each chart.
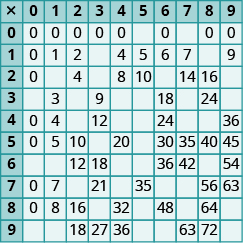
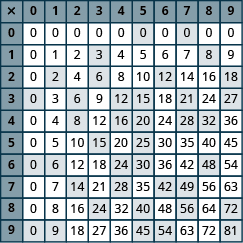
 In the following exercises, multiply.
0
99
ⓐ ⓑ
In the following exercises, multiply.
0
99
ⓐ ⓑ
- ⓐ 28
- ⓑ 28
Divide Whole Numbers
Use Division Notation Translate from math notation to words. fifty-four divided by nine; the quotient of fifty-four and nine seventy-two divided by eight; the quotient of seventy-two and eight Model Division of Whole Numbers In the following exercises, model. Divide Whole Numbers
In the following exercises, divide. Then check by multiplying.
7
13
97
undefined
638
300 R5
Translate Word Phrases to Math Notation
In the following exercises, translate and simplify.
the quotient of and
64 ÷ 16; 4
the quotient of and
Divide Whole Numbers in Applications
In the following exercises, solve.
Ribbon One spool of ribbon is feet. Lizbeth uses feet of ribbon for each gift basket that she wraps. How many gift baskets can Lizbeth wrap from one spool of ribbon?
9 baskets
Juice One carton of fruit juice is ounces. How many ounce cups can Shayla fill from one carton of juice?
Divide Whole Numbers
In the following exercises, divide. Then check by multiplying.
7
13
97
undefined
638
300 R5
Translate Word Phrases to Math Notation
In the following exercises, translate and simplify.
the quotient of and
64 ÷ 16; 4
the quotient of and
Divide Whole Numbers in Applications
In the following exercises, solve.
Ribbon One spool of ribbon is feet. Lizbeth uses feet of ribbon for each gift basket that she wraps. How many gift baskets can Lizbeth wrap from one spool of ribbon?
9 baskets
Juice One carton of fruit juice is ounces. How many ounce cups can Shayla fill from one carton of juice?
Chapter Practice Test
Determine which of the following numbers are- counting numbers
- whole numbers.
- 4, 87
- 0, 4, 8
- six hundred thirteen
- fifty-five thousand two hundred eight
- 613
- 55,208
