Subtracting Fractions With Common Denominators
Learning Outcomes
- Use fraction circles to find the difference between two fractions with like denominators
- Subtract fractions with a like denominator without fraction circles
- Subtract fractions with like denominators that contain variables
Model Fraction Subtraction
Subtracting two fractions with common denominators is much like adding fractions. Think of a pizza that was cut into slices. Suppose five pieces are eaten for dinner. This means that, after dinner, there are seven pieces (or of the pizza) left in the box. If Leonardo eats of these remaining pieces (or of the pizza), how much is left? There would be pieces left (or of the pizza). Let’s use fraction circles to model the same example, . Start with seven pieces. Take away two pieces. How many twelfths are left?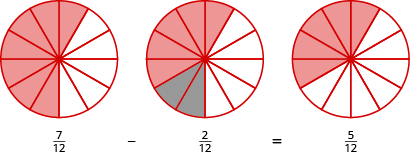 Again, we have five twelfths, .
Doing the Manipulative Mathematics activity "Model Fraction Subtraction" will help you develop a better understanding of subtracting fractions.
Again, we have five twelfths, .
Doing the Manipulative Mathematics activity "Model Fraction Subtraction" will help you develop a better understanding of subtracting fractions.
Example
Use fraction circles to find the difference: Solution: Start with four pieces. Take away one piece. Count how many fifths are left. There are three pieces left.
Try It
#146190 [ohm_question height="270"]146190[/ohm_question]Subtract Fractions with a Common Denominator
We subtract fractions with a common denominator in much the same way as we add fractions with a common denominator.Fraction Subtraction
If are numbers where , then To subtract fractions with a common denominators, we subtract the numerators and place the difference over the common denominator.Example
Find the difference:Answer: Solution:
| Subtract the numerators and place the difference over the common denominator. | |
| Simplify the numerator. | |
| Simplify the fraction by removing common factors. |
Try It
#146191 [ohm_question height="270"]146191[/ohm_question]Example
Find the difference:Answer: Solution:
| Subtract the numerators and place the difference over the common denominator. |
Try it
#146192 [ohm_question height="270"]146192[/ohm_question]Example
Find the difference:Answer: Solution: Remember, the fraction can be written as .
| Subtract the numerators. | |
| Simplify. | |
| Rewrite with the negative sign in front of the fraction. |
Try It
#146249 [ohm_question height="270"]146249[/ohm_question]Example
Simplify:Answer: Solution:
| Combine the numerators over the common denominator. | |
| Simplify the numerator, working left to right. | |
| Subtract the terms in the numerator. | |
| Rewrite with the negative sign in front of the fraction. |
