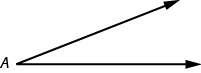
∠A is the angle with vertex at
point A.
We measure angles in degrees, and use the symbol
Supplementary and Complementary Angles
If the sum of the measures of two angles is
180∘, then the angles are supplementary.
If angle
A and angle
B are supplementary, then
m\angleA+m\angleB=180∘.
If the sum of the measures of two angles is
90∘, then the angles are complementary.
If angle
A and angle
B are complementary, then
m\angleA+m\angleB=90∘.
In this section and the next, you will be introduced to some common geometry formulas. We will adapt our Problem Solving Strategy for Geometry Applications. The geometry formula will name the variables and give us the equation to solve.
In addition, since these applications will all involve geometric shapes, it will be helpful to draw a figure and then label it with the information from the problem. We will include this step in the Problem Solving Strategy for Geometry Applications.
example
An angle measures
40∘. Find 1. its supplement, and 2. its complement.
Solution
| 1. |
|
| Step 1. Read the problem. Draw the figure and label it with the given information. |
 |
| Step 2. Identify what you are looking for. |
The supplement of a 40°angle. |
| Step 3. Name. Choose a variable to represent it. |
Let s=the measure of the supplement. |
| Step 4. Translate.
Write the appropriate formula for the situation and substitute in the given information. |
m∠A+m∠B=180
s+40=180 |
| Step 5. Solve the equation. |
s=140 |
| Step 6. Check:
140+40=?180
180=180✓ |
|
| Step 7. Answer the question. |
The supplement of the 40°angle is 140°. |
| 2. |
|
| Step 1. Read the problem. Draw the figure and label it with the given information. |
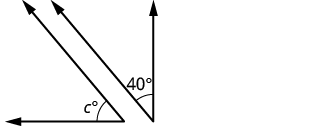 |
| Step 2. Identify what you are looking for. |
| The complement of a 40°angle. |
|
| Step 3. Name. Choose a variable to represent it. |
Let c=the measure of the complement. |
| Step 4. Translate.
Write the appropriate formula for the situation and substitute in the given information. |
m∠A+m∠B=90 |
| Step 5. Solve the equation. |
c+40=90
c=50 |
| Step 6. Check:
50+40=?90
90=90✓ |
|
| Step 7. Answer the question. |
The complement of the 40°angle is 50°. |
Exercises
Two angles are supplementary. The larger angle is
30∘ more than the smaller angle. Find the measure of both angles.
Answer:
Solution:
| Step 1. Read the problem. Draw the figure and label it with the given information. |
 |
| Step 2. Identify what you are looking for. |
The measures of both angles. |
| Step 3. Name. Choose a variable to represent it.
The larger angle is 30° more than the smaller angle. |
Let a= measure of smaller angle
a+30= measure of larger angle |
| Step 4. Translate.
Write the appropriate formula and substitute. |
m∠A+m∠B=180 |
| Step 5. Solve the equation. |
(a+30)+a=180
2a+30=180
2a=150
a=75measure of smaller angle.
a+30measure of larger angle.
75+30
105 |
| Step 6. Check:
m∠A+m∠B=180
75+105=?180
180=180✓ |
|
| Step 7. Answer the question. |
The measures of the angle are 75°and 105°. |
 An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle and the common endpoint is called the vertex. An angle is named by its vertex. In the image below, is the angle with vertex at point . The measure of is written .
An angle is formed by two rays that share a common endpoint. Each ray is called a side of the angle and the common endpoint is called the vertex. An angle is named by its vertex. In the image below, is the angle with vertex at point . The measure of is written .


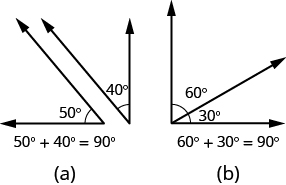
 If the sum of the measures of two angles is , then the angles are complementary angles. In the images below, each pair of angles is complementary, because their measures add to . Each angle is the complement of the other.
The sum of the measures of complementary angles is .
If the sum of the measures of two angles is , then the angles are complementary angles. In the images below, each pair of angles is complementary, because their measures add to . Each angle is the complement of the other.
The sum of the measures of complementary angles is .