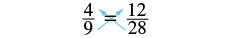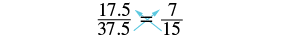We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Prealgebra

Writing Proportions

Learning Outcomes

  • Given a statement, write a proportion
  • Given an equation, determine whether it is a proportion
 

In the section on Ratios and Rates we saw some ways they are used in our daily lives. When two ratios or rates are equal, the equation relating them is called a proportion.

Proportion

A proportion is an equation of the form [latex]\frac{a}{b}=\frac{c}{d}[/latex], where [latex]b\ne 0,d\ne 0[/latex]. The proportion states two ratios or rates are equal. The proportion is read [latex]\text{"}a[/latex] is to [latex]b[/latex], as [latex]c[/latex] is to [latex]d\text{".}[/latex]
  The equation [latex]\frac{1}{2}=\frac{4}{8}[/latex] is a proportion because the two fractions are equal. The proportion [latex]\frac{1}{2}=\frac{4}{8}[/latex] is read [latex]\text{"}1[/latex] is to [latex]2[/latex] as [latex]4[/latex] is to [latex]8\text{".}[/latex] If we compare quantities with units, we have to be sure we are comparing them in the right order. For example, in the proportion [latex]\frac{\text{20 students}}{\text{1 teacher}}=\frac{\text{60 students}}{\text{3 teachers}}[/latex] we compare the number of students to the number of teachers. We put students in the numerators and teachers in the denominators.  

example

Write each sentence as a proportion: ⓐ [latex]3[/latex] is to [latex]7[/latex] as [latex]15[/latex] is to [latex]35[/latex]. ⓑ [latex]5[/latex] hits in [latex]8[/latex] at bats is the same as [latex]30[/latex] hits in [latex]48[/latex] at-bats. ⓒ [latex]\text{\$1.50}[/latex] for [latex]6[/latex] ounces is equivalent to [latex]\text{\$2.25}[/latex] for [latex]9[/latex] ounces. Solution
ⓐ
[latex]3[/latex] is to [latex]7[/latex] as [latex]15[/latex] is to [latex]35[/latex].
Write as a proportion. [latex]\frac{3}{7}=\frac{15}{35}[/latex]
ⓑ
[latex]5[/latex] hits in [latex]8[/latex] at-bats is the same as [latex]30[/latex] hits in [latex]48[/latex] at-bats.
Write each fraction to compare hits to at-bats. [latex]\frac{\text{hits}}{\text{at-bats}}=\frac{\text{hits}}{\text{at-bats}}[/latex]
Write as a proportion. [latex]\frac{5}{8}=\frac{30}{48}[/latex]
ⓒ
[latex]\text{\$1.50}[/latex] for [latex]6[/latex] ounces is equivalent to [latex]\text{\$2.25}[/latex] for [latex]9[/latex] ounces.
Write each fraction to compare dollars to ounces. [latex]\frac{$}{\text{ounces}}=\frac{$}{\text{ounces}}[/latex]
Write as a proportion. [latex]\frac{1.50}{6}=\frac{2.25}{9}[/latex]
 

try it

  [ohm_question]146798[/ohm_question] [ohm_question]146799[/ohm_question]
  Look at the proportions [latex]\frac{1}{2}=\frac{4}{8}[/latex] and [latex]\frac{2}{3}=\frac{6}{9}[/latex]. From our work with equivalent fractions we know these equations are true. But how do we know if an equation is a proportion with equivalent fractions if it contains fractions with larger numbers? To determine if a proportion is true, we find the cross products of each proportion. To find the cross products, we multiply each denominator with the opposite numerator (diagonally across the equal sign). The results are called a cross products because of the cross formed. The cross products of a proportion are equal. The figure shows cross multiplication of two proportions. There is the proportion 1 is to 2 as 4 is to 8. Arrows are shown diagonally across the equal sign to show cross products. The equations formed by cross multiplying are 8 · 1 = 8 and 2 · 4 = 8. There is the proportion 2 is to 3 as 6 is to 9. Arrows are shown diagonally across the equal sign to show cross products. The equations formed by cross multiplying are 9 · 2 = 18 and 3 · 6 = 18. Cross Products of a Proportion For any proportion of the form [latex]\frac{a}{b}=\frac{c}{d}[/latex], where [latex]b\ne 0,d\ne 0[/latex], its cross products are equal. No Alt Text Cross products can be used to test whether a proportion is true. To test whether an equation makes a proportion, we find the cross products. If they are the equal, we have a proportion.

example

Determine whether each equation is a proportion: ⓐ [latex]\frac{4}{9}=\frac{12}{28}[/latex] ⓑ [latex]\frac{17.5}{37.5}=\frac{7}{15}[/latex]

Answer: Solution To determine if the equation is a proportion, we find the cross products. If they are equal, the equation is a proportion.

ⓐ
[latex]\frac{4}{9}=\frac{12}{28}[/latex]
Find the cross products. [latex]28\cdot 4=1129\cdot 12=108[/latex] .
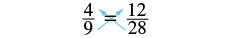Since the cross products are not equal, [latex]28\cdot 4\ne 9\cdot 12[/latex], the equation is not a proportion.
ⓑ
[latex]\frac{17.5}{37.5}=\frac{7}{15}[/latex]
Find the cross products. [latex]15\cdot 17.5=262.537.5\cdot 7=262.5[/latex] .
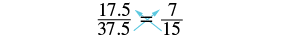Since the cross products are equal, [latex]15\cdot 17.5=37.5\cdot 7[/latex], the equation is a proportion.

 

try it

[ohm_question]146809[/ohm_question]
 

NEW VIDEO REQUEST

Licenses & Attributions

CC licensed content, Original

  • Question ID 146809, 146808, 146807. Authored by: Lumen Learning. License: CC BY: Attribution.

CC licensed content, Specific attribution