Solutions 22: Graphs of Exponential Functions
Solutions to Try Its
1. The domain is ; the range is ; the horizontal asymptote is .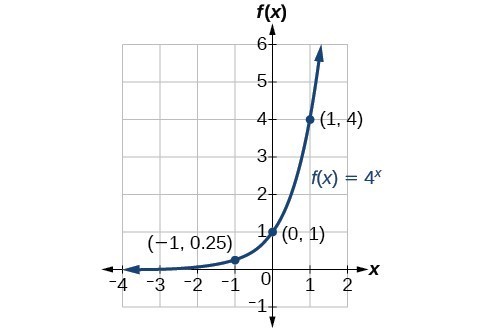 2. The domain is ; the range is ; the horizontal asymptote is y = 3.
2. The domain is ; the range is ; the horizontal asymptote is y = 3.
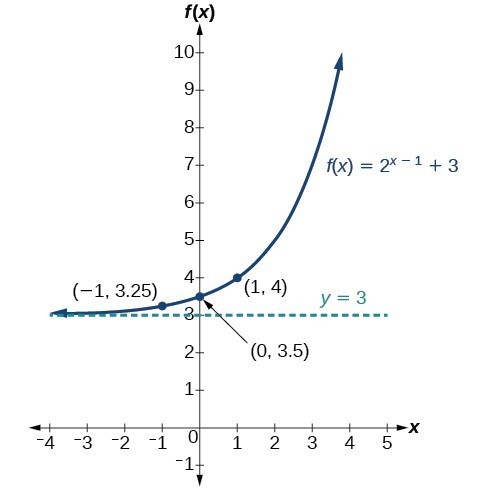 3.
4. The domain is ; the range is ; the horizontal asymptote is .
3.
4. The domain is ; the range is ; the horizontal asymptote is .
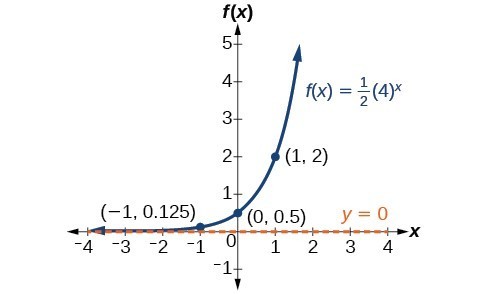 5. The domain is ; the range is ; the horizontal asymptote is .
5. The domain is ; the range is ; the horizontal asymptote is .
 6. ; the domain is ; the range is ; the horizontal asymptote is .
6. ; the domain is ; the range is ; the horizontal asymptote is .
Solutions to Odd-Numbered Exercises
1. An asymptote is a line that the graph of a function approaches, as x either increases or decreases without bound. The horizontal asymptote of an exponential function tells us the limit of the function’s values as the independent variable gets either extremely large or extremely small. 3. ; y-intercept: ; Domain: all real numbers; Range: all real numbers greater than 0. 5. ; y-intercept: ; Domain: all real numbers; Range: all real numbers less than 7. 7. ; y-intercept: ; Domain: all real numbers; Range: all real numbers greater than 0. 9. y-intercept: 11.
11.
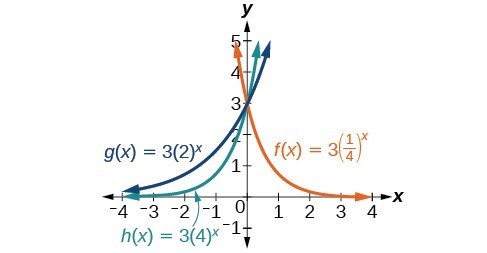 13. B
15. A
17. E
19. D
21. C
23.
13. B
15. A
17. E
19. D
21. C
23.
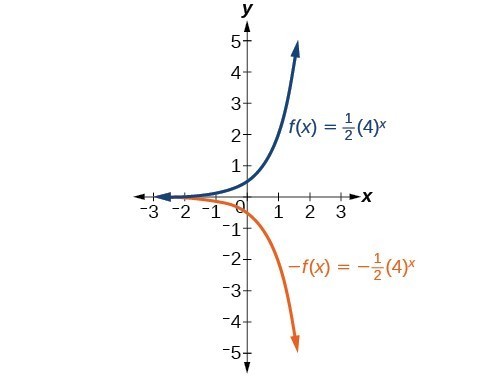 25.
25.
 27. Horizontal asymptote: ; Domain: all real numbers; Range: all real numbers strictly greater than 3.
27. Horizontal asymptote: ; Domain: all real numbers; Range: all real numbers strictly greater than 3.
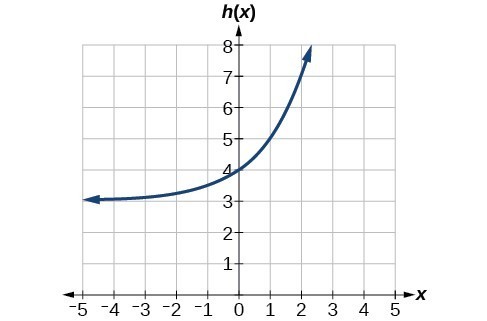 29. As , ;
As ,
31. As , ;
As ,
33.
35.
37.
39.
41.
43.
45.
47.
49.
51. The graph of is the reflection about the y-axis of the graph of ; For any real number and function , the graph of is the the reflection about the y-axis, .
53. The graphs of and are the same and are a horizontal shift to the right of the graph of ; For any real number n, real number , and function , the graph of is the horizontal shift .
29. As , ;
As ,
31. As , ;
As ,
33.
35.
37.
39.
41.
43.
45.
47.
49.
51. The graph of is the reflection about the y-axis of the graph of ; For any real number and function , the graph of is the the reflection about the y-axis, .
53. The graphs of and are the same and are a horizontal shift to the right of the graph of ; For any real number n, real number , and function , the graph of is the horizontal shift .