Solutions for Sum and Difference Identities
Solutions to Try Its
1. 2. 3. 4. 5.Solutions to Odd-Numbered Answers
1. The cofunction identities apply to complementary angles. Viewing the two acute angles of a right triangle, if one of those angles measures , the second angle measures . Then . The same holds for the other cofunction identities. The key is that the angles are complementary. 3. , so is odd. , so is even. 5. 7. 9. 11. 13. 15. 17. 19. 21. 23. 25.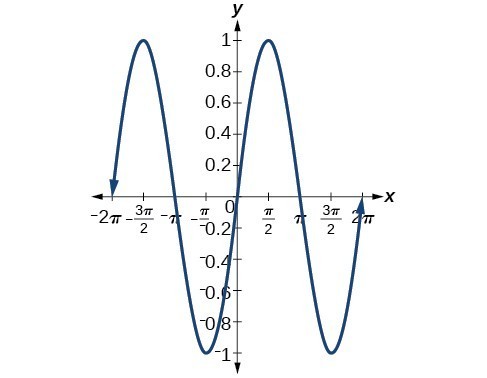 27.
27.
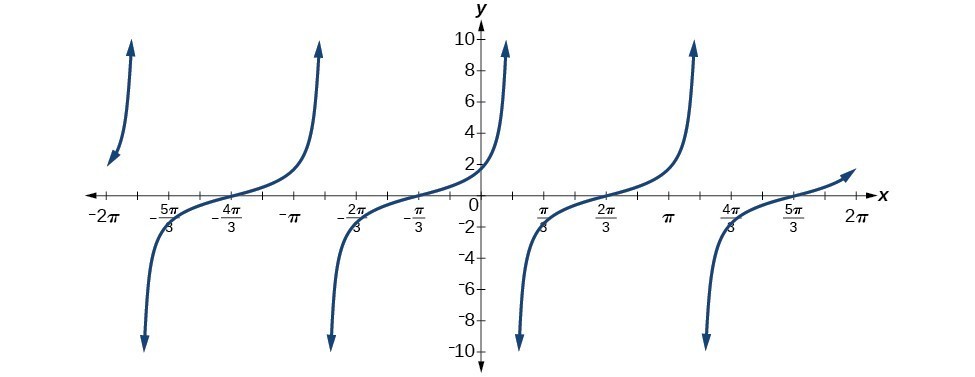 29.
29.
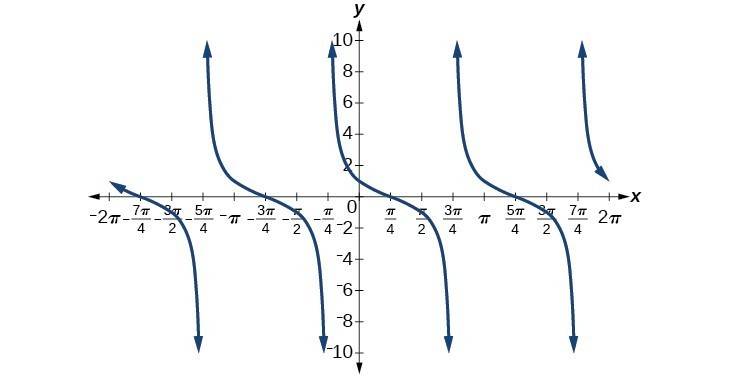 31.
31.
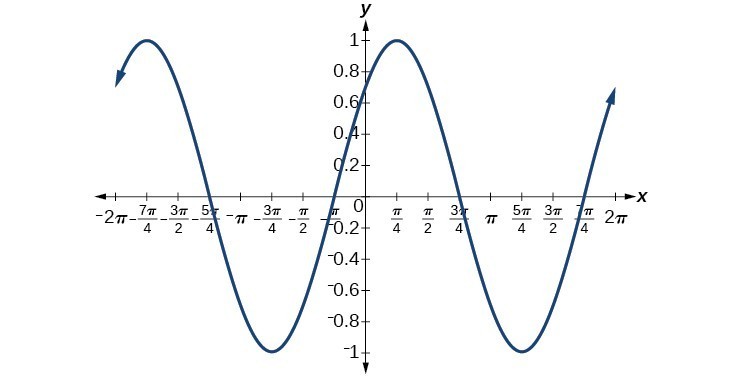 33. They are the same.
35. They are the different, try .
37. They are the same.
39. They are the different, try .
41. They are different, try .
43.
45. , or 0.9659
47.
49.
51.
53. True
55. True. Note that and expand the right hand side.
33. They are the same.
35. They are the different, try .
37. They are the same.
39. They are the different, try .
41. They are different, try .
43.
45. , or 0.9659
47.
49.
51.
53. True
55. True. Note that and expand the right hand side.