Logistic Growth
In our basic exponential growth scenario, we had a recursive equation of the form Pn = Pn-1 + r Pn-1 In a confined environment, however, the growth rate may not remain constant. In a lake, for example, there is some maximum sustainable population of fish, also called a carrying capacity.
Carrying Capacity
The carrying capacity, or maximum sustainable population, is the largest population that an environment can support.
For our fish, the carrying capacity is the largest population that the resources in the lake can sustain. If the population in the lake is far below the carrying capacity, then we would expect the population to grow essentially exponentially. However, as the population approaches the carrying capacity, there will be a scarcity of food and space available, and the growth rate will decrease. If the population exceeds the carrying capacity, there won’t be enough resources to sustain all the fish and there will be a negative growth rate, causing the population to decrease back to the carrying capacity.
If the carrying capacity was 5000, the growth rate might vary something like that in the graph shown. Note that this is a linear equation with intercept at 0.1 and slope , so we could write an equation for this adjusted growth rate as:
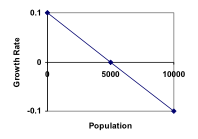 radjusted =
Substituting this in to our original exponential growth model for r gives
radjusted =
Substituting this in to our original exponential growth model for r gives
Logistic Growth
If a population is growing in a constrained environment with carrying capacity K, and absent constraint would grow exponentially with growth rate r, then the population behavior can be described by the logistic growth model:
Unlike linear and exponential growth, logistic growth behaves differently if the populations grow steadily throughout the year or if they have one breeding time per year. The recursive formula provided above models generational growth, where there is one breeding time per year (or, at least a finite number); there is no explicit formula for this type of logistic growth.
Example 15
A forest is currently home to a population of 200 rabbits. The forest is estimated to be able to sustain a population of 2000 rabbits. Absent any restrictions, the rabbits would grow by 50% per year. Predict the future population using the logistic growth model. Modeling this with a logistic growth model, r = 0.50, K = 2000, and P0 = 200. Calculating the next year: We can use this to calculate the following year: A calculator was used to compute several more values:| n | 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| Pn | 200 | 290 | 414 | 578 | 784 | 1022 | 1272 | 1503 | 1690 | 1821 | 1902 |

