Find or evaluate the inverse of a function
Once we have a one-to-one function, we can evaluate its inverse at specific inverse function inputs or construct a complete representation of the inverse function in many cases.
Inverting Tabular Functions
Suppose we want to find the inverse of a function represented in table form. Remember that the domain of a function is the range of the inverse and the range of the function is the domain of the inverse. So we need to interchange the domain and range.
Each row (or column) of inputs becomes the row (or column) of outputs for the inverse function. Similarly, each row (or column) of outputs becomes the row (or column) of inputs for the inverse function.
Example 5: Interpreting the Inverse of a Tabular Function
A function is given below, showing distance in miles that a car has traveled in minutes. Find and interpret .
| 30 | 50 | 70 | 90 | |
| 20 | 40 | 60 | 70 |
Solution
The inverse function takes an output of and returns an input for . So in the expression , 70 is an output value of the original function, representing 70 miles. The inverse will return the corresponding input of the original function , 90 minutes, so . The interpretation of this is that, to drive 70 miles, it took 90 minutes.
Alternatively, recall that the definition of the inverse was that if , then . By this definition, if we are given , then we are looking for a value so that . In this case, we are looking for a so that , which is when .
Try It 5
Using the table below, find and interpret (a) , and (b) .
| 30 | 50 | 60 | 70 | 90 | |
| 20 | 40 | 50 | 60 | 70 |
Evaluating the Inverse of a Function, Given a Graph of the Original Function
We saw in Functions and Function Notation that the domain of a function can be read by observing the horizontal extent of its graph. We find the domain of the inverse function by observing the vertical extent of the graph of the original function, because this corresponds to the horizontal extent of the inverse function. Similarly, we find the range of the inverse function by observing the horizontal extent of the graph of the original function, as this is the vertical extent of the inverse function. If we want to evaluate an inverse function, we find its input within its domain, which is all or part of the vertical axis of the original function’s graph.
How To: Given the graph of a function, evaluate its inverse at specific points.
- Find the desired input on the y-axis of the given graph.
- Read the inverse function’s output from the x-axis of the given graph.
Example 6: Evaluating a Function and Its Inverse from a Graph at Specific Points
A function is given in Figure 5. Find and . Figure 5
Figure 5Solution
To evaluate , we find 3 on the x-axis and find the corresponding output value on the y-axis. The point tells us that .
To evaluate , recall that by definition means the value of x for which . By looking for the output value 3 on the vertical axis, we find the point on the graph, which means , so by definition, .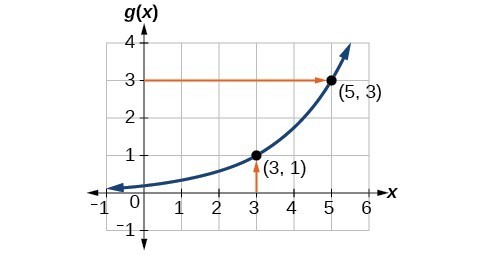 Figure 6
Figure 6Try It 6
Using the graph in Example 6, (a) find , and (b) estimate .
SolutionFinding Inverses of Functions Represented by Formulas
Sometimes we will need to know an inverse function for all elements of its domain, not just a few. If the original function is given as a formula— for example, as a function of we can often find the inverse function by solving to obtain as a function of .
How To: Given a function represented by a formula, find the inverse.
- Make sure is a one-to-one function.
- Solve for .
- Interchange and .
Example 7: Inverting the Fahrenheit-to-Celsius Function
Find a formula for the inverse function that gives Fahrenheit temperature as a function of Celsius temperature.
Solution
By solving in general, we have uncovered the inverse function. If
then
In this case, we introduced a function to represent the conversion because the input and output variables are descriptive, and writing could get confusing.
Try It 7
Solve for in terms of given
SolutionExample 8: Solving to Find an Inverse Function
Find the inverse of the function .
Solution
So or .
Example 9: Solving to Find an Inverse with Radicals
Find the inverse of the function .
Solution
So .
The domain of is . Notice that the range of is , so this means that the domain of the inverse function is also .
Analysis of the Solution
The formula we found for looks like it would be valid for all real . However, itself must have an inverse (namely, ) so we have to restrict the domain of to in order to make a one-to-one function. This domain of is exactly the range of .
Try It 8
What is the inverse of the function State the domains of both the function and the inverse function.
Solution
Analysis of the Solution
The domain and range of f exclude the values 3 and 4, respectively. f and f−1 are equal at two points but are not the same function, as we can see by creating the table below.