Section Exercises
1. Describe why the horizontal line test is an effective way to determine whether a function is one-to-one? 2. Why do we restrict the domain of the function to find the function’s inverse? 3. Can a function be its own inverse? Explain. 4. Are one-to-one functions either always increasing or always decreasing? Why or why not? 5. How do you find the inverse of a function algebraically? 6. Show that the function is its own inverse for all real numbers . For the following exercises, find for each function. 7. 8. 9. 10. 11. 12. For the following exercises, find a domain on which each function is one-to-one and non-decreasing. Write the domain in interval notation. Then find the inverse of restricted to that domain. 13. 14. 15. 16. Given and a. Find and b. What does the answer tell us about the relationship between and For the following exercises, use function composition to verify that and are inverse functions. 17. and 18. and For the following exercises, use a graphing utility to determine whether each function is one-to-one. 19. 20. 21. 22. For the following exercises, determine whether the graph represents a one-to-one function. 23. 24.
24.
 For the following exercises, use the graph of shown in [link].
For the following exercises, use the graph of shown in [link].
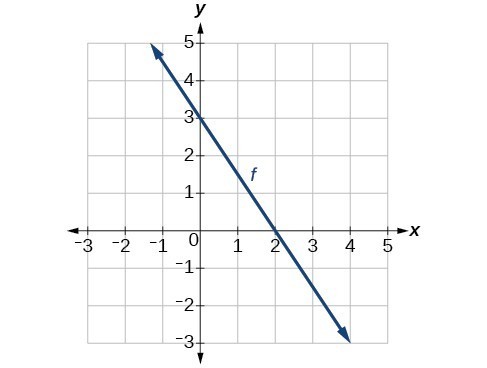 25. Find .
26. Solve .
27. Find .
28. Solve .
For the following exercises, use the graph of the one-to-one function shown below.
25. Find .
26. Solve .
27. Find .
28. Solve .
For the following exercises, use the graph of the one-to-one function shown below.
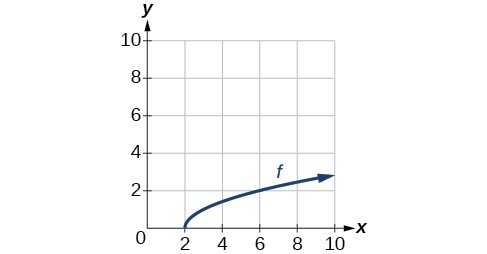 29. Sketch the graph of .
30. Find .
31. If the complete graph of is shown, find the domain of .
32. If the complete graph of is shown, find the range of .
For the following exercises, evaluate or solve, assuming that the function is one-to-one.
33. If , find .
34. If , find .
35. If , find .
36. If , find .
For the following exercises, use the values listed in the table below to evaluate or solve.
29. Sketch the graph of .
30. Find .
31. If the complete graph of is shown, find the domain of .
32. If the complete graph of is shown, find the range of .
For the following exercises, evaluate or solve, assuming that the function is one-to-one.
33. If , find .
34. If , find .
35. If , find .
36. If , find .
For the following exercises, use the values listed in the table below to evaluate or solve.
| 0 | 8 |
| 1 | 0 |
| 2 | 7 |
| 3 | 4 |
| 4 | 2 |
| 5 | 6 |
| 6 | 5 |
| 7 | 3 |
| 8 | 9 |
| 9 | 1 |
| 3 | 6 | 9 | 13 | 14 | |
| 1 | 4 | 7 | 12 | 16 |
