Solutions
Solutions to Try Its
1. Focus: ; Directrix: ; Endpoints of the latus rectum: 2. Focus: ; Directrix: ; Endpoints of the latus rectum: .
2. Focus: ; Directrix: ; Endpoints of the latus rectum: .
 3.
4.
5. Vertex: ; Axis of symmetry: ; Focus: ; Directrix: ; Endpoints of the latus rectum: and .
3.
4.
5. Vertex: ; Axis of symmetry: ; Focus: ; Directrix: ; Endpoints of the latus rectum: and .
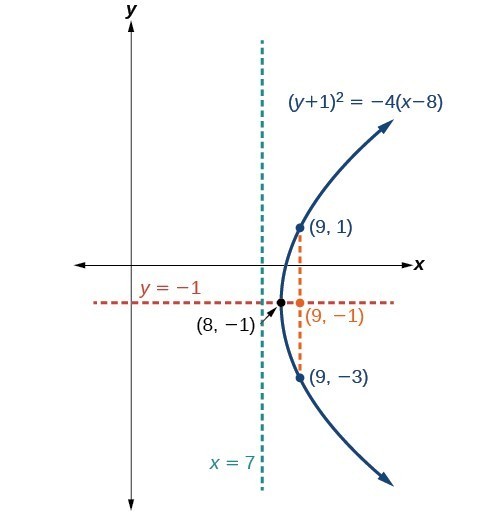 6. Vertex: ; Axis of symmetry: ; Focus: ; Directrix: ; Endpoints of the latus rectum: and .
6. Vertex: ; Axis of symmetry: ; Focus: ; Directrix: ; Endpoints of the latus rectum: and .
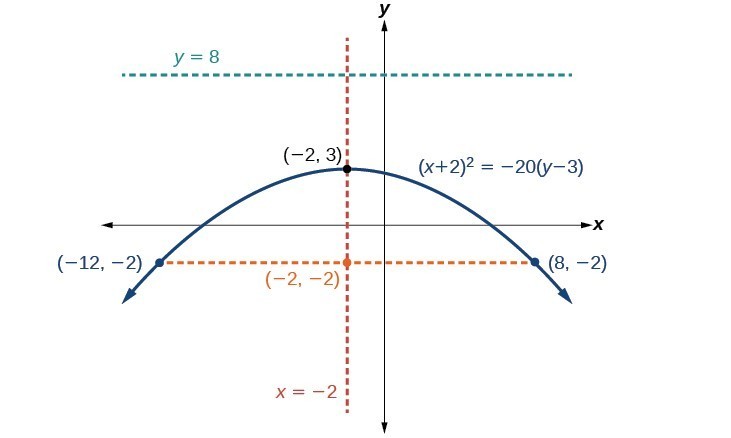 7. a.
b. The depth of the cooker is 500 mm
7. a.
b. The depth of the cooker is 500 mm
Solutions to Odd-Numbered Exercises
1. A parabola is the set of points in the plane that lie equidistant from a fixed point, the focus, and a fixed line, the directrix. 3. The graph will open down. 5. The distance between the focus and directrix will increase. 7. yes 9. yes 11. 13. 15. 17. 19. 21. 23. 25. 27. 29. 31.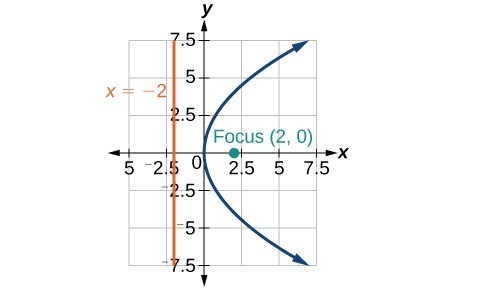 33.
33.
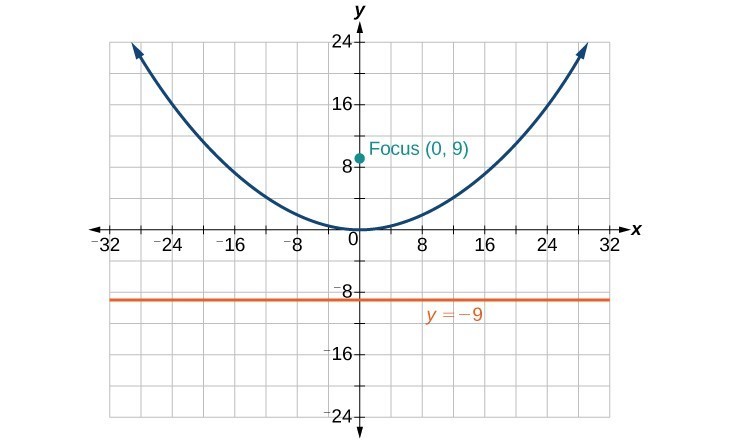 35.
35.
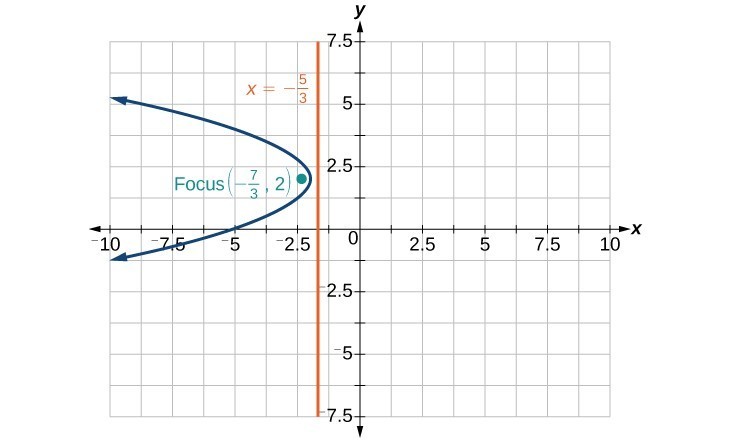 37.
37.
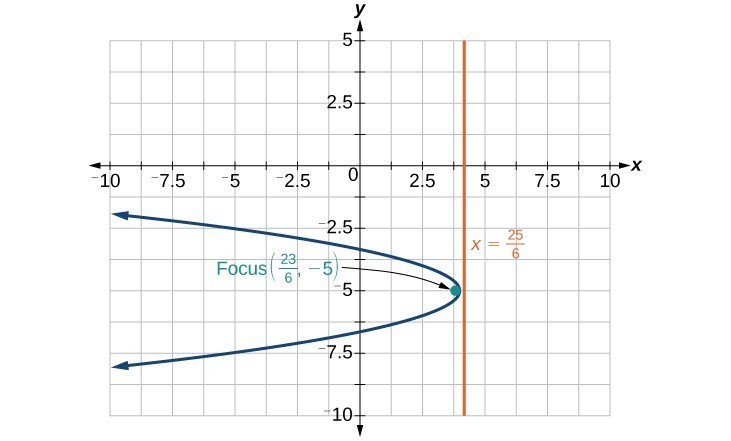 39.
39.
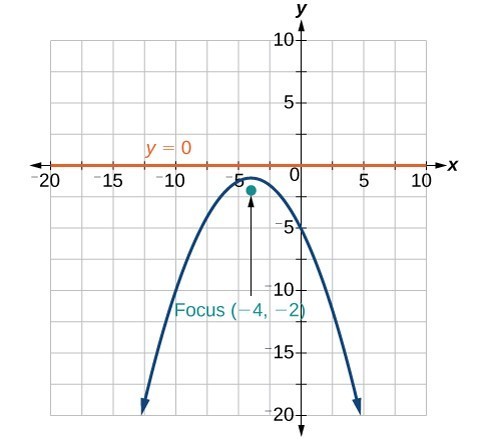 41.
41.
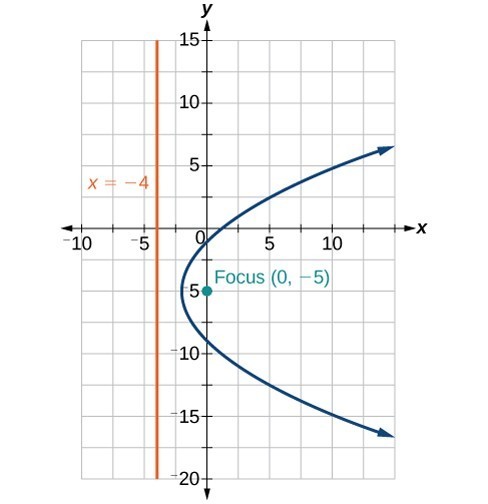 43.
43.
 45.
47.
49.
51.
53.
55.
57.
59.
61.
63. At the point 2.25 feet above the vertex.
65. 0.5625 feet
67. , height is 7.2 feet
69. 2304 feet
45.
47.
49.
51.
53.
55.
57.
59.
61.
63. At the point 2.25 feet above the vertex.
65. 0.5625 feet
67. , height is 7.2 feet
69. 2304 feet