Example
Graph
f(x)=−x+1.
Answer: Start with a table of values. You can choose different values for x, but once again, it’s helpful to include 0, some positive values, and some negative values.
If you think of f(x) as y, each row forms an ordered pair that you can plot on a coordinate grid.
f(−2)=−(−2)+1=2+1=3f(−1)=−(−1)+1=1+1=2f(0)=−(0)+1=0+1=1f(1)=−(1)+1=−1+1=0f(2)=−(2)+1=−2+1=−1
| x |
f(x) |
| −2 |
3 |
| −1 |
2 |
| 0 |
1 |
| 1 |
0 |
| 2 |
−1 |
Plot the points.
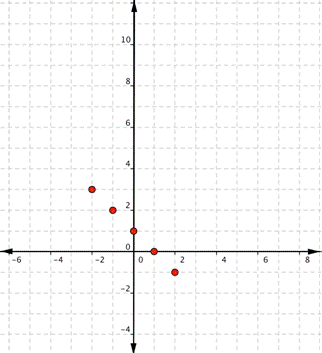
Answer
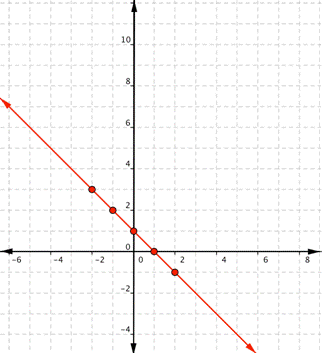
Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.
In the following video we show another example of how to graph a linear function on a set of coordinate axes.
https://youtu.be/sfzpdThXpA8
These graphs are representations of a linear function. Remember that a function is a correspondence between two variables, such as
A General Note: Linear Function
A
linear function is a function whose graph is a line. Linear functions can be written in the slope-intercept form of a line
f(x)=mx+b
where
b is the initial or starting value of the function (when input,
x=0), and
m is the constant rate of change, or
slope of the function. The
y-intercept is at
(0,b).
 Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.
Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.
 Let’s try another one. Before you look at the answer, try to make the table yourself and draw the graph on a piece of paper.
Let’s try another one. Before you look at the answer, try to make the table yourself and draw the graph on a piece of paper.

 Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.
Since the points lie on a line, use a straight edge to draw the line. Try to go through each point without moving the straight edge.
