Read: Solution Sets of Inequalities
Learning Objectives
- Identify whether an ordered pair is in the solution set of a linear inequality
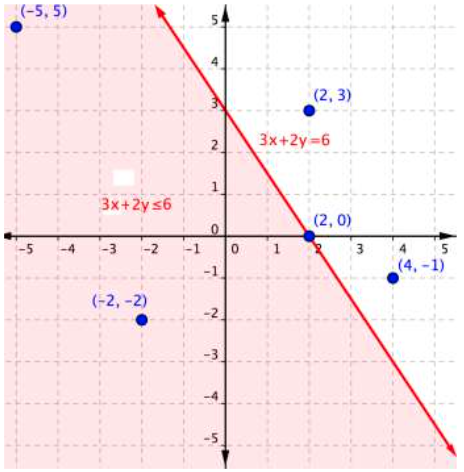 You can substitute the x- and y-values in each of the ordered pairs into the inequality to find solutions. Sometimes making a table of values makes sense for more complicated inequalities.
You can substitute the x- and y-values in each of the ordered pairs into the inequality to find solutions. Sometimes making a table of values makes sense for more complicated inequalities.
| Ordered Pair | Makes the inequality a true statement | Makes the inequality a false statement |
|---|---|---|
Example
Use the graph to determine which ordered pairs plotted below are solutions of the inequality .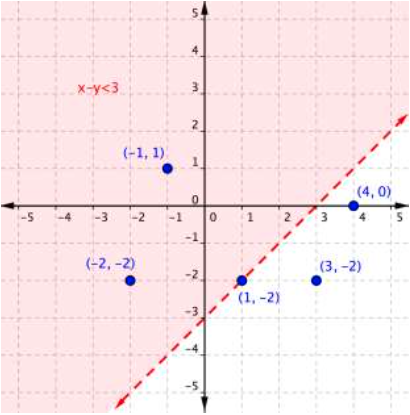
Answer: Solutions will be located in the shaded region. Since this is a “less than” problem, ordered pairs on the boundary line are not included in the solution set. These values are located in the shaded region, so are solutions. (When substituted into the inequality , they produce true statements.)
These values are not located in the shaded region, so are not solutions. (When substituted into the inequality , they produce false statements.)
Answer
Example
Is a solution of the inequality ?Answer: If is a solution, then it will yield a true statement when substituted into the inequality .
Substitute and into inequality.
Evaluate.
This statement is not true, so the ordered pair is not a solution.
