We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

TEXT
Unlock Solution Steps
Sign in to
Symbolab
Get full access to all Solution Steps for any math problem
By continuing, you agree to our Terms of Use
and have read our Privacy Policy

Study Guides > Intermediate Algebra

Read: Graph Piecewise Functions

−x−3[/latex], erasing the part where x is greater than [latex]-3[/latex]. Place an open circle at 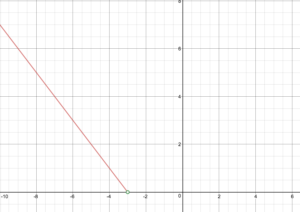[latex](-3,0)[/latex]. Graph of the line f(x)=-3-x with the restriction x<-3 Now place the line [latex]f(x) = x+3[/latex] on the graph, starting at the point [latex](-3,0)[/latex]. Note that for this portion of the graph, the point [latex](-3,0)[/latex] is included, so you can remove the open circle. 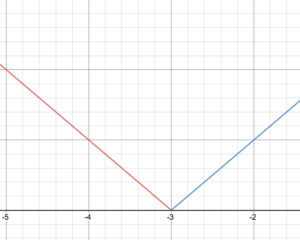graph of the line f(x)=-x-3 and f(x) = x+3 The two graphs meet at the point [latex](-3,0)[/latex] The domain of this function is all real numbers because [latex](-3,0)[/latex] is not included as the endpoint of [latex]f(x) = −x−3[/latex], but it is included as the endpoint for [latex]f(x) = x+3[/latex]. 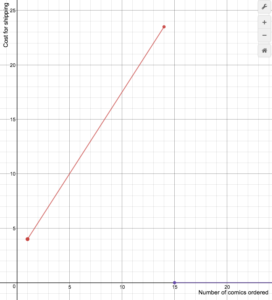The range of this function starts at [latex]f(x)=0[/latex] and includes [latex]0[/latex],  and goes to infinity, so we would write this as [latex]x\ge0[/latex][/hidden-answer] In the next example, we will graph a piecewise defined function that models the cost of shipping for an online comic book retailer.

Example

An on-line comic book retailer charges shipping costs according to the following formula [latex]S(n)=\begin{cases}1.5n+2.5\text{ if }1\le{n}\le14\\0\text{ if }n\ge15\end{cases}[/latex] Draw a graph of the cost function.

Answer: First, draw the line [latex]S(n)=1.5n+2.5[/latex].  We can use transformations: this is a vertical stretch of the identity by a factor of [latex]1.5[/latex], and a vertical shift by [latex]2.5[/latex].

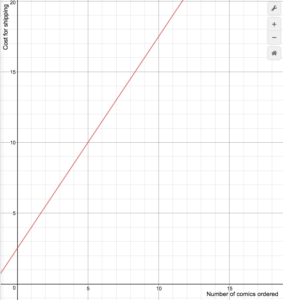S(n)=1.5n+2.5 S(n)=1.5n+2.5
Now we can eliminate the portions of the graph that are not in the domain based on [latex]1\le{n}\le14[/latex]
S(n) = 1.5n+2.5 for 1<=n<=14 S(n) = 1.5n+2.5 for 1=n=14
Last, add the constant function S(n)=[latex]0[/latex] for inputs greater than or equal to [latex]15[/latex]. Place closed dots on the ends of the graph to indicate the inclusion of the end points. Screen Shot 2016-07-07 at 1.09.58 PM

In the following video we show how to graph a piecewise defined function which is linear over both domains. https://youtu.be/B1jfpiI-QQ8

Summary

To graph piecewise functions, first identify where the domain is divided.  Graph functions on the domain using tools such as plotting points, or transformations. Be careful to use open or closed circles on the endpoints of each domain based on whether the endpoint is included.

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • College Algebra, Unit 1.4 Function Notation. Authored by: Carl Stitz and Jeff Zeager. Located at: https://www.stitz-zeager.com/szca07042013.pdf. License: CC BY: Attribution.
  • Ex 2: Graph a Piecewise Defined Function. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.

CC licensed content, Specific attribution

  • College Algebra. Provided by: OpenStax Authored by: Jay Abramson, et al.. Located at: https://openstax.org/books/precalculus/pages/1-introduction-to-functions. License: CC BY: Attribution. License terms: Download for free at: http://cnx.org/contents/[email protected].