We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Intermediate Algebra

Read: Proportions

Learning Objectives

  • Define and write a proportion
  • Solve proportional problems involving scale drawings
Matryoshka, or nesting dolls. Matryoshka, or nesting dolls.
A proportion is a statement that two ratios are equal to each other.  There are many things that can be represented with ratios, including the actual distance on the earth that is represented on a map.  In fact, you probably use proportional reasoning on a regular basis and don't realize it.  For example, say you have volunteered to provide drinks for a community event.  You are asked to bring enough drinks for [latex]35-40[/latex] people.  At the store  you see that drinks come in packages of [latex]12[/latex]. You multiply [latex]12[/latex] by [latex]3[/latex] and get [latex]36[/latex] - this may not be enough if [latex]40[/latex] people show up, so you decide to buy [latex]4[/latex] packages of drinks just to be sure. This process can also be expressed as a proportional equation and solved using mathematical principles. First, we can express the number of drinks in a package as a ratio:

[latex]\frac{12\text{ drinks }}{1\text{ package }}[/latex]

Then we express the number of people who we are buying drinks for as a ratio with the unknown number of packages we need. We will use the maximum so we have enough.

[latex]\frac{40\text{ people }}{x\text{ packages }}[/latex]

We can find out how many packages to purchase by setting the expressions equal to each other:

[latex]\frac{12\text{ drinks }}{1\text{ package }}=\frac{40\text{ people }}{x\text{ packages }}[/latex]

To solve for x, we can use techniques for solving linear equations, or we can cross multiply as a shortcut.

[latex]\begin{array}{l}\,\,\,\,\,\,\,\frac{12\text{ drinks }}{1\text{ package }}=\frac{40\text{ people }}{x\text{ packages }}\\\text{}\\x\cdot\frac{12\text{ drinks }}{1\text{ package }}=\frac{40\text{ people }}{x\text{ packages }}\cdot{x}\\\text{}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,12x=40\\\text{}\\\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,x=\frac{40}{12}=\frac{10}{3}=3.33\end{array}[/latex]

We can round up  to [latex]4[/latex] since it doesn't make sense to by [latex]0.33[/latex] of a package of drinks.  Of course, you don't write out your thinking this way when you are in the grocery store, but doing so helps you to be able to apply the concepts to less obvious problems.  In the following example we will show how to use a proportion to find the number of people on the planet who don't have access to a toilet. Because, why not?

Example

As of March, [latex]2016[/latex] the world's population was estimated at [latex]7.4[/latex] billion. [footnote] "Current World Population." World Population Clock: [latex]7.4[/latex] Billion People [latex](2016)[/latex]. Accessed June [latex]21, 2016[/latex]. http://www.worldometers.info/world-population/. "Current World Population." World Population Clock: [latex]7.4[/latex] Billion People [latex](2016)[/latex]. Accessed June [latex]21, 2016[/latex]. http://www.worldometers.info/world-population/. "Current World Population." World Population Clock: [latex]7.4[/latex] Billion People [latex](2016)[/latex]. Accessed June [latex]21, 2016[/latex]. http://www.worldometers.info/world-population/.[/footnote].  According to water.org, [latex]1[/latex] out of every [latex]3[/latex] people on the planet lives without access to a toilet.  Find the number of people on the planet that do not have access to a toilet.

Answer: We can use a proportion to find the unknown number of people who live without a toilet since we are given that [latex]1[/latex] in [latex]3[/latex] don't have access, and we are given the population of the planet. We know that [latex]1[/latex] out of every [latex]3[/latex] people don't have access, so we can write that as a ratio (fraction)

[latex]\frac{1}{3}[/latex].

Let the number of people without access to a toilet be x. The ratio of people with and without toilets is then

[latex]\frac{x}{7.4\text{ billion }}[/latex]

Equate the two ratios since they are representing the same fractional amount of the population.

[latex]\frac{1}{3}=\frac{x}{7.4\text{ billion }}[/latex]

Solve:

[latex]\begin{array}{l}\frac{1}{3}=\frac{x}{7.4}\\\text{}\\7.4\cdot\frac{1}{3}=\frac{x}{7.4}\cdot{7.4}\\\text{}\\2.46=x\end{array}[/latex]

The original units were billions of people, so our answer is [latex]2.46[/latex] billion people don't have access to a toilet.  Wow, that's a lot of people.

Answer

[latex]2.46[/latex] billion people don't have access to a toilet.

In the next example, we will use the length of a person't femur to estimate their height.  This process is used in forensic science and anthropology, and has been found in many scientific studies to be a very good estimate.

Example

It has been shown that a person's height is proportional to the length of their femur [footnote]Obialor, Ambrose, Churchill Ihentuge, and Frank Akapuaka. "Determination of Height Using Femur Length in Adult Population of Oguta Local Government Area of Imo State Nigeria." Federation of American Societies for Experimental Biology, April [latex]2015[/latex]. Accessed June [latex]22, 2016[/latex]. http://www.fasebj.org/content/29/1_Supplement/LB19.short.[/footnote]. Given that a person who is [latex]71[/latex] inches tall has a femur length of [latex]17.75[/latex] inches, how tall is someone with a femur length of [latex]16[/latex] inches?

Answer: Height and femur length are proportional for everyone, so we can define a ratio with the given height and femur length.  We can then use this to write a proportion to find the unknown height. Let x be the unknown height.  Define the ratio of femur length and height for both people using the given measurements.

Person [latex]1[/latex]:  [latex]\frac{\text{femur length}}{\text{height}}=\frac{17.75\text{inches}}{71\text{inches}}[/latex]

Person [latex]2[/latex]:  [latex]\frac{\text{femur length}}{\text{height}}=\frac{16\text{inches}}{x\text{inches}}[/latex]

Equate the ratios, since we are assuming height and femur length are proportional for everyone.

[latex]\frac{17.75\text{inches}}{71\text{inches}}=\frac{16\text{inches}}{x\text{inches}}[/latex]

 Solve by using the common denominator to clear fractions.  The common denominator is [latex]71x[/latex]

[latex]\begin{array}{c}\frac{17.75}{71}=\frac{16}{x}\\\\71x\cdot\frac{17.75}{71}=\frac{16}{x}\cdot{71x}\\\\17.75\cdot{x}=16\cdot{71}\\\\x=\frac{16\cdot{71}}{17.75}=64\end{array}[/latex]

The unknown height of person [latex]2[/latex] is [latex]64[/latex] inches. In general, we can reduce the fraction [latex]\frac{17.75}{71}=0.25=\frac{1}{4}[/latex] to find a general rule for everyone.  This would translate to sayinga person's height is 4 times the length of their femur.

Another way to describe the ratio of femur length to height that we found in the last example is to say there's a [latex]1:4[/latex] ratio between femur length and height, or [latex]1[/latex] to [latex]4[/latex]. Ratios are also used in scale drawings. Scale drawings are enlarged or reduced drawings of objects, buildings, roads, and maps. Maps are smaller than what they represent and a drawing of dendritic cells in your brain is most likely larger than what it represents. The scale of the drawing is a ratio that represents a comparison of the length of the actual object and it's representation in the drawing. The image below shows a map of the us with a scale of [latex]1[/latex] inch representing [latex]557[/latex] miles. We could write the scale factor as a fraction [latex]\frac{1}{557}[/latex] or as we did with the femur-height relationship, [latex]1:557[/latex].
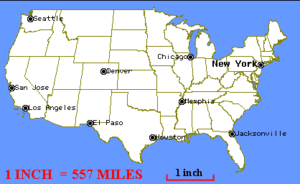
map of the lower 48 states with a scale factor of 1 inch equals 557 miles. Map with scale factor
In the next example we will use the scale factor given in the image above to find the distance between Seattle Washington and San Jose California.

Example

Given a scale factor of [latex]1:557[/latex] on a map of the US, if the distance from Seattle, WA to San Jose, CA is [latex]1.5[/latex] inches on the map,  define a proportion to find the actual distance between them.

Answer: We need to define a proportion to solve for the unknown distance between Seattle and San Jose.  The scale factor is [latex]1:557[/latex], and we will call the unknown distance x. The ratio of inches to miles is [latex]\frac{1}{557}[/latex]. We know inches between the two cities, but we don't know miles, so the ratio that describes the distance between them is [latex]\frac{1.5}{x}[/latex]. The proportion that will help us solve this problem is [latex]\frac{1}{557}=\frac{1.5}{x}[/latex]. Solve using the common denominator [latex]557x[/latex] to clear fractions. [latex-display]\begin{array}{ccc}\frac{1}{557}=\frac{1.5}{x}\\557x\cdot\frac{1}{557}=\frac{1.5}{x}\cdot{557x}\\x=1.5\cdot{557}=835.5\end{array}[/latex-display] We used the scale factor [latex]1:557[/latex] to find an unknown distance between Seattle and San Jose. We also checked our answer of [latex]835.5[/latex] miles with Google maps, and found that the distance is [latex]839.9[/latex] miles, so we did pretty well!

In the next example, we will find a scale factor given the length between two cities on a map, and their actual distance from each other.

Example

Two cities are [latex]2.5[/latex] inches apart on a map.  Their actual distance from each other is [latex]325[/latex] miles.  Write a proportion to represent and solve for the scale factor for one inch of the map.

Answer: We know that for each [latex]2.5[/latex] inches on the map, it represents [latex]325[/latex] actual miles. We are looking for the scale factor for one inch of the map. The ratio we want is [latex]\frac{1}{x}[/latex] where x is the actual distance represented by one inch on the map.  We know that for every [latex]2.5[/latex] inches, there are [latex]325[/latex] actual miles, so we can define that relationship as [latex]\frac{2.5}{325}[/latex] We can use a proportion to equate the two ratios and solve for the unknown distance.

[latex]\begin{array}{ccc}\frac{1}{x}=\frac{2.5}{325}\\325x\cdot\frac{1}{x}=\frac{2.5}{325}\cdot{325x}\\325=2.5x\\x=130\end{array}[/latex]

[latex]\begin{array}{ccc}\frac{1}{x}=\frac{2.5}{325}\\325x\cdot\frac{1}{x}=\frac{2.5}{325}\cdot{325x}\\325=2.5x\\x=130\end{array}[/latex]

The scale factor for one inch on the map is [latex]1:130[/latex], or for every inch of map there are [latex]130[/latex] actual miles.

In the video that follows, we present an example of using proportions to obtain the correct amount of medication for a patient, as well as finding a desired mixture of coffees. https://www.youtube.com/watch?v=yGid1a_x38g&feature=youtu.be

Licenses & Attributions

CC licensed content, Original

  • Screenshot: Matroyshka, or nesting dolls.. Provided by: Lumen Learning License: CC BY: Attribution.
  • Screenshot: map with scale factor. Provided by: Lumen Learning License: CC BY: Attribution.
  • Revision and Adaptation. Provided by: Lumen Learning License: CC BY: Attribution.

CC licensed content, Shared previously

  • Unit 15: Rational Expressions, from Developmental Math: An Open Program. Provided by: Monterey Institute of Technology and Education Located at: https://www.nroc.org/. License: CC BY: Attribution.
  • Ex: Proportion Applications - Mixtures . Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.