Read: Pythagorean Theorem
Learning Objectives
- Recognize a right triangle from other types of triangles
- Use the Pythagorean theorem to find the lengths of a right triangle
 Triangles
Triangles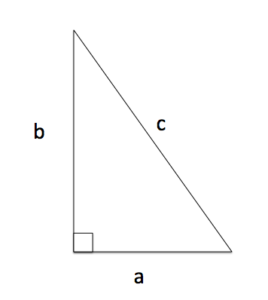 Right Triangle Labeled
Right Triangle LabeledThe Pythagorean theorem is often used to find unknown lengths of the sides of right triangles. If the longest leg of a right triangle is labeled c, and the other two a, and b as in the image on the left, The Pythagorean Theorem states that
Given enough information, we can solve for an unknown length. This relationship has been used for many, many years for things such as celestial navigation and early civil engineering projects. We now have digital GPS and survey equipment that have been programmed to do the calculations for us.
In the next example we will combine the power of the Pythagorean theorem and what we know about solving quadratic equations to find unknown lengths of right triangles.
Example
A right triangle has one leg with length x, another whose length is greater by two, and the length of the hypotenuse is greater by four. Find the lengths of the sides of the triangle. Use the image below.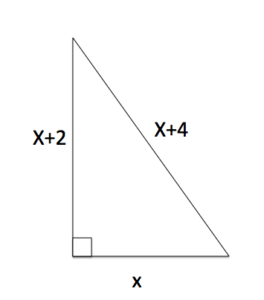
Answer: Read and understand: We know the lengths of all the sides of a triangle in terms of one side. We also know that the Pythagorean theorem will give us a relationship between the side lengths of a right triangle. Translate:
Solve: If we can move all the terms to one side and factor, we can use the zero product principle to solve. Since this is the only method we know - let's hope it works! First, multiply the binomials and simplify so we can see what we are working with.
Now move all the terms to one side and see if we can factor.
This went from a messy looking problem to something promising. We can factor using the shortcut:
So we can build our binomial factors with and :
Set each factor equal to zero:
Interpret: Ok, it doesn't make sense to have a length equal to , so we can safely throw that solution out. The lengths of the sides are as follows:
Check: Since we know the relationship between the sides of a right triangle we can check that we are correct. Sometimes it helps to draw a picture
.
We know that , so we can substitute the values we found:
Our solution checks out.
