Read: Solve Systems by Substitution
Learning Objectives
- Use substitution to solve a system algebraically
- Recognize when a system is inconsistent from algebraic results
- Find the break even point for a cost and revenue system
- Write a system of equations based on attendance and cost data
How To: Given a system of two equations in two variables, solve using the substitution method.
- Solve one of the two equations for one of the variables in terms of the other.
- Substitute the expression for this variable into the second equation, then solve for the remaining variable.
- Substitute that solution into either of the original equations to find the value of the first variable. If possible, write the solution as an ordered pair.
- Check the solution in both equations.
Example
Solve the following system of equations by substitution.Answer: First, we will solve the first equation for .
Now we can substitute the expression for in the second equation.
Now, we substitute into the first equation and solve for .
Our solution is .
Check the solution by substituting into both equations.
The substitution method can be used to solve any linear system in two variables, but the method works best if one of the equations contains a coefficient of 1 or so that we do not have to deal with fractions.
Example
Solve the following system of equations.
Answer: We can approach this problem in two ways. Because one equation is already solved for x, the most obvious step is to use substitution.
Clearly, this statement is a contradiction because . Therefore, the system has no solution. The second approach would be to first manipulate the equations so that they are both in slope-intercept form. We manipulate the first equation as follows.
We then convert the second equation expressed to slope-intercept form.
Comparing the equations, we see that they have the same slope but different y-intercepts. Therefore, the lines are parallel and do not intersect.
Writing the equations in slope-intercept form confirms that the system is inconsistent because all lines will intersect eventually unless they are parallel. Parallel lines will never intersect; thus, the two lines have no points in common. The graphs of the equations in this example are shown below.
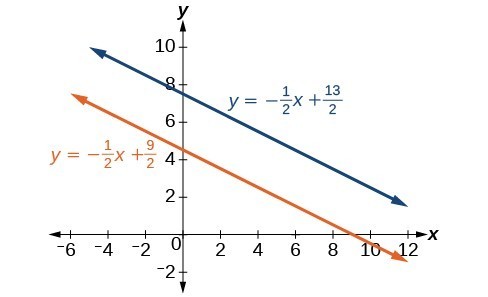
Answer
There is no solution to this system of linear equations.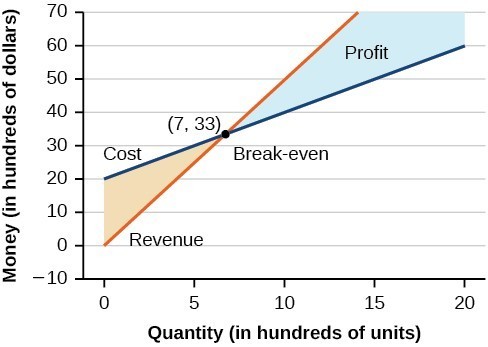 The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
The point at which the two lines intersect is called the break-even point. We can see from the graph that if 700 units are produced, the cost is $3,300 and the revenue is also $3,300. In other words, the company breaks even if they produce and sell 700 units. They neither make money nor lose money.
The shaded region to the right of the break-even point represents quantities for which the company makes a profit. The shaded region to the left represents quantities for which the company suffers a loss.
Example
Given the cost function and the revenue function , find the break-even point.Answer: Write the system of equations using to replace function notation.
Substitute the expression from the first equation into the second equation and solve for .
Then, we substitute into either the cost function or the revenue function.
The break-even point is . The cost to produce units is $77,500, and the revenue from the sales of units is also $77,500. To make a profit, the business must produce and sell more than units.
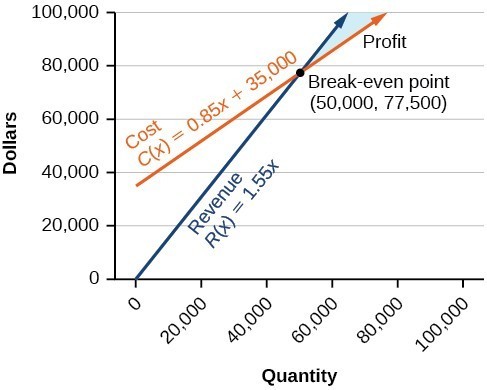 The company will make a profit after units are produced.
The company will make a profit after units are produced.
Example
The cost of a ticket to the circus is $25.00 for children and $50.00 for adults. On a certain day, attendance at the circus is and the total gate revenue is $70,000. How many children and how many adults bought tickets?Answer: Let the number of children and the number of adults in attendance. The total number of people is . We can use this to write an equation for the number of people at the circus that day
The revenue from all children can be found by multiplying $25.00 by the number of children, . The revenue from all adults can be found by multiplying $50.00 by the number of adults, . The total revenue is $70,000. We can use this to write an equation for the revenue.
We now have a system of linear equations in two variables.
In the first equation, the coefficient of both variables is . We can quickly solve the first equation for either or . We will solve for .
Substitute the expression in the second equation for and solve for .
Substitute into the first equation to solve for .
We find that children and adults bought tickets to the circus that day.
