We've updated our
Privacy Policy effective December 15. Please read our updated Privacy Policy and tap

Study Guides > Mathematics for the Liberal Arts

Another Method for Converting Between Bases

Learning Outcomes

  • Become familiar with the history of positional number systems
  • Identify bases that have been used in number systems historically
  • Convert numbers between bases
  • Use two different methods for converting numbers between bases

Another Method For Converting From Base 10 to Other Bases

As you read the solution to this last example and attempted the “Try It” problems, you may have had to repeatedly stop and think about what was going on. The fact that you are probably struggling to follow the explanation and reproduce the process yourself is mostly due to the fact that the non-decimal systems are so unfamiliar to you. In fact, the only system that you are probably comfortable with is the decimal system. As budding mathematicians, you should always be asking questions like “How could I simplify this process?” In general, that is one of the main things that mathematicians do: they look for ways to take complicated situations and make them easier or more familiar. In this section we will attempt to do that. To do so, we will start by looking at our own decimal system. What we do may seem obvious and maybe even intuitive but that’s the point. We want to find a process that we readily recognize works and makes sense to us in a familiar system and then use it to extend our results to a different, unfamiliar system. Let’s start with the decimal number, 486310. We will convert this number to base 10. Yeah, I know it’s already in base 10, but if you carefully follow what we’re doing, you’ll see it makes things work out very nicely with other bases later on. We first note that the highest power of 10 that will divide into 4863 at least once is 103 = 1000. In general, this is the first step in our new process; we find the highest power that a given base that will divide at least once into our given number.

We now divide 1000 into 4863:

4863 ÷ 1000 = 4.863

This says that there are four thousands in 4863 (obviously). However, it also says that there are 0.863 thousands in 4863. This fractional part is our remainder and will be converted to lower powers of our base (10). If we take that decimal and multiply by 10 (since that’s the base we’re in) we get the following:

0.863 × 10 = 8.63

Why multiply by 10 at this point? We need to recognize here that 0.863 thousands is the same as 8.63 hundreds. Think about that until it sinks in.

(0.863)(1000) = 863 (8.63)(100) = 863

These two statements are equivalent. So, what we are really doing here by multiplying by 10 is rephrasing or converting from one place (thousands) to the next place down (hundreds).

0.863 × 10 ⇒ 8.63 (Parts of Thousands) × 10 ⇒ Hundreds

What we have now is 8 hundreds and a remainder of 0.63 hundreds, which is the same as 6.3 tens. We can do this again with the 0.63 that remains after this first step.

0.63 × 10 ⇒ 6.3 Hundreds × 10 ⇒ Tens

So we have six tens and 0.3 tens, which is the same as 3 ones, our last place value. Now here’s the punch line. Let’s put all of the together in one place: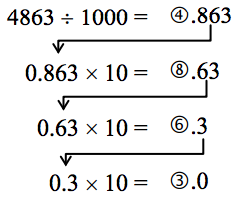 4863 divided by 1000 = 4.863, 0.863x 10 = 8.63, 0.63x10 = 6.3, 0.3x10 = 3.0 Converting from Base 10 to Base b: Another methodNote that in each step, the remainder is carried down to the next step and multiplied by 10, the base. Also, at each step, the whole number part, which is circled, gives the digit that belongs in that particular place. What is amazing is that this works for any base! So, to convert from a base 10 number to some other base, b, we have the following steps we can follow:

Converting from Base 10 to Base b: Another method

  1. Find the highest power of the base b that will divide into the given number at least once and then divide.
  2. Keep the whole number part, and multiply the fractional part by the base b.
  3. Repeat step two, keeping the whole number part (including 0), carrying the fractional part to the next step until only a whole number result is obtained.
  4. Collect all your whole number parts to get your number in base b notation.
We will illustrate this procedure with some examples.

Example

Convert the base 10 number, 34810, to base 5.

Answer: This is actually a conversion that we have done in a previous example. The powers of five are: 50 = 1 51 = 5 52 = 25 53 = 125 54 = 625 Etc… The highest power of five that will go into 348 at least once is 53. We divide by 125 and then proceed. Fig5_1_21 By keeping all the whole number parts, from top bottom, gives 2343 as our base 5 number. Thus, 23435 = 34810.  

We can compare our result with what we saw earlier, or simply check with our calculator, and find that these two numbers really are equivalent to each other.

Example

Convert the base 10 number, 300710, to base 5.

Answer: The highest power of 5 that divides at least once into 3007 is 54 = 625. Thus, we have: 3007 ÷ 625 = ④.8112 0.8112 × 5 = ④.056 0.056 × 5 = ⓪.28 0.28 × 5 = ①0.4 0.4 × 5 = ②0.0 This gives us that 300710 = 440125. Notice that in the third line that multiplying by 5 gave us 0 for our whole number part. We don’t discard that! The zero tells us that a zero in that place. That is, there are no 52s in this number.

This last example shows the importance of using a calculator in certain situations and taking care to avoid clearing the calculator’s memory or display until you get to the very end of the process.

Example

Convert the base 10 number, 6320110, to base 7.

Answer: The powers of 7 are: 70 = 1 71 = 7 72 = 49 73 = 343 74 = 2401 75 = 16807 etc… The highest power of 7 that will divide at least once into 63201 is 75. When we do the initial division on a calculator, we get the following: 63201 ÷ 75 = 3.760397453 The decimal part actually fills up the calculators display and we don’t know if it terminates at some point or perhaps even repeats down the road. So if we clear our calculator at this point, we will introduce error that is likely to keep this process from ever ending. To avoid this problem, we leave the result in the calculator and simply subtract 3 from this to get the fractional part all by itself. Do not round off! Subtraction and then multiplication by seven gives: 63201 ÷ 75 = ƒ③.760397453 0.760397453 × 7 = …⑤.322782174 0.322782174 × 7 =‚ ②.259475219 0.259475219 × 7 = ①.816326531 0.816326531 × 7 = ⑤….714285714 0.714285714 × 7 = …⑤.000000000 Yes, believe it or not, that last product is exactly 5, as long as you don’t clear anything out on your calculator. This gives us our final result: 6320110 = 3521557.

If we round, even to two decimal places in each step, clearing our calculator out at each step along the way, we will get a series of numbers that do not terminate, but begin repeating themselves endlessly. (Try it!) We end up with something that doesn’t make any sense, at least not in this context. So be careful to use your calculator cautiously on these conversion problems. Also, remember that if your first division is by 75, then you expect to have 6 digits in the final answer, corresponding to the places for 75, 74, and so on down to 70. If you find yourself with more than 6 digits due to rounding errors, you know something went wrong.

Try It

Convert the base 10 number, 935210, to base 5.

Answer: [latex]9352_{10} = 244402_{5}[/latex]

Convert the base 10 number, 1500, to base 3. Be careful not to clear your calculator on this one. Also, if you’re not careful in each step, you may not get all of the digits you’re looking for, so move slowly and with caution.

Answer: [latex-display]1500_{10} = 2001120_{3}[/latex-display]

The following video shows how to use a calculator to convert numbers in base 10 into other bases. https://youtu.be/YNPTYelCeIs

Licenses & Attributions

CC licensed content, Original

CC licensed content, Shared previously

  • Math in Society. Authored by: Lippman, David. Located at: http://www.opentextbookstore.com/mathinsociety/. License: CC BY: Attribution.
  • Question ID 8681. Authored by: Lippman, David. License: CC BY: Attribution. License terms: IMathAS Community License CC-BY + GPL.
  • Convert Numbers in Base Ten to Different Bases: Calculator Method. Authored by: James Sousa (Mathispower4u.com) . License: CC BY: Attribution.